Complete Solutions to Question Bank of chapter LIMITS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | LIMITS |
| CENGAGE | Question Bank |
| Number Of Questions | 17 |
| Academic Year | 2023-24 |
If `Lim_(x rarr 0) (1-cosx)/(e^(ax)-bx-1)` exist and is equal to 1, then `(a^(2) + b^(2))` equals
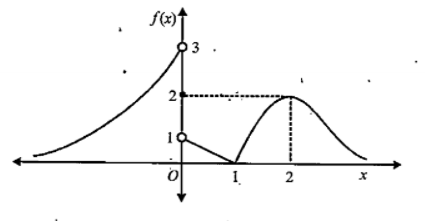
04:58View SolutionIf graph of a function f(x) is shown in the adjacent figure, then `Lim_(x rarr 0^(-)) [(4f(x)-6[f(x)])/(tan(2f(x)-6))]` is equal to
 03:11View Solution
03:11View SolutionLet f(x) =`(sec x)^(cosecx) + (cot x)^(sin x)`. Then the value of `Lim_(x rarr 0^(+)) f(x)` is equal to
07:44View Solution`Lim_(z rarr 0){{max((sin^(-1)x + cos^(-1)x)^(2), min(x^(2)+4x+7))}. (sin^(-1)z)/z]` is equal to (where [.] denotes greatest integer function)
02:42View SolutionThe reciprocal of the value of: `("lim")_(ntooo)(1-1/(2^2))(1-1/(3^2))(1-1/(4^2))(1-1/(n^2))i s `
03:23View SolutionThe value of `Lim_(x rarr 0)(sum_(k =1)^(2016){(sinx)/x+2015k})/2016` is [where {x} represents fractional part of x]
02:34View Solution`Lim_(x rarr oo) (30+4sqrtx+7root(3)(x))/(2+sqrt(4x-7)+root(3)(6x-2) )`equals
03:17View SolutionIf `Lim_(x rarr0) ((a sin x + b tan x)/x^(3))= 3/2` then |a+2b| is equal to
03:39View Solution`lim_(n rarr 0)(1/(1+n^(2))+2/(1+n^(2))+3/(1+n^(2))+....+n/(1+n^(2)))` is equal to
01:41View SolutionFind the value of `lim_(n rarr oo)sum_(k=1)^(n)(k^(2)+k-1)/((k+1)!) `.
03:35View SolutionThe value of `Lim_(x rarr 0)((tanx)^(1/x)+(1+sinx)^(x))` where `x gt0` is equal to
02:42View SolutionIf `Lim_(x rarr 0) (a sinx + bxe^(x)+3x^(2))/(sin x-2x+tanx) exists and has value equal to L, then the value of (a+L)/b is equal to
02:45View Solution`If Lim_(x rarr a) (sqrt(x-b)-sqrt(a-b))/(x^(2)-a^(2))(a gt b) = 1/64` and ` Lim_(x rarr oo) (sqrt(x^(2)+ax)-sqrt(x^(2)+bx)) = 2` then the value of a/b, is`
06:10View SolutionIf `lim_(x rarr 0) (k+cos lx)/x^(2)`exists and has the value equal to -4 then find `l^(2)`
02:00View Solution`Lim_(x rarr 0)((3sin x- sin 3x)^(4))/((sec x - cosx)^(6))` is equal to
02:40View Solution`Lim_(x rarr 0)(tanx sqrt (tanx)-sin x sqrt(sinx))/(x^(3).sqrtx)` equals
07:02View Solution`f(x)=("ln"(x^(2)+e^(x)))/("ln"(x^(4)+e^(2x)))." Then "underset(xtooo)limf(x)" is equal to "`
04:58View Solution
Question Bank questions and complete solutions for chapter LIMITS of CENGAGE of Class 12
All Exercises from CENGAGE Class 12 MATHS LIMITS
- Comprehension Type
- ComprehensionType
- Examples
- Exercise (Comprehension)
- Exercise (Matrix)
- Exercise (Multiple)
- Exercise (Numerical)
- Exercise (Single)
- Exercise 2.1
- Exercise 2.2
- Exercise 2.3
- Exercise 2.4
- Exercise 2.5
- Exercise 2.6
- Exercise 2.7
- Exercise 2.8
- JEE Advanced Previous Year
- JEE Main Previous Year
- Multiple Correct Answer Type
- Multiple Correct Answers Type
- Question Bank
- Single Correct Answer Type
