Complete Solutions to Exercise 2.2 of chapter WAVE OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| CENGAGE PHYSICS | Exercise 2.2 |
| Number Of Questions | 22 |
| Academic Year | 2023-24 |
In Young's double slit experiment with wavelength 5890 `Å`, there are 60 fringe in the field of vision, How many fringes will be observed in the same field of vision id wavelength used is 5460 `Å`?
View SolutionIn YDSE performed with wavelength `lambda = 5890 Å` the angular fringe width is `0.40^(@)`. What is the angular fringe width if the entire set-up is immersed in water?
View SolutionIn YDSE for wavelength `lambda = 589nm`, the interference fringe have angular separation of `3050 xx 10^(-3)` rad. For what wavelength would the angular separation be 10.0% greater?
View SolutionA beam of light consisting of wavelengths 6000 `Å` and 4500 `Å` is used in a YDSE with D = 1 m and d = 1 mm. Find the least distance from the central maxima, where bright fringes due to the two wavelengths coincide.
View SolutionWhite light is used in a YDSE with `D = 1 m` and `d = 0.9 mm`. Light reaching the screen at position `y = 1 mm` us passed through a prism and its spectrum is obtained. Find the missing lines in the visible region of this spectrum.
View SolutionMonichromatic lightof wavelenght 600 nm is used ina Young's double slilt experient. One of the slits is covered by a transparent sheet of thicknes `1.8xx10^-5` m made of a material of refractive index 1.6. How many fringe will shift due to the introduction of the sheet?
View SolutionInterference fringes are produced by a double slit arrangement
and a piece of plane parallel glass of refractive index 1.5 is interposed in one of
the interfering beam. If the fringes are displaced through 30 fringe widths for
light of wavelength `6xx 10^(-5) cm`, find the thickness of the plate.
View SolutionIn YDSE with `d = 1 mm` and `D = 1 m`, Slabs of `(t = 1 mu m, mu = 3)` and `(t = 0.5mu m, mu = 2)` are introduced in front of upper and lower slits, respectively. Find the shift in the fringe pattern.
View SolutionIn YDSE with `D = 1 m, d = 1 mm`, light of wavelength `500 nm` is incident at an angle of `0.57^(@)` w.r.t. the axis of symmetry of the experimental set-up. If center of symmetry of screen is O as shown figure.
a. find the position of centrla maxima,
b. find intensity at point O in terms of intensity of central maxima `I_(0)`, and
c. find number of maixma lying central maxima.
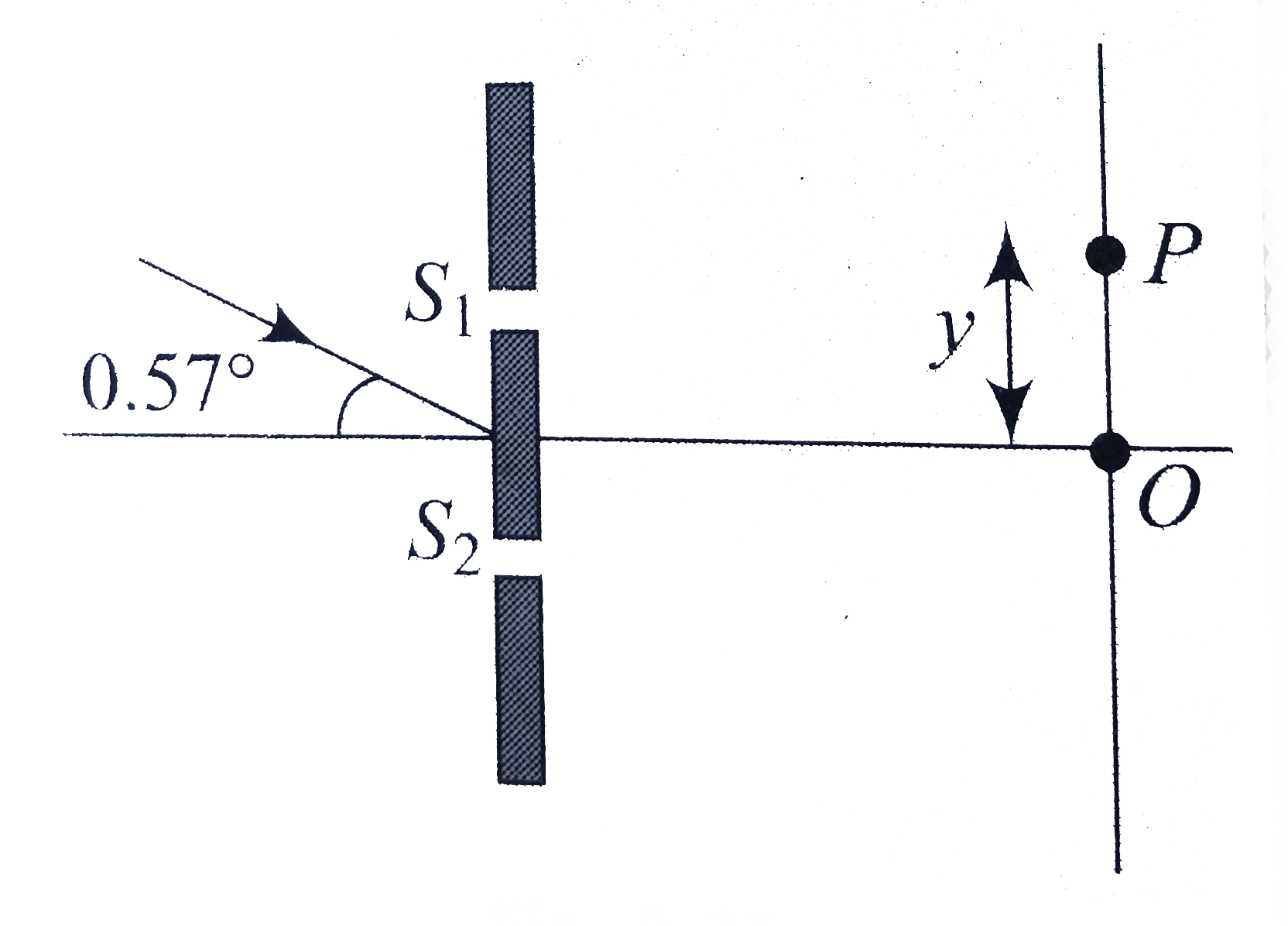 .
.
View SolutionIn Young's experiment, find the distance between two slits that results in the third minimum for `420 nm` violet light at an angle `30^(@)`
 .
.
View SolutionConsider an interference arrangement similar to YDSE. Slits `S_(1)` and `S_(2)` are illuminated with coherent microwave sources each of frequency 2 MHz The sources are synchronized to have zero phase difference. The slits are separated by distance d= 75m. The intensity `I_((theta))` is measured as a function of `theta` where `theta` is defined as shown in figure. if `I_(0)` is maximum intensity then calculated `I_((theta))` for `(i) theta= 0^(@), (ii) theta=pi//6 and (iii) theta = pi//2`
View SolutionIf Young's double-slit experiment is performed under water, how would be observed interference pattern be affected ?
View SolutionIn Young's double -slit experiment, why do we use mono chromatic light ? If while light is used, how would the pattern change ?
View SolutionYoung's interference experiment is performed with monochromatic light. The separation between the slits is 0.500 mm, and the interference pattern on a screen 3.30 m away shows the first side maximum 3.40 mm form the center of the pattern. What is the wavelength ?
View SolutionTwo radio antennas separated by 300 m as shown in figure simultaneously broadcast indentical singals at the same wavelength. A radio in a car traveling due north receives the signals. (a) If the car is at the position of the second maximum, what is the wavelength of the signals? (b) How much farther must the car travel to encounter the next mininum in reception? (Note: Do not use the small- angle approximation in this problem.)
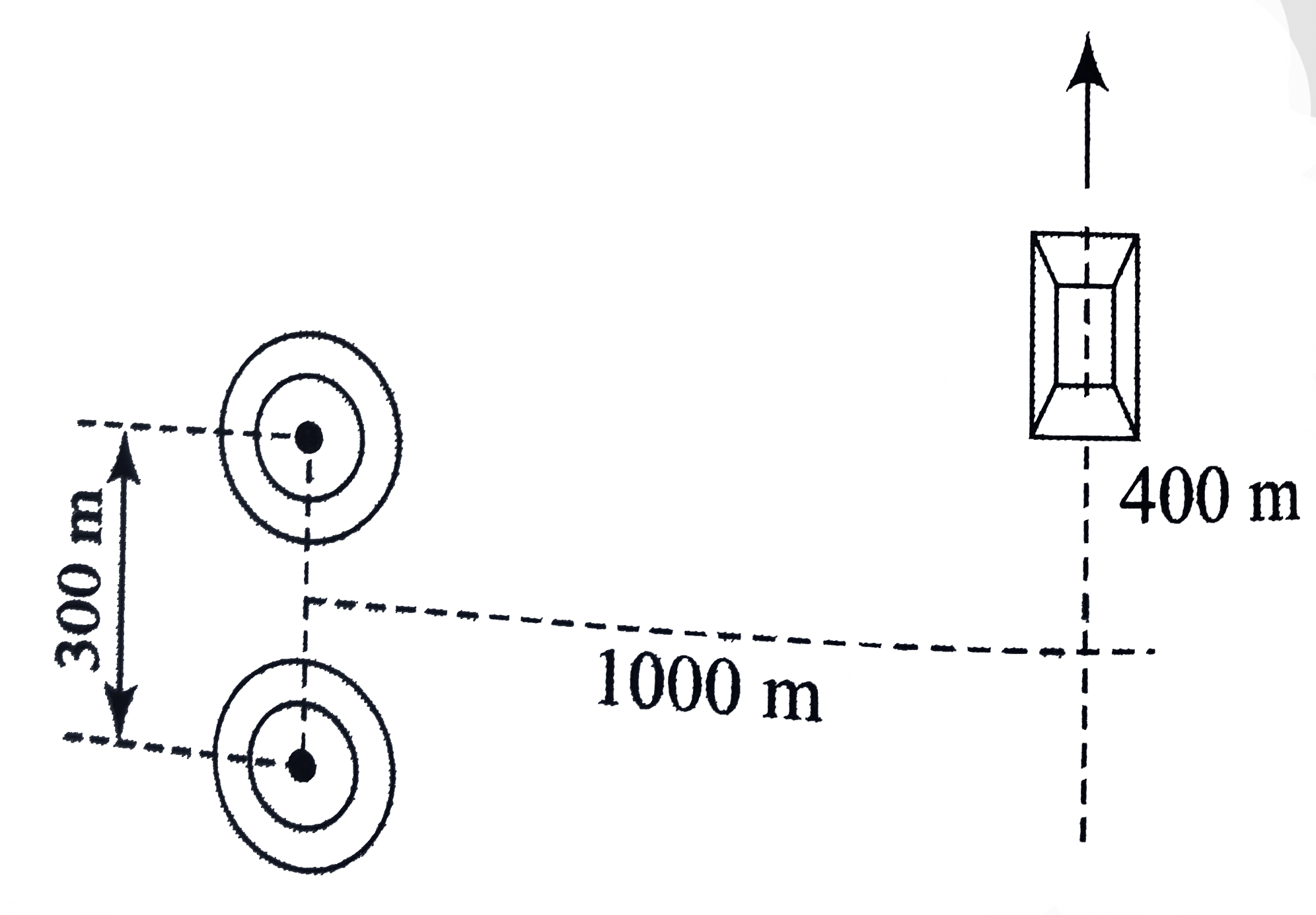
View SolutionYoung's double-slit experiment is performed with a 600 nm light and a distance of 2.00 m between the slits and the screen. The tenth interference minimum is observed 7.5mm, from the central maximum . Determine the spacing of the slits.
View SolutionTwo slits are separated by 0.320 mm. A beam of 500 nm light strikes the slits, producing an interference pattern. Determine the number of maxima observed in the angular range `- 30.0^(@) lt theta lt 30.0^(@)`.
View SolutionThe intensity on the screen at a certain point in a double-slit interference patteren is 25.0% of the maximum value. (a) What minimum phase difference (in radians) between the sources produces this result? (b) Express this phase difference as a path difference for 486.1 nm light.
View SolutionDistance between the slits, in YDSE, shown in figure is `d=20lamda` where `lambda` is the wavelength of light used. Find the `angle theta` where

(i) central maxima (where path difference is zero) is obtained.
(ii) third order maxima is obtained.
View SolutionIn Young's double slit experiment, first slit has width four times the width of the second slit. The ratio of the maximum intensity to the minimum intensity in the interference fringe system is
View SolutionThe width of one of the two slits in a Young's double slit experiment is double of the other slit. Assuming that the amplitude of the light coming from a slit is proportional to the slit width, find the ratio of the maximum to the minimum intensity in the interference pattern.
View SolutionIn Young's double slit experiment, one of the slit is wider than other, so that the amplitude of the light from one slit is double of that from other slit. If `I_(m)` be the maximum intensity, the resultant intensity I when they interfere at phase difference `phi` is given by
View Solution
Exercise 2.2 questions and complete solutions for chapter WAVE OPTICS of CENGAGE PHYSICS (ENGLISH) of Class 12