Complete Solutions to Single Correct of chapter ATOMIC PHYSICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ATOMIC PHYSICS |
| CENGAGE PHYSICS | Single Correct |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
If the wavelength of the first line of the Balmer series of hydrogen is `6561 Å`, the wavelngth of the second line of the series should be
View SolutionThree photons coming from excited atoms hydrogen sample are picked up .There energies are `12.1eV, 10.2eV and 1.9eV` these photons must come from
View SolutionWhenever a hydrogen atom emits a photon in the Balmer series .
View Solutiontwo electron are revolving around a nucleus at distance `t and `4 r`. The ratio of their periods is
View SolutionA hydrogen atom in ground state absorbs `10.2eV` of energy .The orbital angular momentum of the electron is increases by
View SolutionIn terms of Rydberg constant `R`, the shortest wavelength in the Balmer series of the hydrogen , atom spestrum will have wavelength
View SolutionThe ratio of minimum to maximum wavelength in Balmer series is
View SolutionThe frequency of revolution of an electron in nth orbit is `f_(n)`. If the electron makes a transition from nth orbit to `(n = 1)` th orbit , then the relation between the frequency `(v)` of emitted photon and `f_(n)` will be
View SolutionIn a hydrogen like atom electron make transition from an energy level with quantum number `n` to another with quantum number `(n - 1)` if `n gtgt1` , the frequency of radiation emitted is proportional to :
View SolutionFigure shown five energy levels of an atom , one being much lower than the other four. Five transitios between the levels are idicated, each of which produces a photon of definite energy and frequency.
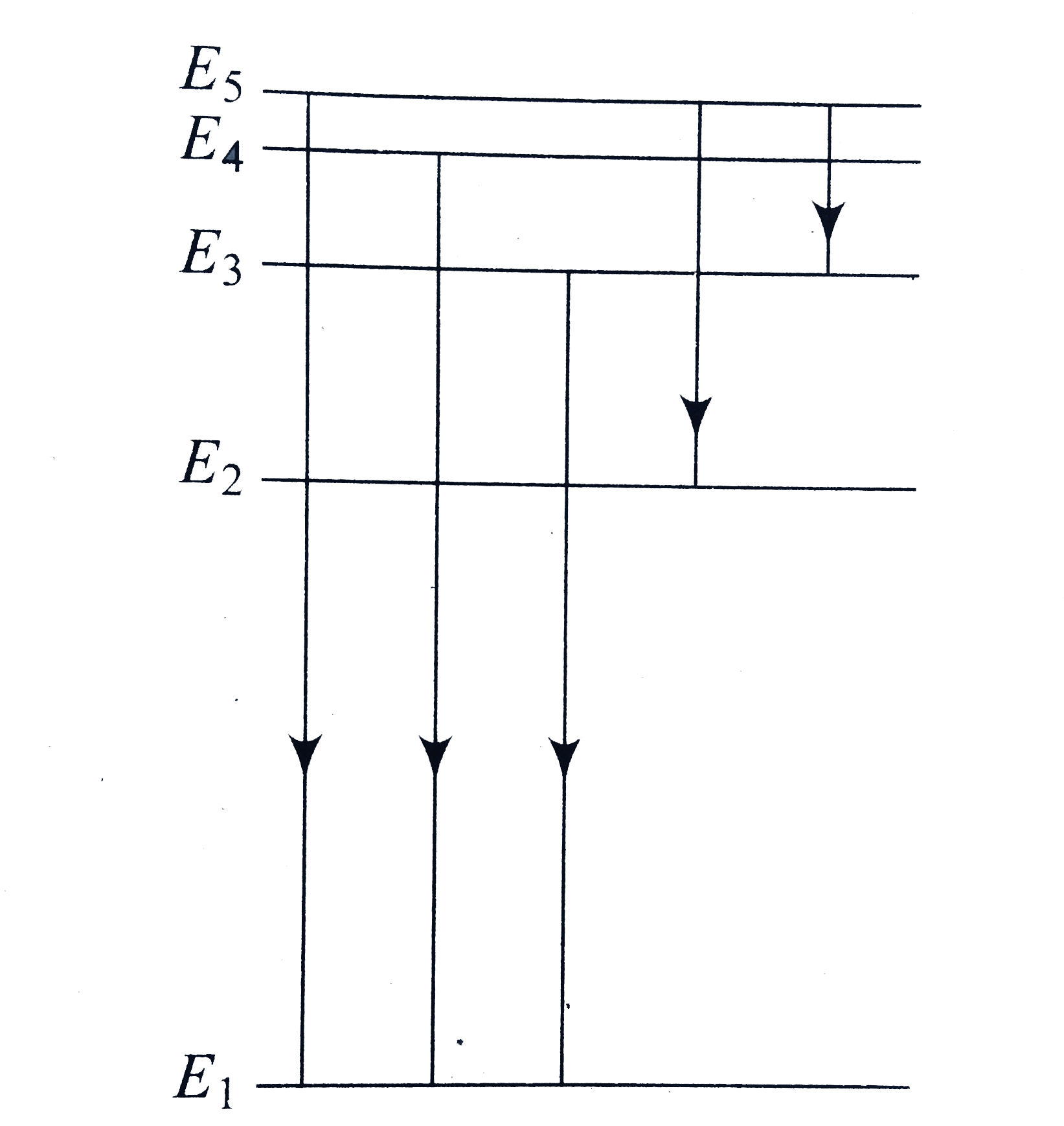 Which one of the spectrum below best corresponding to the set of transition indicated?
Which one of the spectrum below best corresponding to the set of transition indicated?
View SolutionFigure represent some of the lower energy level of the hydrogen atom in simplified from.
If the transition of an electron from `E_(4) to E_(2)` were accociated with the emission of blue light , which one of the following transition could be accociated with the emission of red light?

View SolutionThe orbiting `v_(n) of e^(bar)` in the nth orbit in the case of positronium is x-field compared to that in the nth orbit in a hydrogen atom , where `x` has the value
View SolutionFigure shown the electron energy level , referred to the ground state (the lowest possible energy) as zero , for five different isoleted atoms. Which atom can produce radiation of the shortest wavelength when atoms in the ground state are bombarded with electrons energy `W`?

View SolutionWhen an electron jumps from a level `n = 4` to` n = 1`, the momentum of the recoiled hydrogen atom will be
View SolutionCheck the corretness of the following statement about the Bohr model of hydrogen atom:
(i) The acceleration of the electron in `n = 2` orbit is more than that in `n = 1` orbit.
(ii) The angular momentum of the electron in `n = 2` orbit is more than that in `n = 1` orbit.
(iii) The `KE` of the electron in `n = 2` orbit is more than that in `n = 1` orbit.
View Solutionwhen a hydrogen atom is raised from the ground state to an excited state
View SolutionAs the electron in the Bohr orbit is hydrogen atom passes from state `n = 2` to `n = 1` , the `KE (K) and PE (U)` charge as
View SolutionConsider a spectral line resulting `n = 5` to `n = 1`, in the atom and loss given below. The shortest wavelength is produced by
View SolutionWhich of the following statement is true regarding Bohr's model of hydrogen atom ?
(I) Orbiting speed of electrons decreases as if falls to discrete orbits away from the nuclues.
(II) Radii of allowed orbits of electrons are proportional to the principle quantum number.
(III) Frequency with which electrons orbit around the nucleus in discrete orbits is inversely proportional to the principle quantum number.
(IV) Binding froce with which the elctron is bound to the nuclues increases as it shifts to outer orbits.
Selected the correct answer using the codes given below:
View SolutionIn the Bohr model of a hydrogen atom, the centripetal force is furnished by the Coulomb attraction between the proton and the electrons. If `a_(0)` is the radius of the ground state orbit, `m` is the mass and `e` is the charge on the electron and `e_(0)` is the vacuum permittivity, the speed of the electron is
View SolutionHydrogen atoms are excited from ground state of the principle quantum number `4`. Then the number of spectral lines observed will be
View SolutionWhen an electron jumps from `n_(1) th orbit to `n_(2)` th orbit, the energy radiated is given by
View SolutionIn Bohr's model of hydrogen atom, let `PE` represents potential energy and `TE` the total energy. In going to a higher level
View Solutionif the electron in hydrogen orbit jumps form third orbit to second orbit, the wavelength of the emitted radiation is given by
View SolutionThe energy change is greatest for a hydrogen atom when its state changes from
View SolutionThe electron in a hydrogen atom jumps from ground state to the higher energy state where its velcoity is reduced to one-third its initial value. If the radius of the orbit in the ground state is `r` the radius of new orbit will be
View SolutionEnergy of an electron in an excited hydrogen atom is `-3.4eV`. Its angualr momentum will be: `h = 6.626 xx 10^(-34) J-s`.
View SolutionThe wavelength of the first line of Lyman series in hydrogen atom is `1216`. The wavelength of the first line of Lyman series for `10` times ionized sodium atom will be added
View SolutionEnergy `E` of a hydrogen atom with principle quantum number `n` is given by `E = (-13.6)/(n^(2)) eV`. The energy of a photon ejected when the electron jumps from `n = 3` state to `n = 2` state of hydrogen is approximately
View SolutionIf `13.6 eV` energy is required to ionized the hydrogen atom then the energy required to ionize the hydrogen atom , then the energy required to remove an electron from `n = 2 ` is
View SolutionIn which of the following systems will the radius of the first orbit `(n = 1)` be minimum ?
View SolutionThe radius of the Bohr orbit in the ground state of hydrogen atom is `0.5 Å`. The radius o fthe orbit of the electron in the third excited state of `He^(+)` will be
View SolutionLet `v_(1)` be the frequency of series limit of Lyman series, `v_(2)` the frequency of the first line of Lyman series and `v_(3)` the frequency of series limit of Balmer series. Then which of the following is correct ?
View SolutionThe speed of electron in the second orbit of `Be^(3+)` ion will be
View SolutionThe potential energy of an electron in the fifth orbit of hydrogen atom is
View SolutionIf the radius of an orbit is `r` and the velocity of electron in it is `v` , then the frequency of electron in the orbit will be
View SolutionA sample of hydrogen is bombarded by electrons. Through what potential difference should the electrons be accelerated so that third line of Lyman series be emitted?
View SolutionThe recoil speed of a hydrogen atom after it emits a photon is going form n=5 state to n =1 state is ….. m/s.
View SolutionIf `R` is the Rydberg's constant for hydrogen the wave number of the first line in the Lyman series will be
View SolutionWith the increase in peinciple quantum number, the energy difference between the two successive energy levels
View SolutionIf `lambda_(1) and lambda_(2)` are the wavelength of the first members of the Lyman and Paschen series, respectively , then `lambda_(1) lambda_(2)` is
View SolutionIn figure `E_(1) and E_(2)` represent some of the energy levels of the hydrogen atom.
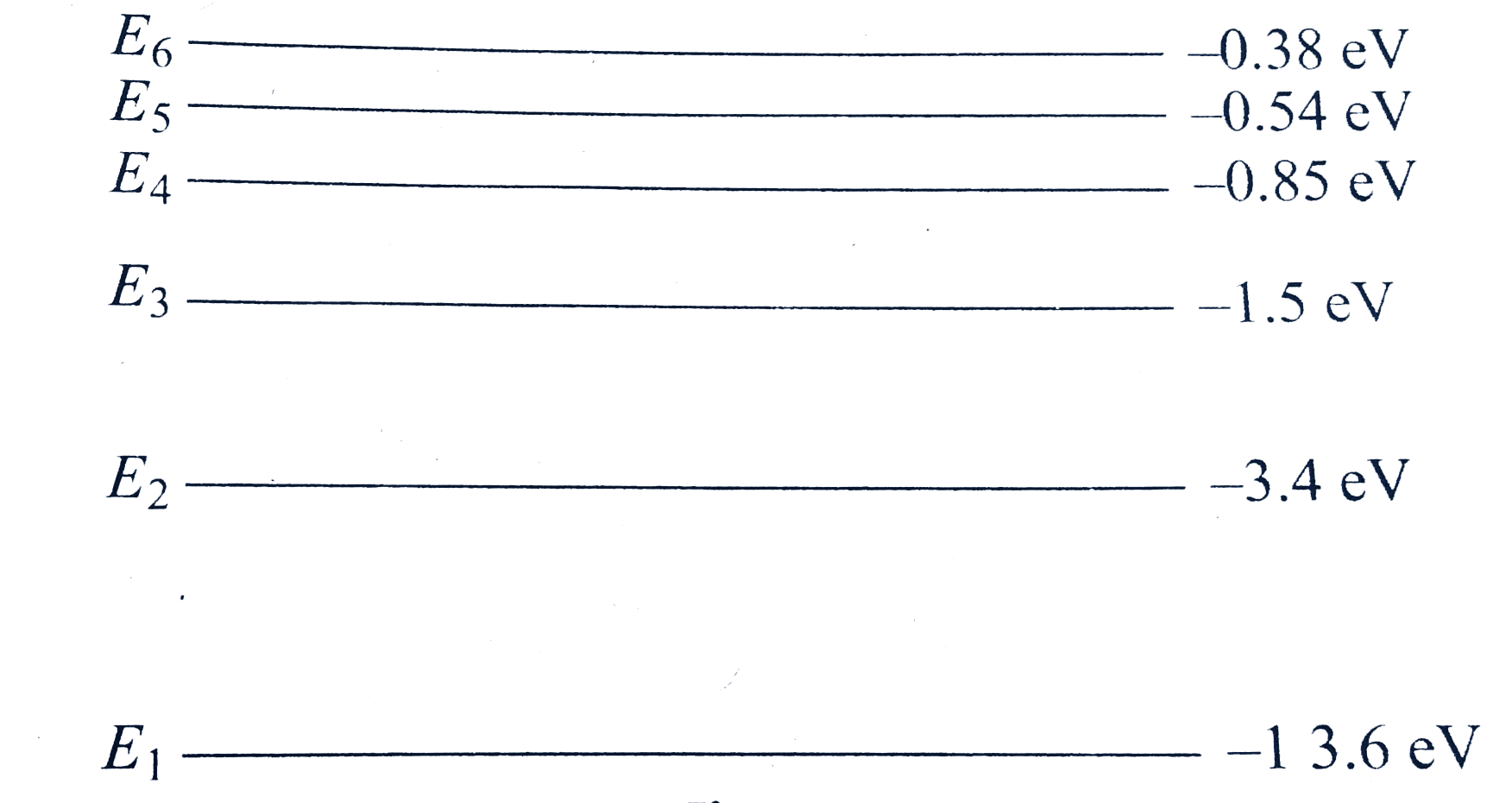
Which one of the following transitions produced a photon of wavelength in the ultraviolet region of the electromagnetic spectrum?
View SolutionWhich of the following parameters are the same for all hydrogen like atoms and ions in their ground state?
View SolutionAn atom emits a spectral line of wavelength `lambda` when an electron makes a transition between levels of energy `E_(1) and E_(2)`. Which expression correctly relates `lambda E_(1) and E_(2)` ?
View SolutionThe frequency of emission line for eny transition in positronium atom (consisting of a positron and an electron) is `x` times the frequency of the corresponding line in the case of `H` atom , where `x` is
View SolutionIf the wavelength of photon emitted due to transition of electron from third orbit to first orbit in a hydrogen atom is `lambda` then the wavelength of photon emitted due to transition of electron from fourth orbit to second orbit will be
View SolutionWhich of the following is true when Balmer gave his model for hydrogen atom?
View SolutionThe shortest wavelength in the Lyman series of hydrogen spectrum is `912 Å` correcponding to a photon energy of `13.6eV`. The shortest wavelength in the Balmer series is about
View SolutionThe ratio of maximum to minimum possible radiation energy Bohr's hypothetical hydrogen atom is equal to
View SolutionA stationary hydrogen atom emits photon corresponding to the first line of Lyman series. If R is the Rydberg constant and M is the mass of the atom, then the velocity acquired by the atom is
View SolutionIn interperting Rutherford's experiments on the scattering of alpha particle by thin foils, one must examine what were known factors, and what the experiment settle. Which of the following are true in this context?
View SolutionAn electron jumps from the fourth orbit to the second orbit hydrogen atom. Given the Rydberg's constant `R = 10^(7) cm^(-1)`. The frequecny , in `Hz` , of the emitted radiation will be
View SolutionGiven mass number of gold `= 197`, Density of gold `= 19.7 g cm^(-1)`. The radious of the gold atom is appoximately:
View SolutionTransition between three energy energy levels in a particular atom give rise to three Spectral line of wavelength , in increasing magnitudes. `lambda_(1), lambda_(2)` and `lambda_(3)`. Which one of the following equations correctly relates `lambda_(1), lambda_(2)` and `lambda_(3)`?
View SolutionThe ratio of the speed of the electron in the first Bohr orbit of hydrogen and the speed of light is equal to (where `e, h and c` have their usual meaning in cgs system)
View SolutionSuppose two deuterons must get as close as `10^(-14) m` in order for the nuclear force to overcome the repulsive electrostatic force. The height of the electrostatic harrier is nearest to
View SolutionAn electron in `H` atom makes a transition from `n = 3 to n = 1`. The recoil momentum of the `H` atom will be
View SolutionA hydrogen-like atom emits rediationof frequency `2.7 xx 10^(15)` Hz` when if makes a transition from `n = 2 to n = 1`. The frequency emitted in a transition from `n = 3 to n = 1` will be
View SolutionThe electron in a hydrogen atom make a transtion `n_(1) rarr n_(2)` where `n_(1) and n_(2)` are the priocipal quantum number of the two states . Assume the Bohr model to be valid . The time period of the electron in the initial state is eight time that in the final state . The possible values of `n_(1) and n_(2)` are
View SolutionAn electron revolving in an orbit of radius `0.5 Å` in a hydrogen atom executes per secon. The magnetic momentum of electron due to its orbital motion will be
View SolutionThe total energy of an electron in the ground state of hydrogen atom is `- 13.6 eV`. The potiential energy of an electron in the ground state of `Li ^(2+)` ion will be
View SolutionA doubly ionized lithium atom is hydrogen like atom with atomic
. number 3. Find the wavelength of the radiation to excite the electron in
. `Li^(2+)` from the first to the third Bohr orbit. The ionization energy of the hydrogen
. Atom is 13.6V.
View SolutionThe force acting on the electron in a hydrogen atom depends on the principal quantum number as
View SolutionThe minimum energy to ionize an atom is the energy required to
View SolutionIf element with particle quantum number `ngt4` were not allowed in nature , the number of possible element would be
View SolutionThe orbital velocity of electron in the ground state is `v`. If the electron is excited to enegry staet `- 0.54 eV` its orbital velocity will be
View SolutionIf potential energy between a proton and an electron is given by `| U | = k e^(2)//2 R^(3)`, where `e` is the charge of electron and `R` is the radius of atom , that radius of Bohr's orbit is given by `(h = Plank's constant, k = constant)`
View SolutionIn hydrogen atom, the transition takes place from `n = 3` to `n = 2`. If Rydberg's constant is `1.09 xx 10` per metre, the wavelength of the limit emitted is
View SolutionAn alpha particle of energy `5 MeV` is scattered through `180^(@)` by a found uramiam nucleus . The distance of closest approach is of the order of
View SolutionHow many time does the electron go round the first bohr orbit of hydrogen atoms in `1 s`?
View SolutionThe radius of hydrogen atom in the ground state is `5.3 xx 10^(-11)` m. When struck by an electron, its radius is found to be `21.2 xx 10^(-11) m`. The principal quantum number of the final state will be
View SolutionThe wavelength of the first line of Balmer series is `6563 Å`. The Rydberg's constant is
View SolutionA hydrogen atom and a `Li^(2+)` ion are both in the second excited state. If `l_H` and `l_(Li)` are their respective electronic angular momenta, and `E_H and E_(Li)` their respective energies, then
(a) `l_H gt l_(Li) and |E_H| gt |E_(Li)|`
(b) `l_H = l_(Li) and |E_H| lt |E_(Li)|`
(C ) `l_H = l_(Li) and |E_H| gt |E_(Li)|`
(d) `l_H lt l_(Li) and |E_H| lt|E_(Li)|`
View SolutionImagine an atom made up of a proton and a hypothetical particle of double the mass of the electron but having the same charge as the electron . Apply the Bohr atom model and consider all possible transitions of this hypothetical particle that will be emitted level . The longest wavelength photon that will be emitted has longest wavelength `lambda` (given in terms of the Rydberg constant `R` for the hydrogen atom) equal to
View SolutionThe electric potential between a proton and as electron is given by `V= V_(0) ln (r )/(r_(0))` , where `r_(0)` is a constant . Assuming Bohr's model to be applicable , write variation of `r_(n)` with `n,n` being the principal quantum number ?
View SolutionIn order to break a chemical bond in the molecules of human skin, causing sunburn , a photon energy of about `3.5 eV` is required. This corresponds to wavelength in the
View SolutionThe longest wavelength that the singly ionized helium atom in its ground state will obsorb is
View SolutionThe electron in a hydrogen atom make a transtion `n_(1) rarr n_(2)` where `n_(1) and n_(2)` are the priocipal quantum number of the two states . Assume the Bohr model to be valid . The time period of the electron in the initial state is eight time that in the final state . The possible values of `n_(1) and n_(2)` are
View SolutionIn which of the following transition will the wavelength be minimum ?
View SolutionA photon collides with a stationary hydrogen atom in ground state inelastically. Energy of the colliding photon is 10.2 eV. After a time interval of the order of micro second another photon collides with same hydrogen atom inelastically with an energy of 15eV. What will be observed by the detector?
View SolutionThe shortest wavelength of the Brackett series of a hydrogen-like atom (atomic number of `Z` ) is the same as the shortest wavelength of the Balmer series of hydrogen atom. The value of `z` is
View SolutionIn a hypothetical system , a partical of mass `m` and charge `-3 q` is moving around a very heavy partical chaRGE `q`. Assume that Bohr's model is applicable to this system , then velocuity of mass `m` in the first orbit is
View SolutionThe angular momentum of an electron in a hydrogen atom is proportional to
View SolutionThe rartio `("in" S 1 units)` of magnetic dipole moment to that of the angular momentum of an electron of mass `m kg` and charge `e` coulomb in Bohr's orbit of hydrogen atom is
View SolutionIf an electron in `n = 3` orbit of hydrogen atom jumps down to `n = 2` orbit, the amount of energy reeased and the and the wavelength of radiation emitted are
View SolutionIn hydrogen spectrum, the shortest wavelength in Balmer series is the `lambda`. The shortest wavelength in the Brackett series will be
View SolutionThe minimum orbital angular momentum of the electron in a hydrogen atom is
View SolutionThe first excitation potential of a hypothetical hydrogen- like atom is `15 V`. Find the third excitation potential of the atom.
View SolutionTwo hydrogen atoms are in excited state with electrons in`n=2` state.First one is moving to wards left and emits a photon.' of energy `E_(1)` towards right. Second one is moving towards right with same speed and emits a photon of energy `E_(2)` towards right. Taking recoil of nucleus.into account during_emission process :
View SolutionIn a hydrogen atom following Bohr's postulates , the product of linear momentum and angular momentum is proportional mass of photon emitted by the X-ray
View SolutionThe voltage applied to an X-ray tube is `18 kV`. The maximum mass of photon emitted by the X-ray tube will be
View SolutionThe wavelength of `K_(alpha)` X-rays of two metals `A and B` are` 4 // 1875 R and 1// 675 R`, respectively , where `R` is rydberg 's constant. The number of electron lying between `A and B` according to this lineis
View SolutionOne of the lines in the emission spectrum of `Li^(2 +)` has the same wavelength as that of the second line of Balmer series in hydrogen spectrum. The electronic transition correspnding to this line is.
View SolutionThe photon radiated from hydrogen corresponding to the second line of Lyman series is absorbed by a hydrogen like atom X in the second excited state. Then, the hydrogen-like atom X makes a transition of nth orbit.
View SolutionThe element which has a `K_(alpha)` X-rays line of wavelength `1.8 Å` is
`(R = 1.1 xx 10^(7) m^(-1), b = 1 and sqrt(5//33) = 0.39)`
View SolutionWhen an electron acceleration by potential different `U` is bombarded on a specific metal, the emitted X-ray spectrum obtained is shown in figure . If the potential different is reduced to `U//3` , the correct spectrum is

View SolutionIn a hydrogen atom , the electron atom makes a transition from `n = 2 to n = 1`. The magnetic field produced by the circulating electron at the nucleus
View SolutionAn electron in a hydrogen atom makes a transition from first excited state to ground state . The equivelent currect due to circulating electron :
View Solutionwhen the voltage applied to an X-ray tube increases from `V_(1) = 10 kV to V_(2) = 20 kV`, the wavelenght interval between K_(alpha)` line and cut-off wavelenght of continuous spectrum increases by a factor of `3`. Atomic number of the metallic target is
View SolutionMonochromatic radiation of wavelength `lambda` is incident on a hydrogen sample in ground state. hydrogen atoms absorb a fraction of light and subsequently and radiation of six different wavelength .Find the value of `lambda`
View Solution
Single Correct questions and complete solutions for chapter ATOMIC PHYSICS of CENGAGE PHYSICS (HINGLISH) of Class 12