Complete Solutions to EXERCISE-1 CONCEPT BUILDER of chapter MECHANICAL PROPERTIES OF FLUIDS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | MECHANICAL PROPERTIES OF FLUIDS |
| DISHA PUBLICATION | EXERCISE-1 CONCEPT BUILDER |
| Number Of Questions | 59 |
| Academic Year | 2023-24 |
The pressure at the bottom of a tank containing a liquid does not depend on
View SolutionHow does liquid pressure depend on the depth of a point below the surface of a liquid
View SolutionAn egg when placed in ordinary water sinks but floats when placed in brine. This is because
View SolutionA common hydrometer reads specific gravity of liquids Compared to the 1.6 mark of the stem the mark 1.5 will be
View SolutionThe spring balance A reads 2 kg with a block of mass m suspended from it. A balance B reads 5 kg when a beaker with liquid is put on the pan of the balance. The two balances are now so arranged that the hanging mass is inside the liquid in a beaker as shown in fig. Then

View SolutionThe density of ice is `xgm//c c` and that of water is `y gm//c c`. What is the change in volume in `c c`, when `m gm` of ice metls?
View SolutionA small lead shot is embedded in a big lump of ice floating in a jar of water. The level of water in the jar is noted. When all the ice melts down, the level of water in the jar would
View SolutionThe pressure at depth h below the surface of a liquid of density `rho` open to the atmosphere is
View SolutionThe force acting on a window of are 50`xx`50 cm of a submarine at a depth of 2000 m in an ocean ,the interior of which is maintained at sea level atmospheric pressure is (density of sea water = `10 ^(3) kg m^(-3)` ,g =`10 m s^(-2)` )
View SolutionA wooden block, with a coin placed on its top, floats in water as shown in fig. the distance 1 and h are shown there. After some time the coin falls into the water. Then

View SolutionThe density `rho` of water of buk modulus B at a depth y then ocean is related to the density at surface `rho_(0)` by the relation
View SolutionA large block of ice `5 m` thick has a vertical hole drilled through it and is floating in the middle of a lake. What is the minimum length of a rope required to scoop up a bucket full of water through the hole? Relative density of ice `=0.9`.
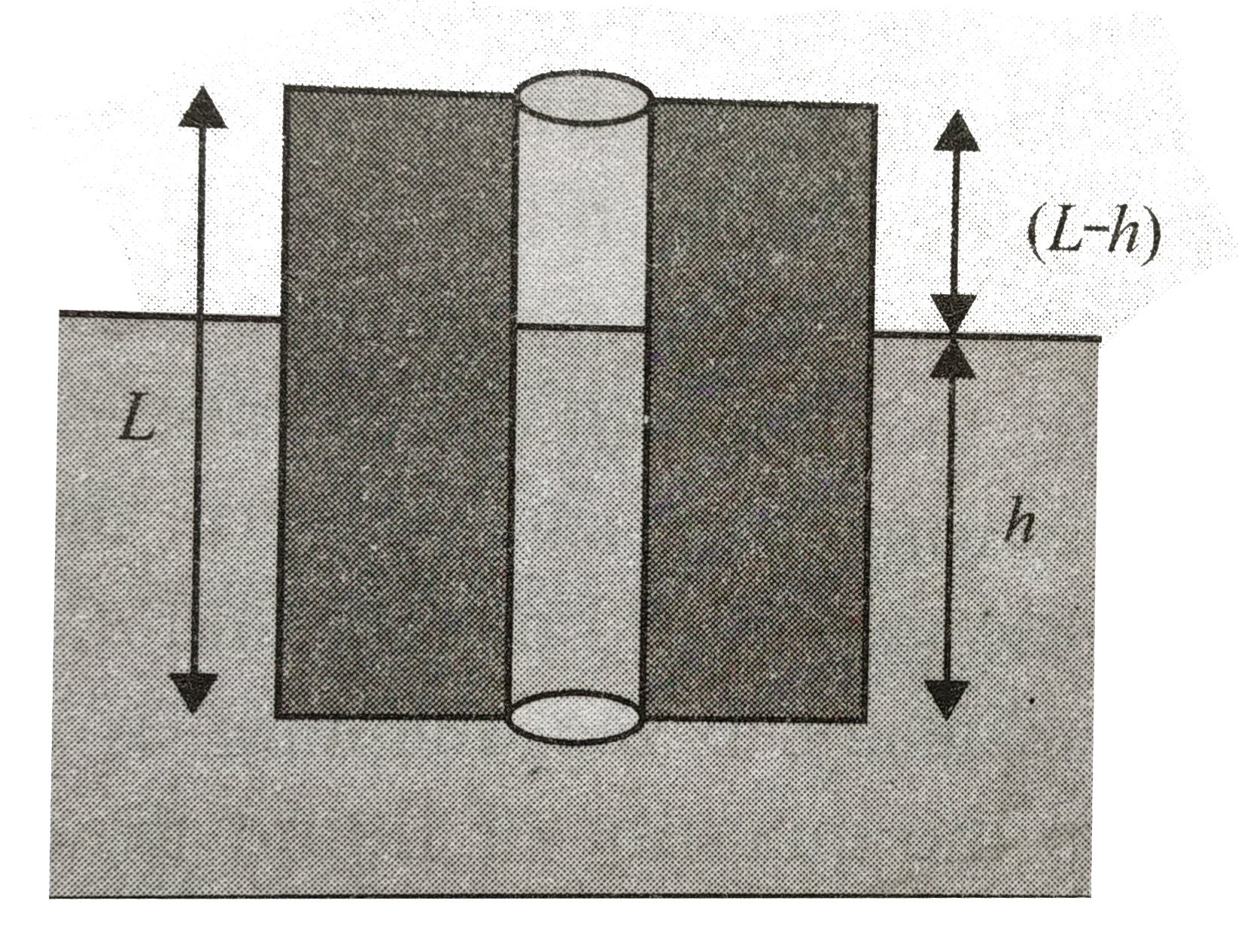
View SolutionA sphere of solid material of specific gravity `8` has a concentric spherical cavity and just sinks in water. The ratio of radius of cavity to that of outer radius of the sphere must be
View SolutionA metallic sphere weighs `210g` in air, 180 g in water and 120 g in an unknown liquid. Find the density of metal and of liquid.
View SolutionAn iceberg is floating partly immersed in sea water, the density of sea water is `1.03 g cm^(-3)` and that of ice is `0.92 g cm^(-3)`. The fraction of the total volume of the iceberg above the level of sea water is
View SolutionA boat having a length of 3 m and breadth of 2 m is floating on a lake. The boat sinks by 1 cm when a man gets on it. The mass of the mas is:
View SolutionA body of density `rho` is dropped from reat from a height h into a lake of density `sigma(sigmagtrho)`. The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
View SolutionA vessel contains oil (density `=0.8gm//cm^3`) over mercury (density `=13.6gm cm^3`). A homogeneous sphere floats with half its volume immersed in mercury and the other half in oil. The density of the material of the sphere in `gm//cm^3` is
View SolutionA sphere of solid material of relative density 9 has a concentric spherical cavity and sinks in water. If the radius of the sphere be R. Then the radius of the cavity (r) will be related to R as
View SolutionA pieceo of ice is floating in water . Find the fraction of volume of he piece of ice outside the water
(Given density of ice `=900 kg//m^(3)` and density of water `=1000 kg//m^(3))`
View SolutionIn car lift compressed air exerts a force `F_1` on a small piston having a radius of 5 cm. This pressure is transmitted to a second piston of radius 15 cm. If the mass of the car to be lifted is 1350 kg, what is `F_1 ?` What is the pressure necessary to ac complish this task ?
View SolutionA cone full of water, is placed on its side on a horizontal table, the thrust on its base is x times the weight of the contained fluid, where `2alpha` is the vertical angle of the cone. Find the value of x.
View SolutionA narrow tube completely filled with a liquid is lying on a series of cylinders as shown in figure.
Assuming no sliding between any surfaces, the value of acceleration of the cylinders for which liquid will not come out of the tube from anywhere is given by

View SolutionThe total weight of a piece of wood is 6 kg in the floating state in water its 1/3 part remains inside the water on this floating solid what maximum weight is to be put such that the whole of the piece of wood is to be drowned in the water
View SolutionWater is flowing continuously from a tap having an internal diameter `8xx10^(-3)`m. The water velocity as it leves the tap is `0.4 ms^(-1)`. The diameter of the water stream at a distance `2xx10^(-1)`m below the tap is close to `(g=10m//s^(2))`
View SolutionThe cylinderical tube of a spray pump has radius R, One end of which has n fine holes, each of radius r. If the speed of the liquid in the tube is V, the speed of the ejection of the liquid through the holes is :
View SolutionA fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct?
View SolutionA cylindrical vessel contains a liquid of density `rho` up to height `h`. The liquid is closed by a piston of mass `m` and area of cross section `A`. There is a small hole at the bottom of the vessel. The speed `v` with which the liquid comes out of the hole is

View SolutionOil is filled in a cylindrical container up to height 4m. A small hole of area 'p' is punched in the wall of the container at a height 1.52m from the bottom. The cross sectional area of the container is Q. If`p/q = 0.1` then v is (where vis the velocity of oil coming out of the hole)
View SolutionFigure shows a liquid flowing through a tube at the rate of `0.1 m^(3)//s.` The tube is branched into two semicircular tubes of cross - sectional area A/3 and 2A/3. The velocity of liquid at Q is (the cross-section of the main tube is `A= 10^(-2) m^(2) and v_(p) = 20 m//s)`

View SolutionA cylinder of height 20m is completely filled with water. The velocity of effux of water `(in ms^(-1))` through a small hole on the side wall of the cylinder near its bottom is
View SolutionAtank is filled with water upto a height H. Water is allowed to come out of a hole P in one of the walls at a depth h below the surface of water (see fig.) Express the horizontal distance X in terms Hand h.

View SolutionThere is a small hole at the bottom of tank filled with water. If total pressure at the bottom is `3 atm(1 atm=10^(5)Nm^(-2))`, then find the velocity of water flowing from hole.
View SolutionIn the figure, the velocity `V_(2)` will be

View SolutionThree tubes X, Y and Z are connected to a horizontal pipe in which ideal liquid is flowing. The radii of the tubes X, Y and Z at the junction are respectively 3 cm, 1 cm and 3 cm. It can be said

View SolutionAir flows horizontally with a speed v= 106 km/hr. A house has plane roof of area `A = 20m^(2)` The magnitude of aerodynamic lift of the roof is
View SolutionA vessel of area of cross-section A has liquid to a height H . There is a hole at the bottom of vessel having area of cross-section a . The time taken to decrease the level from `H_(1)` to `H_(2)` will sec
View SolutionWater is flowing through a horizontal tube having crosssectional areas of its two ends being A and A' such that the ratio All' is 5. If the pressure difference of water between the two ends is `3 xx 10^(5) Nm^(-2)` the velocity of water with which it enters the tube will be (neglect gravity effects)
View SolutionWhich of the following statement(s) is/are true?
View SolutionIf the terminal speed of a sphere of gold (density `=19.5kg//m^3`) is `0.2m//s` in a viscous liquid (density `=1.5kg//m^3`), find the terminal speed of a sphere of silver (density `=10.5kg//m^3`) of the same size in the same liquid
View SolutionWhat is the velocity v of a metallic ball of radius r falling in a tank of liquid at the instant when its acceleration is one-half that of a freely falling body ? (The densities of metal and of liquid are r and s respectively, and the viscosity of the liquid is `eta`).
View SolutionA rain drop of radius 0.3 mm has a terminal velocity in `"air" = 1 "m/s"`. The viscosity of air is `8 xx 10^(–5)` poise. The viscous force on it is
View SolutionA spherical ball of iron of radius 2 mm is falling through a column of glycerine. If densities of glycerine and iron are respectively `1.3 xx 103 "kg/m"^(3)" and" 8 xx 103 "kg/m"^(3)`. `eta` for glycerine = `0.83 "Nm"^(–2)` sec, then the terminal velocity
View SolutionA small spherical ball falling through a viscous medium of negligible density has terminal velocity v. Another ball of the same mass but of radius twice that of the earlier falling through the same viscous medium will have terminal velocity
View SolutionTwo drops of the same radius are falling through air with a steady velcoity of `5 cm s^(-1).` If the two drops coalesce, the terminal velocity would be
View SolutionA lead shot of a 1mm diameter falls through a long column of glycerine.The variation of its velocity `v` with distance covered is represented by,
View SolutionWhen a ball is released from rest in a very long column of viscous. Liquid its downwards acceleration is `a` (just after release). Then its acceleration when it has acquired two third of the maximum velocity:
View SolutionA ball of radius r and density p falls freely under gravity through a distance h before entering water. Velocity of ball does not change even on entering water. If viscosity of water is n the value of his given by

View SolutionA small ball (mass m) falling under gravity in a viscous medium experience a drag force proportional to the instantaneous speed u such that `F_(drag)=ku`. Then the terminal speed of ball within viscous medium is
View SolutionAn air bubble of radius 1 cm rises with terminal velocity 0.21 cm/s in liquid column. If the density of liquid is `1.47 xx 10^(3) kg//m^(3).` Then the value of coefficient of viscosity of liquid ignoring the density of air, will be
View SolutionTwo soap bubbles `A` and `B` are kept in a closed chamber where the air is maintained at pressure `8 N//m^(2)`. The radii of bubbles `A` and `B` are `2 cm` and `4 cm`, respectively. Surface tension of the soap. Water used to make bubbles is `0.04 N//m`. Find the ratio `n_(B)//n_(A)`, where `n_(A)` and `n_(B)` are the number of moles of air in bubbles `A` and `B` respectively. [Neglect the effect of gravity.]
View SolutionLet `T_(1)` be surface tension between solid and air, `T_(2)` be the surface tension between solid and liquid and T be the surface tension between liquid and air. Then in equilibrium, for a drop of liquid on a clean glass plate, the relation is (`theta` is angle of contact)

View SolutionAn isolated and charged spherical soap bubble has a radius r and the pressure inside is atmospheric. IfT is the surface tension of soap solution, then charge on drop is` Xpi sqrt(2r T epsi _(0))` then find the value of X.
View SolutionThe rise in the water level in a capillary tube of radius 0.07 cm when dipped veryically in a beaker containing water of surface tension `0.07 N m^(-1)` is (g = `10 m s^(-2)`)
View SolutionIf two glass plates have water between them and are separated by very small distance (see figure), it is very difficult to pull them apart. It is because the water in between forms cylindrical surface on the side that gives rise to lower pressure in the water in comparison to atmosphere. If the radius of the cylindrical surface is R and surface tension of water is T then the pressure in water between the plates is lower by

View SolutionWater rises to a height of 10 cm in capillary tube and mercury falls to a depth of 3.112 cm in the same capillary tube. If the density of mercury is 13.6 and the angle of contact for mercury is `135^(@)`, the ratio of surface tension of water and mercury is
View SolutionA water film is formed between two parallel wires of 10 cm length. The distance of 0.5 cm between the wires is increased by 1mm. What will be the work done ? (Given, surface tension of water of `72xx10^(-3) Nm^(-1)`)
View SolutionA film of water is formed between two straight parallel wires of length 10 cm each separated by `0.5cm` If their separation is increased by `1mm` while still maintaining their parallelism, how much work will have to be done (Surface tension of water `=7.2xx10^-2(N)/(m)`)
View SolutionA glass capillary tube of inner diameter 0.28 mm is lowered vertically into water in a vessel. The pressure to be applied on the water in the capillary tube so that water level in the tube is same as the vessel in `(N)/(m^(2))` is (surface tension of water `=0.07(N)/(m)` atmospheric pressure `=10^(5)(N)/(m^(2))`
View Solution
EXERCISE-1 CONCEPT BUILDER questions and complete solutions for chapter MECHANICAL PROPERTIES OF FLUIDS of DISHA PUBLICATION PHYSICS (HINGLISH) of Class 12