Complete Solutions to EXERCISE-2: CONCEPT APPLICATOR of chapter ATOMS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ATOMS |
| DISHA PUBLICATION | EXERCISE-2: CONCEPT APPLICATOR |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
If the series limit wavelength of the Lyman series for hydrogen atom is `912 Å`, then the series limit wavelength for the Balmer series for the hydrogen atom is
View SolutionAn `alpha`-particle with kinetic energy `K` is heading towards a stationary nucleus of atomic number `Z`. Find the distance of the closest approach.
View SolutionUltraviolet light of wavelength `lambda_(1)` and `lambda_(2)` (with `lambda_(2) gt lambda_(1)`) when allowed to fall on hydrogen atoms in their ground state is found to liberate electrons with kinetic energies `E_(1)` and `E_(2)` respectively. The value of the planck's constant can be found from the relation
View SolutionThe difference between the longest wavlength line of the Balmar series and shortest wavelength line of the Lyman series for a hydrogen like atom (atomic number Z) equal to Deltalambda. The value of the Rydberg constant for the given atom is
View SolutionIonization potential of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1 eV. According to Bohr's theory, the spectral lines emitted by hydrogen will be
View SolutionElectrons in a certain energy level `n=n_(1)` can emit 3 spectral lines. When they are in another energy level, `n=n_(2)`, they can emit 6 spectral lines. The orbital speed of the electrons in the two orbits are in the ratio
View SolutionThe electron in a hydrogen atom jumps from ground state to the higher energy state where its velcoity is reduced to one-third its initial value. If the radius of the orbit in the ground state is `r` the radius of new orbit will be
View SolutionIn a hypothetical system , a partical of mass `m` and charge `-3 q` is moving around a very heavy partical chaRGE `q`. Assume that Bohr's model is applicable to this system , then velocuity of mass `m` in the first orbit is
View SolutionOne of the lines in the emission spectrum of `Li^(2+)` has the same wavelength as that of the 2nd line of Balmer series in hydrogen spectrum. The electronic transition corresponding to this line is n = 12 `to`n=x. Find the value of x.
View SolutionThe wavelength of the first spectral line in the Balmer series of hydrogen atom is 6561 Å. If the wavelength of the spectral line in the Balmer series of singly-ionized helium atom is 1215 when electron jumps from `n_2` to `n_1`, then `n_2` and `n_1`, are
View SolutionA 12.5eV electron beam is used to bombard gaseous hydrogen at room temperature What series of wavelengths will be emitted.
View SolutionOrbits of a particle moving in a circle are such that the perimeter of the orbit equals an integer number of de-Broglie wavelengths of the particle. For a charged particle moving in a plane perpendicular to a magnetic field, the radius of the `n^(th)` orbital will therefore be proportional to:
View SolutionA stationary hydrogen atom emits photon corresponding to the first line of Lyman series. If R is the Rydberg constant and M is the mass of the atom, then the velocity acquired by the atom is
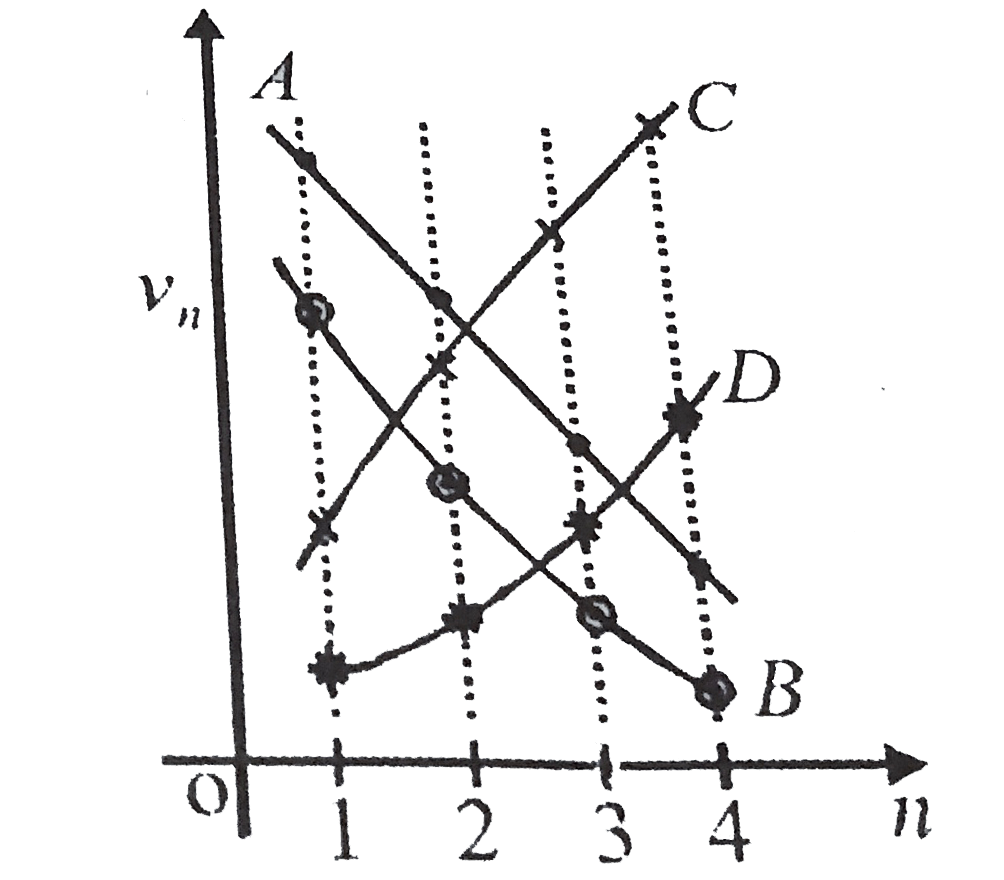
View SolutionWhich of the plots shown in the figure represents speed `(v_n)` of the electron in a hydrogen atom as a function of the principal quantum number (n)?
View SolutionThe diagram shows the energy levels for an electron in a certain atom. Which transition shown represents the emission of photon with the most enegy ?

View SolutionIn the Bohr model an electron moves in a circular orbit around the proton. Considering the orbiting electron to be a circular current loop, the magnetic moment of the hydrogen atom, when the electron is in nth excited state, is :
View SolutionAn electron , in a hydrogen-like atom, is in excited state. It has a total energy of -3.4 eV. Calculate
(a) the kinetic energy and
(b) the de Broglie wavelength of the electron.
View SolutionThe ionization energy of a hydrogen like Bohr atom is 4 Rydbergs. If, `lamda_1`is the wavelength of the radiation emitted when the electron jumps from the first excited state to the ground state then
View SolutionThe wavelength of `K_(alpha)` X-rays of two metals `A and B` are` 4 // 1875 R and 1// 675 R`, respectively , where `R` is rydberg 's constant. The number of electron lying between `A and B` according to this lineis
View SolutionSuppose an electron is attracted toward the origin by a force`(k)/(r )` where `k` is a constant and `r` is the distance of the electron from the origin .By applying Bohr model to this system the radius of the `n^(th)` orbital of the electron is found to be `r_(n)` and the kinetic energy of the electron to be `T_(n)` , Then which of the following is true ?
View SolutionIn the Bohr model of a `pi-mesic` atom , a `pi-mesic` of mass `m_(pi)` and of the same charge as the electron is in a circular orbit of ratio of radius `r` about the nucleus with an orbital angular momentum `h//2 pi`. If the radius of a nucleus of atomic number `Z` is given by `R = 1.6 xx 10^(-15) Z^((1)/(3)) m`, then the limit on `Z` for which `(epsilon_(0) h^(2)//pi me^(2) = 0.53 Å and m_(pi)//m_(e) = 264) pi-mesic` atoms might exist is
View SolutionIf in hydrogen atom, radius of `n^(th)` Bohr orbit is `r_n`., frequency of revolution of electron in `n^(th)` orbit is `f_n` choose the correct option.
View SolutionIn the Bohr model of a hydrogen atom, the centripetal force is furnished by the Coulomb attraction between the proton and the electrons. If `a_(0)` is the radius of the ground state orbit, `m` is the mass and `e` is the charge on the electron and `e_(0)` is the vacuum permittivity, the speed of the electron is
View SolutionAn electron in the hydrogen atom jumps from excited state `n` to the ground state. The wavelength so emitted illuminates a photo-sensitive material having work function `2.75eV`. If the stopping potential of the photoelectron is `10V`, the value of `n` is
View SolutionIn the Bohr's model of hydrogen-like atom the force between the nucleus and the electron is modified as `F=(e^2)/(4 pi epsi_0)((1)/(r^2)+(beta)/(r^3))`, where B is a constant. For this atom, the radius of the nth orbit in terms of the Bohr radius `(a_0 = ( epsi_0 h^2)/(m pi e^2))` is :
View Solutionthe wavelength of radiation emitted is `lambda_0` when an electron jumps. From the third to second orbit of hydrogen atom. For the electron jumping from the fourth to the second orbit of the hydrogen atom, the wavelength of radiation emitted will be
View SolutionIn Bohr's model of the hydrogen atom, let R, V, T and E represent the radius of the orbit, speed of the electron, time period of revolution of electron and the total energy of the electron respectively. The quantity proportional to the quantum number n is
View SolutionAn electron in hydrogen atom makes a transition `n_i to n_2` where `n_1` and `n_2` are principal quantum numbers of the two states. Assuming Bohr's model to be valid the time period of the electron in the initial state is eight times that in the final state. The possible values of `n_1` and `n_2` are
View SolutionThe ionization enegry of the electron in the hydrogen atom in its ground state is `13.6 ev`. The atoms are excited to higher energy levels to emit radiations of `6` wavelengths. Maximum wavelength of emitted radiation corresponds to the transition between
View SolutionIf potential energy between a proton and an electron is given by `| U | = k e^(2)//2 R^(3)`, where `e` is the charge of electron and `R` is the radius of atom , that radius of Bohr's orbit is given by `(h = Plank's constant, k = constant)`
View Solution
EXERCISE-2: CONCEPT APPLICATOR questions and complete solutions for chapter ATOMS of DISHA PUBLICATION PHYSICS (HINGLISH) of Class 12