Complete Solutions to PART-III of chapter SAMPLE PAPER -12 (UNSOLVED) of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | SAMPLE PAPER -12 (UNSOLVED) |
| FULL MARKS | PART-III |
| Number Of Questions | 22 |
| Academic Year | 2023-24 |
Show that the points represented by the complex numbers `7+9i`, `-3+7i`, `3+3i` from a right angled triangle on the Argand diagram.
View SolutionProvet that a stralght line and parabola connot intersect at more than two points.
View SolutionFind the value of `cos(cos^(-1)((4)/(5))+sin^(-1)((4)/(5)))`
View SolutionDetermine whether the points ( -2,1) ,(0,0) and ( -4,-3) lie outside, on or inside the circle ` x^(2) + y^(2) - 5x + 2y - 5 =0`
View SolutionProve that `[vecaxxvecb,vecbxxvecc,veccxxveca]=[veca,vecb,vecc]^(2)`
View SolutionFind the intervals of monotonicity and local extrema of the function `f(x)=x logx+3x`
View SolutionIf `u(x,y) = (x^(2) + y^(2))/sqrt(x+y)`, prove that `x(del u)/(del x) + y(del u)/(del y) = 3/2 u`.
View SolutionFour defective oranges are accidentally mixed with sixteen good ones. Two oranges are drawn at random from the mixed lot. If the random variable 'X' denotes the number of defective oranges, then find the values of 'X' and number of points in its inverse image.
View SolutionShow that `not(p^^q)equiv((notp)vv(notq))`
View SolutionFind the rank of each of the following matrices: (i) `[{:(3,2,5),(1,1,2),(3,3,6):}]` (ii) `[{:(4,3,1,-2),(-3,-1,-2,4),(6,7,-1,2):}]`
View SolutionIn a T20 match, Chennai Super Kings needed just 6 runs to win with 1 ball left to go in the last over. The last ball was bowled and the batsman at the crease hit it high up. The ball traversed along a path in a vertical plane and the equation of the path is `y=ax^(2)+bx+c` with respect to a xy -coordinate system in the vertical plane and the ball traversed through the points (10,8),(20,16),(40,22), can you conclude that Chennai Super Kings won the match?
Justify your answer. (All distances are measured in metres and the meeting point of the plane of the path with the farthest boundary line is (70,0).)
View SolutionProve that `(1+costheta+isintheta)^(n)+(1+costheta-isintheta)^(n)=2^(n+1)cos^(n)((theta)/(2))cos((ntheta)/(2))`
View SolutionSolve `(x-4)(x+2)(x+3)(x-3)+8=0`
View SolutionProve thate
`tan^(-1)((2)/(11))+tan^(-1)((7)/(24))=tan^(-1)((1)/(2))`
View SolutionA road bridge over an irrigation canal have two semi circular vents each with a span of 20 m and the supporting pillars of width 2m. Use figure to write the equations that represent the semi verticular vents.
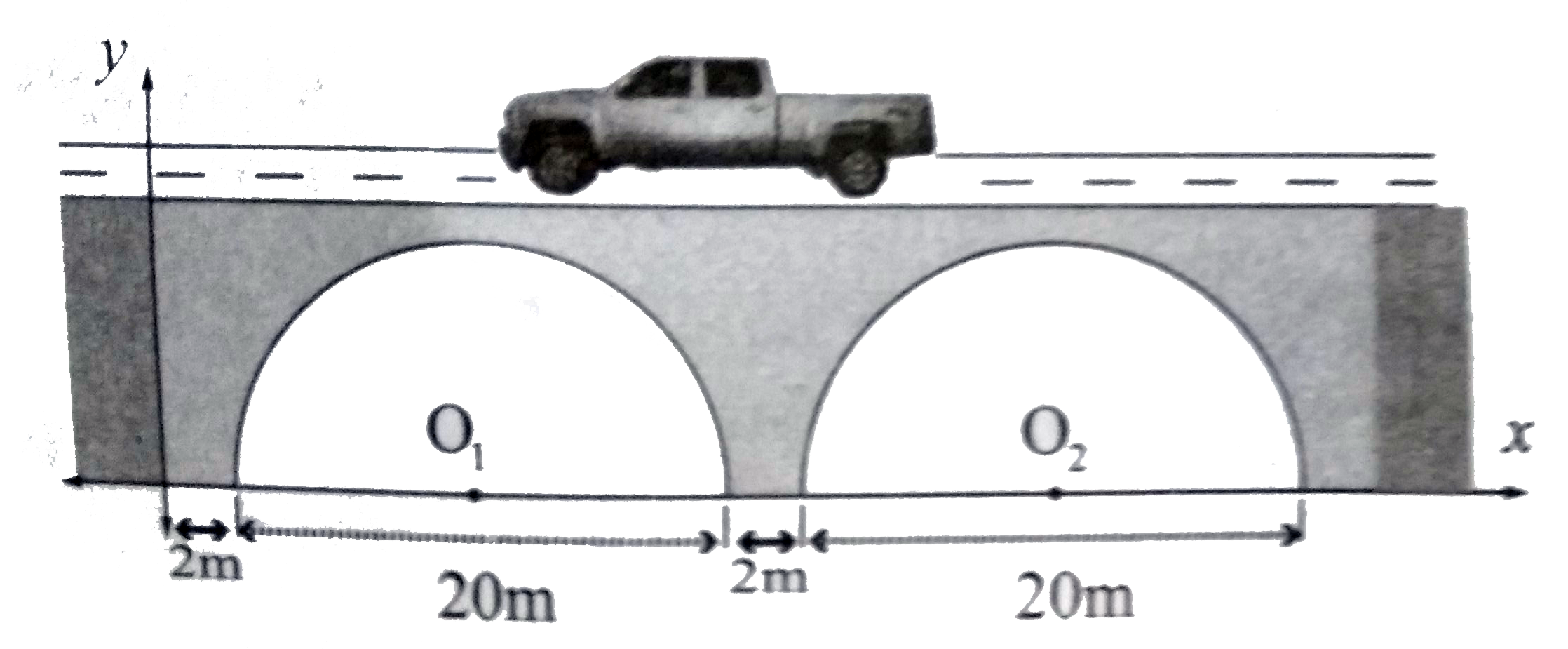
View SolutionShow that the set `G={a+bsqrt2//a,binQ}` is an infinite abelian group with respect to Binary operation addition. Satisfies closure, associative, identity and inverse properties.
View SolutionFind the parametric form of vector equation, and Cartesian equations of the plane containing the line `vecr(hati-hatj+3hatk)+t(2hati-hatj+4hatk)` and perpendicular to the plane `vecr.(hati+2hatj+hatk)=8`.
View SolutionThe mean and standard deviation of a binomial variate X are respectively 6 and 2. Find (i) the probability mass function (ii) P(X=3) (iii) `P(X ge 2)`.
View SolutionFind the intervals of concavity and the points of inflection of the function. `y=12x^(2)-2x^(3)-x^(4)`
View SolutionFor each of the functions find the `f _(x), f _(y),` and show that `f _(xy) = f _(yx).`
`f (x,y) = tan ^(-1)"" ((x)/(y))`
View Solution`(dy)/(dx)+(y)/(x)=sinx`
View SolutionEvaluate: `int_(pi//6)^(pi//3)(dx)/(1+sqrtcotx)`
View Solution
PART-III questions and complete solutions for chapter SAMPLE PAPER -12 (UNSOLVED) of FULL MARKS MATHS (TAMIL ENGLISH) of Class 12