Doubtnut Promotions Sticky

Complete Solutions to OBJECTIVE QUESTIONS of chapter रैखिक प्रोग्रामन of Class 12 book with complete answers and questions

Class 12MATHS
Chapterरैखिक प्रोग्रामन
KC SINHA HINDIOBJECTIVE QUESTIONS
Number Of Questions5
Academic Year2023-24

  1. The corner pont sof the feasible region determined by the followign system of linear inequalities `: 2x+yle10,x+3yle15,xyge0` are `(0,0),(5,0),(3,4)` and `(0,5)`. Let `Z=px+qy` where `p,qge0`. Conditions on `p` and `q` so that the maximum of Z occurs at both `(3,4)` and `(0,5)` is

    View Solution

  2. The maximum value of `z=x+3y` such that `2x+yle20,x+2yle20,xge0,yge0` is

    View Solution

  3. The maximum valueof `z=6x+8y` subjected to constraings `2x+yle30, x+2yle24, xge0, yge0` is

    View Solution

  4. By graphical method, the solutionn of the linear programming problem. Maximize `z=3x_(1)+5x_(2)` subject to `3x_(1)+2x_(2)le18, x_(1)le4,x_(2)le6,x_(1),x_(2)ge0` is

    View Solution

  5. Shaded region is represented by

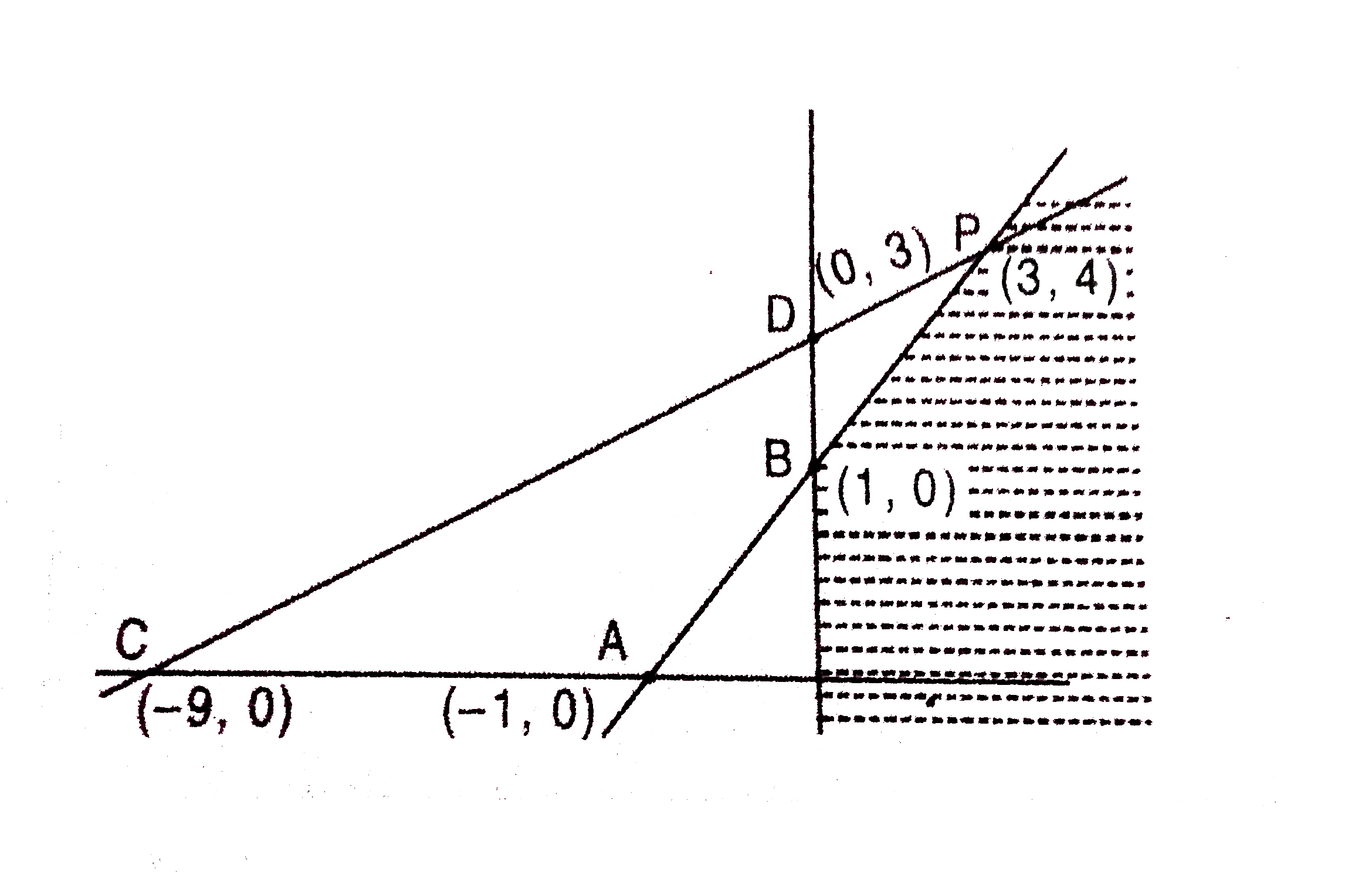
    View Solution

OBJECTIVE QUESTIONS questions and complete solutions for chapter रैखिक प्रोग्रामन of KC SINHA MATHS (HINDI) of Class 12

All Exercises from KC SINHA HINDI Class 12 MATHS रैखिक प्रोग्रामन