Complete Solutions to MULTIPLE CHOICE QUESTIONS of chapter LINEAR PROGRAMMING of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | LINEAR PROGRAMMING |
| KUMAR PRAKASHAN | MULTIPLE CHOICE QUESTIONS |
| Number Of Questions | 42 |
| Academic Year | 2023-24 |
Solution set of the inequality `2x+y gt 5` is ………
01:33View SolutionThe optimal value of the objective function is attained at the points ……..
01:13View SolutionThe region formed by the inequalities `x, y ge 0, y le 6, x+y le 3` is ………
01:53View SolutionThe corner points of the feasible region are (0, 0), (16, 0), (8, 12), (0, 20). The maximum and minimum values of Z = 22x + 18y are m and n respectively then m + n = ………
02:02View SolutionFor linear programming `x+2y ge 10, 3x+4y le 24" and "x ge 0, y ge 0 ………` is not the corner point of feasible region.
03:16View SolutionThe region represented by the inequation `x-y le -1, x-y le 0, x le 0, y le 0` is …………….
02:15View SolutionLet x and y are optimal solution of a LPP, then …
02:21View SolutionThe feasible solution of LPP ………..
01:25View SolutionWhich of the following statement is correct ?
02:57View SolutionConsider a LPP given by minimise Z = 6x + 10y. Subject to `x ge 6, y ge 2, 2x+y ge 10, x ge 0, y ge 0`. Redundant constraints in this LPP are ………..
04:20View SolutionObjective function of a LPP is …………
01:14View SolutionThe maximum value of Z = x + 4y subject to the constraints `3x+6y le 6, 4x+8y ge 16, x ge 0, y ge 0` is ………
02:29View SolutionThe corner points of the feasible region determined by some inequality are (0, 10), (5, 5), (15, 15), (0, 20). Let Z = px + qy, where `p, q gt 0` condition on p and q so that the maximum of Z occurs at both (15, 15) and (0, 20) is …………
02:05View SolutionObjective function of a LPP is …………
01:30View SolutionThe corner points of the feasible region are A(3, 3), B(20, 3), C(20, 10), D(18, 12) and E(12, 12). The maximum value of Z = 2x + 3y is ………….
02:36View SolutionCake-A requires 200g of flour and 25g of fat Cake-B requires 100 g of flour and 50 g of fat. Find the maximum number of cakes which can be made from 5 kg of flour and 1 kg of fat. The mathematical form of this LPP is ……..
03:45View SolutionThe shaded region in the given figure is a graph of ……………
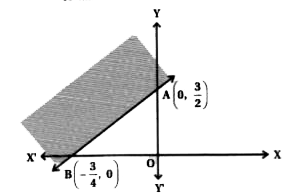 02:16View Solution
02:16View SolutionThe point at which the maximum value of Z = 3x + 2y subject to the constraints `x+2y le 2, x ge 0, y ge 0` is ……….
02:02View SolutionThe solution of linear programming problem, maximize `Z=3x_(1)+5x_(2)` subject to `3x_(1)+2x_(2) le 18, x_(1) le 4, x_(2) le 6, x_(1) ge 0, x_(2) ge 0` is ………..
05:05View SolutionThe maximum value of Z = x + 3y subject to the constraints `2x+y le 20, x+2y le 20, x ge 0, y ge 0` is ……….
04:13View SolutionThe solution set of the constraints `x+2y ge 11, 3x+4y le 30, 2x+5y le 30, x ge 0, y ge 0` includes the point.
03:17View SolutionThe feasible region of the inequality `x+y le 1" and "x-y le 1` lies in …….. quadrants.
03:08View SolutionThe following five inequalities form the feasible region. `2x-y le 8, x+y le 20, -x+y ge -10, x ge 0, y ge 0`. Redundant constraints is …
06:33View SolutionThe position of the points `O(0, 0)` and `P(2, -1)` is ………, in the region of the inequality `2y-3xlt5`.
02:17View SolutionThe constraints `x+y le 4, 3x+3y ge 18, x ge 0, y ge 0` defines on ………
02:16View SolutionThe solution set of the constraints `x+2y le 2000, x+y le 1500, y le 600" and "x ge 0` does not include the point ……….
05:12View SolutionOut of the following points, how many points are satisfied the inequality `2x-3y gt -5` ?
`(1, -1), (-1, 1), (1, -1), (-1, -1), (-2, 1), (2, -1),`
`(-1, 2)` and `(-2, -1)`.02:41View SolutionHow many points having integer co-ordinates are there in the feasible region of the inequality `3x+4y le 12, x ge 0" and "y ge 1`?
03:09View Solutionz = 30x - 30y + 1800 is a objective function. The corner points of the feasible region are (15, 0), (15, 15), (10, 20), (0, 20) and (0, 15). `z` has the minimum value at …….. point.
02:51View SolutionThe corner points of the bounded feasible region are (0, 1), (0, 7), (2, 7), (6, 3) (6, 0) (1, 0). For the objective function Z = 3x - y ……..
At which point, Z is minimum ?
(ii) At which point, Z is maximum ?
(iii) The maximum value of Z is .........
(iv) The minimum value of Z is ..........02:54View SolutionThe constraints `-x+y le 1, -x+3y le 9, x ge 0, y ge 0` defines on ………
03:16View SolutionThe solution set of the constraints `2x+3y le 6, 5x+3y le 15" and "x ge 0, y ge 0` does not include ……. point.
01:45View SolutionThe solution set of the constraints `2x+3y le 6, x+4y le 4" and "x ge 0, y ge 0` includes the point ………. As corner point.
03:53View SolutionThe region formed by the inequalities `2x+3y-5 le 0, 4x-3y+2 le 0" and " x ge 0` ………
04:14View SolutionA wholesale dealer wants to starts the business with Rs. 2,40,000. The cost price of a quintal wheat is Rs. 2000 and the cost price of a quintal rice is Rs. 3000. He has the space capacity for 200 quintals grain. The profit from the sale of one quintal wheat is Rs. 125 and that from one quintal rice is Rs. 200. If he has x quintal rice and y quintal wheat then the objective function for the maximum profit is ............
02:02View SolutionThe corner points of the bounded feasible region are (0, 0), (2, 0), (4, 2), (2, 4) and `(0, 10/3)`. Then for the objective function z = -x + 2y.
(i) Maximum value of z has at ……….
(ii) Minimum value of z has at …………
(iii) The maximum value of z is ...........
(iv) The minimum value of z is ............02:05View SolutionThe production of item A is x and the production of item B is y. If the corner points of the bounded feasible region are (1, 0), (2, 0), (0, 2) and (0, 1) then the maximum profit z = 2000x + 5000y is ……..
01:33View SolutionThe corner points of the bounded feasible region are (60, 0), (120, 0), (60, 40), (40, 20) and (20, 30). For the objective function z = 5x + 10y ………
(i) Maximum value of z.
(ii) Minimum value of z.
(iii) Maximum value of z has at ………..
(iv) Minimum value of z has at ...........02:58View SolutionThe minimum value of z = 2x + 4y subject to constraints `x+2y ge 10, 3x+y ge 10, x ge 0, y ge 0` is ………..
04:15View SolutionObjective function of a LPP is …………
01:30View SolutionThe vertices of the feasible region determined by some linear constraints are (0, 2), (1, 1), (3, 3), (1, 5). Let Z = px + qy where p, q gt 0. The condition on p and q so that the maximum of Z occurs at both the points (3, 3) and (1, 5) is ……..
01:35View SolutionIf the vertices of a feasible region are O(0, 0), A(10, 0), B(0, 20), C(15, 15), then minimum value of a objective function Z = 10x - 20y + 30 is ………
02:14View Solution
MULTIPLE CHOICE QUESTIONS questions and complete solutions for chapter LINEAR PROGRAMMING of KUMAR PRAKASHAN KENDRA MATHS (GUJRATI ENGLISH) of Class 12
