Complete Solutions to MATHEMATICS of chapter MHTCET 2009 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | MHTCET 2009 |
| MHTCET PREVIOUS YEAR PAPERS | MATHEMATICS |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
The approximate value of ` root(3)(28)` is
View SolutionIf `D_(30)` is the set of all divisors of 30 , x, `y in D_(30)`, we define `x+y=LCM(x,y),
x*y=GCD(x,y)
x'=(30)/(x)
and f(x,y,z)=(x+y)*(y'+z)`, then f(2,5,15) is equal toView SolutionLocus of the point of intersection of perpendicular tangents to the circle ` x^(2)+y^(2)=16` is
01:33View SolutionIf `A=[(3,2,4),(1,2,1),(3,2,6)]` and `A_(ij)` are the cofactors of `a_(ij)`, then `a_(11)A_(11)+a_(12)A_(12)+a_(13)A_(13)` is equal to
02:08View SolutionGiven ` vecP=3 hati+2hatj+5hatk, veca=hati+hatj, vecb=hatj+hatk, vecc=hati+hatk and vecP=xveca+yvecb+zvecc`, then x,y,z are respectively
02:35View SolutionA point on XOZ- plane divides tha join of (5,-3,-2) and (1,2,-2) at
04:30View SolutionIf the line `vec(OR)` makes angles ` theta_(1),theta_(2),theta_(3)` with the planes ` XOY, YOZ, ZOX` respectively , then ` cos^(2)theta_(1)+cos^(2)theta_(2)+cos^(2)theta_(3)` is equal to
02:26View SolutionJoint equation of pair of lines through `(3,-2)` and parallel to `x^2-4xy+3y^2=0` is
08:57View Solution`A=[(cos theta, -sin theta),(sin theta, cos theta)]` and `AB=BA=l`, then `B` is equal to
02:24View SolutionIf the equation given by `hxy+10x+6y+4=0` represents a pair of lines, then h is equal to
02:58View SolutionArea bounded between the curve `x^(2)=y` and the line y = 4x is
02:29View SolutionFind ` (dy)/(dx) , " if x " = 2 cos theta - cos 2 theta ` and
y = 2sin theta - sin 2 theta `.04:05View SolutionThe equation of motion of a particle moving along a straight line is `s=2t^(3)-9t^(2)+12t`, where the units of `s` and `t` are centrimetre and second. The acceleration of the particle will be zero after
01:28View SolutionWhich of the following is true ?
02:07View SolutionThe equation of the tangent to the curve `y=4xe^(x)` at `(-1,(-4)/e)` is
04:00View SolutionGiven ` P(A cup B)=0.6 , P(A capB)=0.2` , then probability of exactly one of the event occurs is
01:40View SolutionFrom a group of 8 boys and 3 girls, a committee of 5 members to be formed. Find the probability that 2 particular girls are included in the committee.
01:44View SolutionGiven P(A)=0.56 , P(B)=0.4 , `P(A cap B)=0.3`, then P(A'/B') is equal to
02:07View SolutionEquation of the plane passing through (-2,2,2) and (2,-2,-2) and perpendicular to the plane 9x-13y-3z=0 is
04:16View SolutionThe maximum vlaue of ` Z = 9x+13y ` subject to constraints ` 2x+3yle 18, 2x+y le 10, x ge 0, y ge 0 ` is
02:42View SolutionFind the function ` f(x_(1),x_(2),x_(3))` satisfying ` f(x^(1),x_(2),x_(3))`=1 at ` x_(1)=1,x_(2)=x_(3)=0` .
View SolutionGiven `f(x)=(ax+b)/(x+1), lim_(x to oo)f(x)=1 and lim_(x to 0)f(x)=2`, then f(-2) is
02:11View Solution`lim_(x to 1)(log ex)^(1//logx)` is equal to
03:29View Solution`int[sin(logx)+cos(logx)]dx`
04:00View Solution`int e^(x)""((x-1)/(x^(2)))dx` is equal to
01:45View Solution`int_(5)^(10) ""(1)/((x-1)(x-2))dx` is equal to
04:53View SolutionFor a certain function `u_(x)`, given that `u_(0)=3, u_(1)=12, u_(2)=81, u_(3)=200,u_(4)=100,u_(5)=8`, then ` Delta^(5)u_(x)` is equal to
View SolutionThe value of ` ((Delta^(2))/(E))x^(3)` at h=1 is
View SolutionGeneral solution of the differential equation ` (dy)/(dx)=(x+y+1)/(x+y-1)` is given by
03:43View SolutionThe order and degree of the differential equation ` (d^(2)y)/(dx^(2))=root(3)(1-((dy)/(dx))^(4))` are respectively
01:55View SolutionForm of the differential equation of all family of lines `y=mx+(4)/(m)` by eliminating the arbitrary constant m is
02:30View SolutionThe focal distance of a point P on the parabola `y^(2)=12x` if the ordinate of P is 6, is
01:33View SolutionFocus of hyperbola is `(+-3,0)` and equation of tangent is `2x+y-4=0`, find the equation of hyperbola is
03:56View SolutionIf ` 4x-3y+k=0` touches the ellipse ` 5x^(2)+9y^(2)=45` , then k is equal to
03:02View SolutionIf `m_1` and `m_2` are the slopes of tangents to `x^2+y^2= 4` from the point `(3,2)`, then `m_1- m_2` is equal to
05:04View SolutionIf `theta` is the angle between the lines `ax^2 +2hxy + by^2 =0` , then angle between `x^2 + 2xy sectheta + y^2 =0` is
02:07View Solutionfind the derivative of ` e^(x) + e^(y) = e^(x +y)`
02:25View SolutionIf ` xy = tan^(-1) (xy) + cot^(-1) (xy), " then" (dy)/(dx) ` is equal to
01:26View SolutionFind a polynomial f(x) of degree 2 where f(0)=8, f(1)=12, f(2)=18
02:28View SolutionTangent to the ellipse ` (x^(2))/(32)+(y^(2))/(18)=1` having slope `-(3)/(4)` meet the coordinates axes in A and B. Find the area of the `DeltaAOB` , where O is the origin .
04:05View Solution`int x log x dx` is equal to
02:06View Solution`int_(0)^(pi//2) ""(sin x - cos x)/( 1-sin x * cos x) dx ` is equal to
03:02View SolutionVolume of the parallelopiped having vertices at ` O-=(0,0,0) , A-=(2,-2,1),B-=(5,-4,4), and C= (1,-2,4)` is
02:08View SolutionIf ` 2veca+ 3vecb-5 vecc=vec0`, then ratio in which ` vecc` divides ` vec(AB)` is
02:59View Solution`~(~p to q)-=`
01:54View SolutionSimplify the following circuit and find the boolean polynomial .
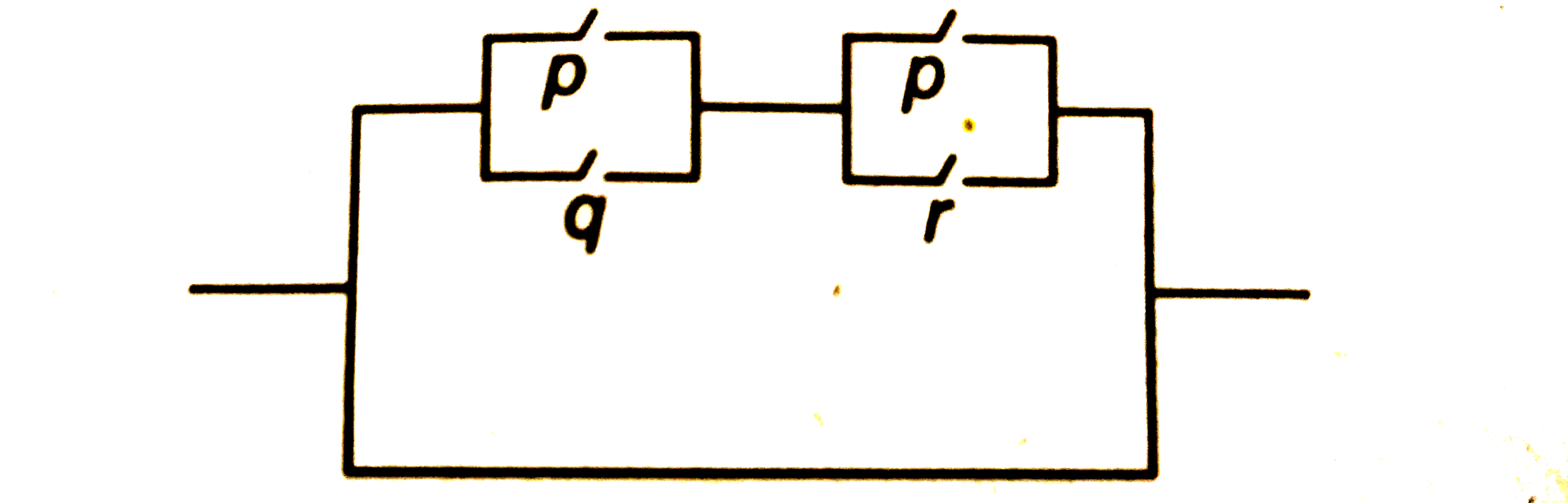 View Solution
View SolutionSimplify `(p vv q) ^^ (p vv ~q)`.
02:20View SolutionEquation of tangent to the parabola `y^(2)=16xx` at P(3,6) is
03:43View SolutionIf `lim_(x to 1)((e^(k)-1)sin kx)/(x^(2))=4`, then k is equal to
02:16View SolutionThe derivative of ` cos x^(3)` w.r.t. ` x^(3)` is
01:51View Solution
MATHEMATICS questions and complete solutions for chapter MHTCET 2009 of MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS of Class 12
