Complete Solutions to Multiple Choice Questions - LEVEL - II of chapter PERMUTATIONS AND COMBINATIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PERMUTATIONS AND COMBINATIONS |
| MODERN PUBLICATION | Multiple Choice Questions - LEVEL - II |
| Number Of Questions | 61 |
| Academic Year | 2023-24 |
If `""^(2n)C_3:""^nC_2=44:3` , then for which of the following values of r, the value of `""^nC_r` will be 15 ?
View SolutionIf x, y and r are positive integers , then : `""^xC_r+""^rC_(r-1)""^yC_1+^xC_(r-2)""^yC_2+..........+""^yC_r` =
View SolutionThe number of positive integers satisfying the inequality : `""^(n+1)C_(n-2)-""^(n+1)C_(n-1)le100` is :
View SolutionHow many words can be formed by taking four different letters of the word ' MATHEMATICS '' ?
View SolutionThe exponent of 3 in (100) ! Is :
View SolutionThe sum of the digits in unit place of all the numbers formed with the help of 3,4, 5,6 , taken all at a time , is :
View SolutionA five digit number divisible by 3 is to be formed using the numbers 0,1,2,3,4 and 5 without repetitions . The total number of ways in which this can be done is
View SolutionAn n - digit number is a positive number with exactly n digits. Nine hundred distinct n - digit numbers are to be formed using only the three digits 2,5 and 7 . The smallest value of n for which this is possible is :
View SolutionA parallelogram is cut by two sets of m lines parallel to the sides. The number of parallelograms thus formed is
View SolutionNumber of different arrangements which can be made out of the letters in the expansion of `A^2B^3C^4`, when written in full , is ,
View SolutionThe maximum number of points into which 4 circles and 4 st . Lines intersect is :
View SolutionA box contains two white balls, three black balls and four red balls. The number of ways in which three balls can be drawn from the box so that least one of the balls is black is :
View SolutionThere are n books having p copies of each . The number of ways in which a selection can be made from them is :
View SolutionTen different letters of an alphabet are given . Words with five letters are formed from these given letters. Then the number of wards which have at least one letter repeated is :
View Solutionm parallel lines in a plane are intersected by a family of n parallel lines. The total number of parallelograms so formed is :
View SolutionA student is allowed to select at most n books from a collection of (2n+1) books. If the total number of ways in which he can select the books is 63, then n is :
View SolutionThe sides AB, BC BC, CA of triangles ABC have 3 4 and 5 interior points respectively on them . The toal number of triangles that can be constructed by using these points as vertices is :
View SolutionHow many number between 5000 and 10,000 can be formed using the digits 1,2,3,4,5,6,7,8,9, each digit appearing not more than once in each number ?
View SolutionIf the letters of the word '' RACI II T '' are arranged in all possible ways and these words written out as in a dictionary , then the rank of the word ' RACHIT' is :
View SolutionLet A be the set of 4 - digit numbers abcd, where `a gt b gt c gt d ` , then n (A) equals :
View SolutionFour couples (husband and wife ) decide to form a committee of four members . The number of different committees that can be formed in which no couple finds a place is :
View SolutionNumber of odd proper divisors of `3^(p).6^(m).21^(n)` is :
View SolutionSum of divisors of `2^(5).3^(7).5^(3).7^(2)` is :
View SolutionEight chairs are numbered 1 to 8.Two women and three men wish to occupy one chair each . First the women choose the chair from the remaining chairs. The number of possible arrangements is :
View SolutionThere are four balls of different colours and four boxes of colours same as those of the balls. The number of ways in which the balls, one in each box, could be placed such that a ball does not got to box of its own colour own colour , is :
View SolutionIn a city no two person have identical set of teeth and there is no person without a tooth. Also no person has more than 32 teeth. If two disregard the shape and size of tooth and consider only the positioning of the teeth, then the maximum population of the city is :
View SolutionNumber of non - negative integral solutions of `a+b+c+d=n,n in N,` is :
View SolutionThe straight lines , `l_1,l_2 and l_3` are parallel and lie in the same plane . A total number of m points are taken on l : n points on `l_2` k points on `l_3` The maximum number of triangle formed with vertices at these points is :
View SolutionSeven different lecturers are to deliver lectures in seven periods of a class on a particular day X,Y and Z are three of the lecturers . The number of ways in which a routine for the day can be made such that X delivers his lecture before Y and Y before Z , is :
View SolutionA shop keeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase. The number of different ways of displaying the three varieties of perfumes in the showcase is :
View SolutionOn a railway there are 20 stations. The number of different tickets required in order that it may be possible to travel from every station to every station is :
View SolutionThe sum of all 4 digit number that can be formed by using the digits 2,4,6,8 (repetition of digits no allowed ) is :
View SolutionThe greatest common divisor of :
`""^(31)C_(16),^(31)C_(17),..........,^(31)C_(30)` is :
View SolutionMr. P has m children from his first wife and Mrs. Q has m + 1 children by her first husband. They marry and have children of their own . The whole family has 10 children . Assuming that two children of the same parents do not fight , the maximum number of fights that can take place among children is :
View SolutionFive distinct letters are to be transmitted through a communication channel. A total number of 15 blanks is to be inserted between the two letters with at least three between every two. The number of ways in which this can be done is :
View SolutionAll the letters of the word ' EAMCET '' are arranged in all possible ways. The number of such arrangements in which two vowels are adjacent to each other is :
View SolutionThe different letters of alphabet are given words with five letters are for med from these give letters . The number of word which have at least one latter repeated is :
View SolutionThe number of ways in which four particular persons A,B,C,D and six more persons can stand in a queue so that A always stands before B,B before C and C before D , is :
View SolutionThe total number of arrangements of the letters in the expansion `a^3b^2c^4` when written at full length is :
View SolutionThere are 2m horizontal lines equispaced at unit distance and 2n vertical lines equi- spaced at unit distance .
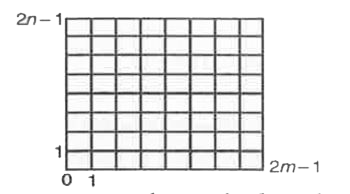
How many rectangles can be formed such that the length of their sides is odd ?
View SolutionAt an election , a voter may vote for any number of candidates , not greater than the number to be elected . There are 10 candidates and 4 are to be elected , If a voter votes for at least one candidates, then the number of ways in which he can vote is :
View SolutionThe letters of the word ' COCHIN ' are permuted and all permutations are arranged in an alphabetical order as in an english dictionary . The number of words that appear before the word ' COCHIN ' is :
View SolutionHow many different words can be formed by jumbling the letters of word MISSISSIPPI in which no two S are adjacent ?
View Solution.The number of ordered triplets of positive integers which are solutions of the equation` x +y+z=100`.
View SolutionThe number of ways in which 8 different flowers can be strung to form a garland so that 4 particular flowers are never separated is :
View SolutionThe sum of al the numbers that can be formed with the digits 2,3,4,5 , taken all at a time is :
View SolutionThe number of words that can be made by writing down the letters of the word '' CALCULATE '' such that each word starts and ends with a consonant is :
View SolutionIf the letters of the word KRISNA are arranged in all possible ways and these word 23 written out as in a dictionary, then the rank of the word KRISNA is
View SolutionThe number of ways in which four letters of the word '' MATHEMATICS '' can be arranged is given by :
View SolutionNumber of positive integral solutions of :
`x_1x_2x_3=30` is
View SolutionIf x,y,z are integers and `x ge 0 , y ge 1 , z ge 2 , x+y+z = 15` , then the number of values of the ordered triplet (x,y,z) is :
View SolutionSum of non - negative integral solutions of :
`x_1+x_2+.......+x_nle x` (where n is +ve integer ) is :
View SolutionThere are n straight lines in a plane, no two of which are parallel, and no three pass through the same point . Their points of intersection are joined . Then the number of fresh lines thus obtained is :
View SolutionIn the next World Cup there will be 12 teams , divided equally in two groups . Teams of each group will play a match against each other. From each group 3 top teams will qualify for the next round. In this round , each team will play against others once . Four top teams of this round will qualify for the semi - final round , where each team will play against the others once. Two top teams of this round will go to the final round , where they will play the best of three matches . The minimum number of matches in the next World Cup will be :
View SolutionPassengers are to travel by a double decked bus which can accommodate 13 in upper deck and 7 in the lower deck. The number of ways that they can be distributed if 5 refuse to sit in the upper desk and 8 refuse to sit in the lower deck is :
View SolutionThere are p,q,r points on three parallel lines `L_1,L_2 and L_3` respectively, all of which lie in one plane . The number of triangle which can be formed with vertices at these points is :
View SolutionIf 'P' is a prime number such that `p ge 23` and `n+ p! + 1 ` , then the number of primes in the list `n+ 1, n+2 , ….. , n+p-1` is
View SolutionIf a, b,c are three rational numbers in A.P. and a +b+c = 21 , then the possible number of values of the ordered triplet (a,b,c) is :
View SolutionIf `a_n=sum_(r=0)^n1/(""^nC_r)` , then `sum_(r=0)^nr/(""^nC_r)` equals :
View SolutionThe number of seven digit integers with sum of the digits equal to 10 and formed by using the digits 1,2, and 3 only , is :
View SolutionFrom 6 different novels and 3 different dictionaries , 4 novels 1 dictionary are to be selected and arranged in a row on a shelf so that the dictionary is always in middle. Then the number of such arrangement is :
View Solution
Multiple Choice Questions - LEVEL - II questions and complete solutions for chapter PERMUTATIONS AND COMBINATIONS of MODERN PUBLICATION MATHS (KANNADA ENGLISH) of Class 12