Complete Solutions to Multiple Choice Questions - LEVEL -I of chapter PERMUTATIONS AND COMBINATIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PERMUTATIONS AND COMBINATIONS |
| MODERN PUBLICATION | Multiple Choice Questions - LEVEL -I |
| Number Of Questions | 76 |
| Academic Year | 2023-24 |
If `""^nC_(12)=""^nC_8` , then n is equal to :
View SolutionThe number of possible outcomes when a coin is tossed 6 times is :
View SolutionThe number of different four digit numbers that can be formed with the digits 2,3,4,7 and using each digit only once is :
View SolutionThe sum of the digits in unit place of all the numbers formed with the help of 3,4,5 and 6, taken all at a time, is :
View SolutionTotal number of words formed by 2 vowels and 3 consonants taken from 4 vowels and 5 consonants, ie equal to :
View SolutionA five digit number divisible by 3 is to be formed using the numbers 0,1,2,3,4 and 5 without repetitions . The total number of ways this can be done is :
View SolutionEverybody in a room shakes hand with everybody else. The total number of handshakes is 66. The total number of persons in the room is :
View SolutionThe number of triangles that are formed by choosing the vertices from a set of 12 points , seven of which lie on the same line, is :
View SolutionThe straight lines , `l_1,l_2 and l_3` are parallel and lie in the same plane . A total number of m points are taken on l : n points on `l_2` k points on `l_3` The maximum number of triangle formed with vertices at these points is :
View SolutionThe number of parallelograms that can be formed from a set of four parallel lines intersecting another set of three parallel line intersecting another set of three parallel lines is:
View SolutionThe number of ways in which a team of eleven players can be selected from 22 players always including 2 of them and excluding 4of them is :
View SolutionThe number of 5- digit telephone number having at least one of their digits repeated is :
View SolutionThe number of ways in which we can choose a the committee from four men and six women so that the committee includes at least two men and exactly twice as many women as women as men is :
View SolutionThe total number 9- digit number Which have all different digits is :
View SolutionThe number of words that can be formed out of the letters of the word "ARTICLE" so that the vowels occupy even places is
View SolutionGiven 5 different green dyes , 4 different blue dyes and 3 different red dyes , the number of combinations of dyes which can be chosen taking at least one green and one blue dye is
View SolutionThere are four bus routes between And B , and three bus routes between B and C. A man can travel round - trip in number of ways by bus from A and C via B . If he does not want to use a bus route more than once ,in how many ways can he make round trip ?
View SolutionIn how many ways a committee consisting of 3 men and 2 women , can be chosen from 7 men and 5 women ?
View SolutionAll the latter of the word ' EAMCOT ' are arranged in different possible ways. The number of such arrangements in which no two vowels are adjacent to each other is :
View SolutionTen different letters of alphabet are given. Words with five letters are formed from these give letters.Then the number of words which have at least one letter repeated, is :
View SolutionThe number of signals, that can be sent by 5 flags of different colours , taking one or more at a time is :
View SolutionIn an examination there are three multiple choice questions and each questions has 4 choices . Number of ways in which a students can find to get all answers correct is :
View SolutionThe value of `""2^n[1.3.5 ..........(2n-3)(2n-1)]` is
View SolutionIf `""^nC_r-84,""^nC_(r-1)=36 and ""^nC_(r+1)=126` , then n equals :
View SolutionThe value of the expression :
`""^(47)C_4+sum_(j=1)^5""^(52-j)C_3` is equal to :
View SolutionThe smallest value of x satisfying the inequality `""^(10)C_(x-1)gt 2""^(10)C_x` is :
View SolutionThere are 4 letters and 4 directed envelopes . The number of ways in which every letter is put into a wrong envelope is :
View Solution66 games were played in a tournament where each player is placed against the rest. The number of players is :
View SolutionA lady gives dinner party to six guests . The number of ways in which they may be selected from among ten friends if two of the friends , will not attend the party together is :
View SolutionAll the letters of the Word '' AGAIN '' are permuted in all possible ways and the words so formed (With or Without meaning ) are written as in dictionary , then the 50th word is :
View SolutionThe number of different arrangements, which can be made from the letters of the word '' SERIES '' , taken all together is :
View SolutionThere are 15 points in a plane , no three of which are in a st line , except 6 , all of which are in a st. line The number of st lines , which can be drawn by joining them is :
View SolutionThere are `n( gt2)` points in each of two parallel lines Every point on one line is joined to every point on the other line by a line segment drawn within the lines . The number of points (between the lines) in which these segments intersect is :
View SolutionThere are 16 points in a plane no three of which are in a st , line except except 8 which are all in a st . Line The number of triangles that can be formed by joining them equals :
View SolutionNumber of ways in which 6 persons can be seated around a circular table so that two particular persons are never seated together , is equal to :
View SolutionA convex polygon has 44 diagonals. Find the number of sides.
View SolutionNumber of diagonals in a polygon of m sides is :
View SolutionNumber of divisors of the form `4n+2(n ge 0)` of integer 240 is :
View SolutionIn an examination there are three multiple choice questions and each questions has 4 choices . Number of ways in which a students can fail to get all answers correct is :
View SolutionThe number of different four - digit numbers that can be formed with the digits 2,3,4,5,7 , using each digit only once, is :
View SolutionThe number of ways in which 10 persons can go in two boats , so that there may be 5 on each boat, supposing that two particular persons will not go in the same boat, is :
View SolutionIn a cricket championship there are 36 matches . The number of teams if each plays one match with other , is :
View Solution12 persons are to be arranged to a round table . If two particular persons among them are not to be side by side, the total number of arrangements is :
View SolutionThe number of ways in which 6 boys and 6 girls can sit alternatively is :
View SolutionIf x is an integer between 0 and 21, then the minimum value of `x!(2l-x)!` is :
View SolutionThe total number of different combinations of letters which can be made from the letters of the word ' MISSISSIPPI '' is :
View SolutionA father with 8 children takes 3 at a time to a Zoological Garden as often as he can without taken same three children together more than once, The number of times :
Each child will go to the garden is :
View SolutionA father with 8 children takes 3 at a time to a Zoological Garden as often as he can without taken same three children together more than once, The number of times :
He will go to the garden is :
View SolutionThere are 10 lamps in a hall . Each one of them can be switched on independently . The number of ways in which the be illuminated is
View SolutionSeven women and seven men are to sit round a circular table such that there is a man on either side of every woman , the number of seating arrangements is :
View SolutionThe number of different words ending and beginning with a consonant which can be made out of the letters of the word '' EQUATION '' is :
View SolutionLet A be a set containing 10 distinct elements . Then the total number of distinct functions from A to A is :
View SolutionFrom 4 officers and 8 jawans, a committee of 6 is to be chosen to include exactly one officer. The number of such committees is :
View SolutionSix X ' s have to be placed in the squares of the figure given below such that each row contains at least one X . Th number of ways in which this can be done is :
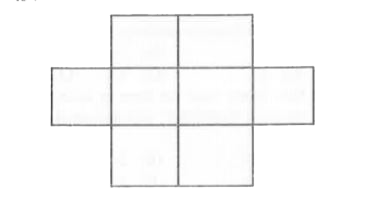
View SolutionLet A = { x : x is a prime number and `x lt 30` } The number of different rational numbers whose numerator and denominator belong to A is :
View SolutionThere are three coplanar lines. If any p points are taken on each of the lines , the maximum number of triangles with vertices at these points is :
View SolutionThe number of ways in which the letters of the word '' ARRANGE '' can be arranged such that both R do not come together is :
View SolutionIn a circus there are 10 cages for accommodating 10 animals. Out of these 4 cages are so small that 5 out of 10 animals cannot enter into them . In how many will it be possible to accommodate 10 animals in these 10 cages ?
View SolutionA is a set containing n elements. A subset P of A is chosen . The set A is reconstructed by replacing the elements of P.A subset Q of A is again chosen . The number of ways of choosing P and Q so that ` P cap Q = phi` is :
View SolutionHow many different nine - digit numbers can be formed from the number 223355888 by rearranging its digits so that the odd digit occupy even positions ?
View SolutionTwo straight lines intersect at a point O. Points `P_1 , P_2 , ...........,P_n` on the other . Ithe point O is not to be used, the number of triangles that can be drawn using these points as vertices is :
View SolutionThe number of proper divisors of 1800, which are also divisible by 10, is :
View SolutionLet `a_n=10^n/(n!)` for `nge1` Then `a_n` takes the greatest value when :
View SolutionHow many different nine - digit numbers can be formed from the number 223355888 by rearranging its digits so that the odd digit occupy even positions ?
View SolutionFor `2le rlen,{:((n),(r)):}+2({:(n),(r-1):})({:(n),(r-2):})=`
View SolutionThe number of arrangements of the letters of the word ' BANANA ' in which the two N's do not appear adjacently is :
View SolutionLet `T_n` denote the number of triangles which can be formed using the vertices of a regular polygon of n sides . If `T_(n+1)-T_n=21` , then n equals :
View SolutionThe number of ways in which 6 mean and 5 women can dine at a round table if no two women are to sit together is given by :
View SolutionA student is to answer 10 out of 13 questions in an examination such that he must choose at least 4 from the first five questions . The number of choices available to him is :
View SolutionIf `""^nC_r` denotes the number of combinations of n things , taken r at a time , then the expression : `""^nC_(r+1)+""^nC_(r-1)+2""^nC_r` equals :
View SolutionIf `""^(n-1)C_r=(k^2-3)""^nC_(r+1)`, then `kin` :
View SolutionIf `s_n=sum_(r=0)^n1/(""^nC_r)and t_n=sum_(r=0)^nr/(""^nC_r),` then `t_n/s_n` is equal to :
View SolutionThe number of ways of distributing 8 identical balls in 3 distinct boxes so that none of the boxes is empty is :
View SolutionHow many ways are there to arrange the letters in the word ' GARDEN ' with the vowels in alphabetical order ?
View SolutionThe value of `""^(50)C_4+sum_(r=1)^6""^(56-r)C_3` is
View SolutionIf the letters of the word ' SACHIN ' are arranged in all possible ways and these words are written out as in dictionary, then the word ' SACHIN ' appears at serial number :
View Solution
Multiple Choice Questions - LEVEL -I questions and complete solutions for chapter PERMUTATIONS AND COMBINATIONS of MODERN PUBLICATION MATHS (KANNADA ENGLISH) of Class 12