Complete Solutions to Revision Exercise of chapter LINEAR PROGRAMMING of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | LINEAR PROGRAMMING |
| MODERN PUBLICATION | Revision Exercise |
| Number Of Questions | 17 |
| Academic Year | 2023-24 |
Find the maximum and minimum values of `f: 2x + y` subject to the constraints :
`x + 3y ge 6, x - 3y le3, 3x + 4y le 24`,
`-3x + 2y le 6, 5x + y ge 5, x, y ge 0`.
View SolutionAn aeroplane can carry a maximum of 200 passengers. A profit of Rs 1000 is made on each executive class ticket and a profit of Rs 600 is made on each economy class ticket. The airline reserves at least 20 seats for executive class. However, at le
View SolutionA manufacturer of a line of patent medicines is preparing a production plane on medicines A and B. There are sufficient ingredients available to make 20,000 bottles of A and 40, 000 bottles of B but there are only 45, 000 bottles into which either of the medicines can be put. Further more, it takes 3 hours to prepare enough material to fill 100 bottles of A, it takes on hours to prepare enough material to fill 1000 bottles of B and there are 66 hours available for this operation. Te profit is Rs. 8 per bottle for A and Rs. 7 per bottle for B. Formulate this problem as a linear programming problem.
View SolutionA medical company has factories at two places, A and B.From these places, supply is made to each of its three agencies situated at P,Q, and R.The monthly requirements of the agencies are respectively 40,40 and 50 packets of the medicines, while the production capacity of the factories A and B, are 60 and 60 packets respectively.The transportation cost per packet from the factories to the agencies are given below:

How many packets from each factory be transported to each agency so that the cost of transportation is minimum? Also find the minimum cost.
View SolutionTwo godowns A and B have gram capacity of 100 quintals and 50 quintals respectively. They supply to 3 ration shops, D. E and F whose requirements are 60, 50 and 40 quintals respectively. The cost of transportation per quintal from the godowns to
View SolutionAn oil company has two depots A and B with capacities of 7000 L and 4000 L respectively. The company is to supply oil to three petrol pumps, D, E and F whose requirements are 4500L, 3000L and 3500L respectively. The distances (in km) between the
View SolutionA soft drinks firm has two bottling plants, one located at P and the other located at Q.Each plant produces three different soft drinks A, B and C.The capacities of two plants in number of bottles per day, are as follows:
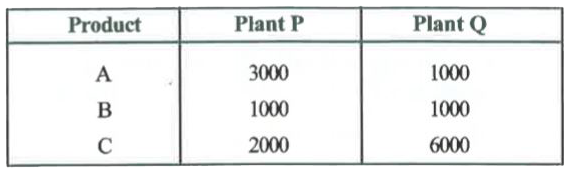
A market survey indicates that during the month of April, there will be a demand for 24,000 bottles of A, 16,000 bottles of B and 48,000 bottles of C.The cost of running the two plants P and Q are respectively ₹6,000 and ₹ 4,000 per day.Find graphically, the number of days for which either of the two plants P and Q should be run in the month of April so as to minimise production cost while still meeting the market demand.
View SolutionA toy company manufactures two types of dolls, A and B. Market tests and available resources have indicated that the combined production level should not exceed 1200 dolls per week and the demand for dolls of type B is at most half of that for do
View SolutionA dietician wishes to mix together two kinds of food `X` and `Y` in such a way that the mixture contains at least 10 units of vitamin A, 12 units of vitamin B and 8 units of vitamin C. The vitamin contents of 1 kg food is given below:

1 kg of food `X` costs Rs. 16 and 1 kg of food `Y` costs Rs. 20. Find the least cost of the mixture which will produce the required diet?
View Solution(Diet problem) A dietician has to develop a special diet using two foods `P` and `Q`. Each packet (containing `30g`) of food `P` contains 12 units of calcium, 4 units of iron, 6 units of cholesterol and 6 units of vitamin A. Each packet of the same quantity of food `Q` contains 3 units of calcium, 20 units of iron, 4 units of cholesterol and 3 units of vitamin A. The diet requires atleast 240 units of calciums atleast 460 units of iron and at most 300 units of cholesterol. How many packets of each food should be used to minimise the amount of vitamin A in the diet? What is the minimum amount of vitamin A? How many packets of each food should be used to maximise the amount of vitamin A in the diet? What is the maximum amount of vitamin A in the diet?
View SolutionA fruit grower can use two types of fertilizer in his garden, brand P and brand Q. The amounts (in kg) of nitrogen, phosphoric acid, potash, and chlorine m a bag of each brand are given m the table. Tests indicate that the garden needs at least 2
View SolutionA factory owner purchases two types of machines, `A` and `B` for his factory. The requirements and the limitations for the machines are as follows: Machine Area occupied Labour force Daily output (in units) `A` `1000\ m^2` `12\ m e n` `60` `B` `1200\ m^2` `8\ m e n` `40` He has maximum area of `9000\ m^2` available, and 72 skilled labourers who can operate both the machines. How many machines of each type should he buy to maximise the daily output?
View Solution(Manufacturing problem) A manufacturer has three machines I, II and III installed in his factory. Machines I and II are capable of being operated for at most 12 hours whereas machine HI must be operated for atleast 5 hours a day. She produces onl
View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the tune (in minutes) required for each toy on the machines is given below: Each machine is available for a maximum of 6 hours per day. If the profit
View SolutionA fanner mixes two brands P and Q of cattle feed. Brand P, costing Rs 250 per bag, contains 3 units of nutritional element A, 2.5 units of element B and 2 units of element C. Brand Q costing Rs 200 per bag contains 1.5 units of nutritional el
View SolutionA packet of plain biscuits costs ₹ 6 and that of choloate biscuits costs ₹ 9 . A house-wife has ₹ 72 and wants to buy at least three packets of plain biscuits and at least four of choloate biscuits.How many of each type should she buy so that she can have maximum number of packets?Make it as an LPP and solve it graphically.
View SolutionA manufacturer produces two products A and B. Both the products are processed on two different machines. The available capacity of first machine is 12 hours and that of second machine is 9 hours per day. Each unit of product A requires 3 hours on both machines and each unit of product B requires 2 hours on first machine and 1 hour on second machine. Each unit of product A is sold at Rs. 7 profit and that of B at a profit of Rs. 4. Find the production level per day for maximum profit graphically.
View Solution
Revision Exercise questions and complete solutions for chapter LINEAR PROGRAMMING of ARIHANT MATHS (HINGLISH) of Class 12
All Exercises from MODERN PUBLICATION Class 12 MATHS LINEAR PROGRAMMING
- (FAQ)
- Chapter Test
- Check your understanding
- EXERCISE
- Exercise - 12.1
- EXERCISE 12 (C ) (B.Fill in the Blanks)
- EXERCISE 12 (C ) (True/False Questions)
- EXERCISE 12 (C ) (Very Short Answer Type Questions)
- EXERCISE 12 C (Long Answer Type Questions )
- EXERCISE 12 C (MULTIPLE CHOICE QUESTIONS)
- EXERCISE 12(a) (Short Answer Type Questions)
- EXERCISE 12(b) (Long Answer Tyoe Questions(I)) (LATQ)
- EXERCISE 12(b) (Short Answer Type Questions)
- Exercise 12.2
- FAQs
- ILLUSTRATIVE EXAMPLES
- Miscellaneous Exercise
- Revision Exercise