Complete Solutions to Multiple Choice Questions (Level - III) (Questions From AIEEE/JEE Examination) of chapter HEAT AND THERMODYNAMICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | HEAT AND THERMODYNAMICS |
| MODERN PUBLICATION | Multiple Choice Questions (Level - III) (Questions From AIEEE/JEE Examination) |
| Number Of Questions | 17 |
| Academic Year | 2023-24 |
A thermally insulated vessel contains an ideal gas of molecular mass M and ratio of specific heats `gamma`. It is moving with speed v and it is suddenly brought to rest. Assuming no heat is lost to the surroundings, its temperature increases by:
View SolutionThree perfect gases at absolute temperatures `T_(1), T_(2) and T_(3)` are mixed. The masses of molecules are `m_(1), m_(2) and m_(3)` and the number of molecules are `n_(1), n_(2) and n_(3)` respectively. Assuming no less of energy, the final temperature of the mixture is:
View SolutionA Carnot engine operating between temperatures `T_(1) and T_(2)` has efficiency `(1)/(6)`. When `T_(2)` is lowered by 62 K its efficiency increase to `(1)/(3)`. Then `T_(1) and T_(2)` are, resectively :
View Solution100g of water is heated from `30^(@)" to "50^(@)C`. Ignoring the slight expansion of the water, the change in its internal energy is (specific heat of water is 4184 J/kg/K):
View SolutionThe specific heat capacity of a metal at low temperature (T) is given as :
`C_(p) (kJK^(-1)kg^(-1))=32 ((T)/(400))^(3)`
A 100 gram vessel of this metal is to be cooled from 20K to 4K by a special refrigerator operating at room temperature `(27^(@)C)`. The amount of work required to cool the vessel is:
View SolutionA metal rod of Young's modulus Y and coefficient of thermal expansion a is held at its two ends such that its length remains invariant. If its temperature is raised by `t^(@)C`, the linear stress developed in it is:
View SolutionAn aluminium sphere of 20 cm diameter is heated from `""^(@)C" to "100^(@)C`. Its volume changes by (given that coefficient of linear expansion for aluminium `alpha _(Al)= 23 xx 10^(6)//^(@)C)`.
View Solution5.6 litre of helium gas at STP is adiabatically compressed to 0.7 litre. Taking the initial temperature to be `T_(1)`, the work done in the process is
View SolutionSteel wire of length 'L' at `40^(@)C` is suspended from the ceiling and then a mass 'm' is hung from its free end. The wire is cooled dowa from `40^(@)C" to "30^(@)C` to regain its original length 'L'. The coefficient of linear thermal expansiou of the steel is `10^(-5)//^(@)C`, Young's modulus of steel is `10^(11)N//m^(2)` and radius of the wire is 1 mm. Assume that `L gt gt` diameter of the wire. Then the value of 'm' in kg is nearly
View SolutionHelium gas goes through a cycle ABCDA (consisting of two isochoric and two isobaric lines) as shown in figure. Eficiency of this cycle is nearly:
(Assume the gas to be close to ideal gas)
View SolutionA Carnot engine, whose efficiency is 40%, takes in heat from a source maintained at a temperature of 500 K. It is desired to have an engine of efficiency 60%. Then, the intake temperature for the same exhaust (sink) temperature must be :
View SolutionA wooden wheel of radius R is made of two semicircular parts (see figure). The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than `2pi R`. To fit the ring on the wheel, it is heated so that its temperature rises by `Delta T` and it just steps over the wheel. As it cools down to surrounding temperature, it presses the semicircular parts together. If the coefficient of linear expansion of the metal is `alpha`, and its Youngs modulus is Y, the force that one part of the wheel applies on the other part is:
View Solution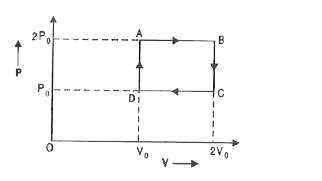
The above p-v diagram represents the thermodynamic cycle of an engine, operating with an ideal monoatomic gas. The amount of heat extracted from the source in a single cycle is:
View SolutionOne mole of diatomic ideal gas undergoes a cyclic process ABC as shown in figure. The process BC is adiabatic. The temperature at A, B and C are 400 K, 800 K and 600 K respectively. Choose the correct statement :
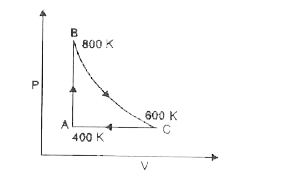
View SolutionThe pressure that has to be applied to the ends of a steel wire of length 10 cm to keep its length constant when its temperature is raised by `100^(@)C` is :
(For steel Young's modulus is `2 xx 10^(11) N m^(2)` and coefficient of themal expansion is `1.1 xx 10^(-5)K^(-1))`
View SolutionA solid of constant heat capacity `1J"/"^(@)C` is being heated by keeping it in contact with reservoirs in two ways : (i) Sequentially keeping in contact with 2 reservoirs such that each reservoir supplies same amount of heat.
(ii) Sequentially keeping in contact with 8 reservoirs such that each reservoir supplies same amount of heat.
In both the cases body is brought from initial temperature `100^(@)C` to final temperature `200^(@)C`. Entropy change of the body in the two cases respectively is:
View SolutionConsider an ideal gas confined in an isolated closed chamber. As the gas undergoes an adiabatic expansion, he average time of collision between molecules increases as `V^(q)`, where V is the volume of gas. The value of q is:
`(gamma =(C_(p))/(C_(v)))`
View Solution
Multiple Choice Questions (Level - III) (Questions From AIEEE/JEE Examination) questions and complete solutions for chapter HEAT AND THERMODYNAMICS of MODERN PUBLICATION PHYSICS (KANNADA ENGLISH) of Class 12