Complete Solutions to Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO of chapter HEAT TRANSFER of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | HEAT TRANSFER |
| PHYSICS GALAXY | Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO |
| Number Of Questions | 66 |
| Academic Year | 2023-24 |
A compound rod is formed of a steel core of diameter `1cm` and outer casing is of copper, whose outer diameter is `2cm`. The length of this compound rod is `2m` and one end is maintained as `100^(@)C` and the end is at `0^(@)C`. If the outer surface of the rod is thermally insulated, then heat current in the rod will be (Given thermal conductivity of steel `j=12cal//m//k//s`, thermal conductivity of copper `=92cal//m//k//s`
View SolutionA hollow glass sphere whose thicknes is `2mm` and external radius is `10cm` is filled with ice and placed in a bath containing boiling water at `100^(@)C`. Calculate the rate of which the ice melts. Thermal conductivity of glass `=1.1Js^(-1) m^(-1) K^(-1)` and `L` of ice `=336xx10^(3)Jkg^(-1)`.
View SolutionA steel boiler whose thickness is 3 cm is placed on a plate of area `1m^(2)`. The temperature of the plate is `300^(@)C` and that of the boiling water in the boiler is `100^(@)C`. How much water will evaporate per minute? (Conductivity of steel `= 63.0Js^(-1) m^(-1) K^(-1)` and sp. latent heat of vaporisation of water `=2251.2 xx 10^(3) Jkg^(-1).)`
View SolutionA certain double plane window consists of two glass sheets each `80 cm xx 80 cm xx 0.30 cm,` separated by `0.3 cm` stagnant air space between them (see figure-4.49). The indoor surface temperature is `20^(@)C,` while the outdoor surface temperature is `0^(@)C.` Find:
(a) the temperature of the surface of the sheets in contact.with the stagnant air
(b) the power transmitted from the inside to the outside. Given that `K_(glass)=2.0xx10^(-3) cal s^(-1) cm^(-1)""^(@)C` ,
`K_(air)=2.0xx10^(-4) cal s^(-1) cm^(-1)""^(@)C^(-1)`

View SolutionWater is being boiled in a flat bottomed kettle placed on a stove The area of the bottom is `300cm^(2)` and the thickness is `2mm` If the amount pf steam produced is `1gm min^(-1)` then the difference of the temperature between the inner and the outer surface of the bottom is (thermal conductivity of the matrial of the kettle `0.5calcm^(-1)C^(-1)` latent heat of the steam is equal to `540calg^(-1))` .
View SolutionA spherical ball of surface area `2xx10^(-3)m^(2)` is suspended in a room at temperature 330 K. If the temperature of the ball is `200^(@)C,` find the net rate of loss of heat from the ball if it behaves like a black body.
View SolutionSome water is placed in a container made ofa material of poor thermal conductivity. Temperature of water in it is 520 K. The total wall area of the container is `8000 cm^(2).` The surrounding temperature is 300 K. Find the rate at which heat current will flow from atmosphere to water.
View SolutionHeat is conducted through a slab composed of paralel layers of two different materials of conductivities `134.4` SI units and 58.8 SI units and of thickness `3.6cm` and `4.2cm` respectively. The temperature of the outer faces of the compound slab are `96^(@)C` and `8^(@)C`. Find (i) the temperature of the interface, (ii) temperature gradient in each section of the slab.
View SolutionTwo bodies each of mass m, having specific heats s, are connected by ametal rod of negligible heat capacity of length l, area of cross section A and thermal conductivity k. Initially both bodies are at different temperatures, find the time taken for the temperature difference between the two bodies to become half of the. initial value.
View SolutionA 2 m long wire of resistance `4 Omega` and diameter 0.64 mm is coated with plastic insulation of thickness 0.66 mm. A current of 5A flows through the wire. Find the temperature difference across the insulation in the steady state. Thermal conductivity of plastic is `0.16 xx (10^-2) cal//s cm.^@C`.
View SolutionOne end of a steel rod of length 1 m and area of cross section `4 xx 10^(-6)m^(2)` is put in boiling water and the other end is kept in an ice bath at `0^(@)C.` If thermal conductivity of steel is `46 W//m^(@)C,` find the amount of ice melting per second if heat flow only by conduction. Given that the latent heat of fusion of ice is `3.36 xx 10^(5)J//kg.`
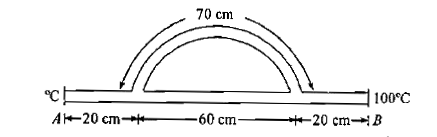
View SolutionFigure-4.50 shows a thermal network of two metal rods of same cross section area. If the heat current from the ends A and B is 130 W, find the heat current through the curved metal rod.
View SolutionThe heat generated by radioactivity within the earth is conducted outward through the oceans. Assuming the average temperature gradient within the solid earth beneath the ocean to be `0.07 ^(@)C^(-1)` and the average thermal conductivity `0.2 cal m^(-1) s^(-1) ^(@)C^(-1)`, determine the rate of heat transfer per square metre. Radius of the earth = 6400 km.- further, determine the quantity of heat transferred through the earth's surface each day
View SolutionTwo rods whose lengths are `l_1` and `l_2` and heat conductivity coefficient `x_1` and `x_2` are placed end to end. Find the heat conductivity coefficient of a uniform rod of length `l_1 + l_2` whose conductivity is the same as that of the system of these two rods. The lateral surfaces of the rods are assumed to be thermally insulated.
View SolutionA solid copper sphere (density `=8900kgm^(-3)` and specific heat `C=390Jkg^(-1)K^(-1)`) of radius `r=10cm` is at an initial temperature `T_(1)=200K`. It is then suspended inside an chamber whose walls are at almost `OK`. Calculate the time required for the temperature of the sphere to drop to `T_(2)=100 K`, `sigma=5.67xx10^(-8)Wm^(-2)K^(-4)`.
View SolutionA calorimeter of mass 100 g contains `100 cm^(3)` of water at `70^(@)C.` It cools down to `30^(@)C` in 12 minutes. When the same volume of glycerine is used in the same calorimeter, it takes 8 minutes to cool down through the same temperature range. Find the specific heat of glycerine of specific heat of the calorimeter is `0.1 calg^(-1)^(@)C^(-1)` and specific gravity of glycerine `=1.27.`
View SolutionA vertical brick duct (tube) is filled with cast iron. The lower end of the duct is maintained at a temperature `T_1` which is greater than the melting point `T_m` of cast iron and the upper end at a temperature `T_2` which is less than the temperature of the melting point of cast iron. It is given that the conductivity of liquid cast iron is equal to k times the conductivity of solid cast iron. Determine the fraction of the duct filled with molten metal.
View SolutionThe gas between two long coaxial cylindrical surfaces is filled with a homogeneous isotropic substance. The radii of the surfaces are `r_(1) = 5.00 cm` and `r_(2) = 7.00 cm`. In the steady state the temperatures of the inner and outer surfaces are `T_(1) =290 K and =320 K` respectively. Find the temperature of a coaxial surface of radius r.
View SolutionThe tungsten filament of an electric lamp has a length `l = 0.25m` and diameter `d = 0.04 mm.` The power rating is `P = 100 W.` Assuming the radiation from the filament to be `eta=80%` of that of a black body radiator at the same temperature, estimate the temperature of the filament. Stefan constant `= 5.7 xx 10^(-8)W//m^(2)K^(4).`
View SolutionThere are two concentric metallic shells of negligible thickness of radii 5 cm and 20 cm. The region between the two shells is filled with a medium of thermal conductivity k. The temperature of inner and outer sphere is maintained at `5^(@)C` and `10^(@)C` respectively. If the heat current flowing from inner to outer sphere is 100 W, find the value of k.
View SolutionTwo bodies A and B ahave thermal emissivities of 0.01 and 0.81 respectively. The outer surface areas of the two bodies are the same. The two bodies emit total radiant power of the same rate. The wavelength `lambda_B` corresponding to maximum spectral radiancy in the radiation from B shifted from the wavelength corresponding to maximum spectral radiancy in the radiation from A, by `1.00mum.` If the temperature of A is 5820K:
View SolutionA thin brass rectangular sheet of sides 15.0 and 12.0 cm is heated in a furnace to `600^@` C and taken out. How much electric power is needed to maintain the sheet at this temperature, given that its emissivity is 0.250?
Neglect heat loss due to convection (Stefan-Boltzmann constant, `sigma=5.67xx10^-8 W//m^(2)-K^(4)`).
View SolutionA body which has a surface area `5.0 cm^(2)` and a temperature of `727^(@)C` radiater 300 J energy each minute. What is its emissivity? Stefan's Boltzmann's constat is `5.76 xx 10^(-8) Wm^(-2)K^(-4)`.
View SolutionOver what distance must there be heat flow by conduction from the blood capillaries beneath the skin to the surface if the temperature difference is `0.50^(@)C` ? Assume `200 W` must be transferred through the whole body's surface area of `1.5 m^(2)` Given that thermal conductivity of blood cells is `0.2W//mK.`
View SolutionA room at `20^@C` is heated by a heater of resistence 20 ohm connected to 200 VV mains. The temperature is uniform throughout the room and the heati s transmitted through a glass window of area `1m^2` and thickness 0.2 cm. Calculate the temperature outside. Thermal conductivity of glass is `0.2 cal//mC^@` s and mechanical equivalent of heat is `4.2 J//cal`.
View SolutionAssume that a planet radiates heat at a rate proportional to the fourth power of its surface temperature T and that the temperature of the planet is such that this loss is exactly compensated by the heat gained from the sun. Show that other things remaining the same, a planet's surface temperature will vary inversely as the square root of its distance from the sun.
View SolutionThe atmospheric temperature above a lake is below `0^(@)C` and constant. It is found that a 2 cm layer of ice is formed in four days. In how many days will the thickness increase to 3 cm?
View SolutionA hollow cube of metal has sides measuring `0.8cm` (internal) and thickenes `0.5cm`. It is filled with ice `0^(@)C` and immersed in boiling water at `100^(@)C`. How many kg of ice will melt in one minute? Thermal conductivity of metal `=252Jm^(-1)s^(-1)K^(-1)` and latent heat capacity of ic e`=336xx10^(3)Jkg^(-1)`.
View SolutionThe walls of a closed cubical box of edge 50cm are mdade of a material of thickness 1mm and thermal conductivity `4xx10^(-4)` cal `s^(-1)cm^(-1).^(@)C^(-1)` the interior of the box maintained at `100^(@)C` above the outside temperature by a heater placed inside the box and connected acros a 400 V d.c. source. Calculate the resistance of the heater.
View SolutionA 300 W lamp loses all its energy by emission of radiation from the surface of its filament. If the area of surface of filament is `2.4cm^(2)` and is of emissivity `0.4,` estimate its temperature. Given that `sigma = 1.36 xx 10^(-12) cal cm^(-2) s^(-1) K^(-4) J=4.2 J cal^(-1)`. Neglect the absorption from the surroundings.
View SolutionA blackened solid copper sphere of radius 2 cm is placed in an evacuated enclosure whose walls are kept at `100^(@)C`. At what rate must energy be supplied to the sphere to keep its temperature constant at `127^(@)C` ? Stefan constant `= 5.67xx 10^(-8)Jm^(-2)K^(-4).`
View SolutionTwo identical solid bodies one of aluminium and other of copper are heated to the same temperature and are put in same surrounding. If the emissivity of the aluminium body is 4 times that of copper body, find the ratio of the thermal power radiated by the two bodies. If specific heat of aluminum is `900//kg^(@)C` and that of copper is `390 J//kg^(@)C` and density of copper is 3.4 times that of aluminum, find the ratio of rate of cooling of the two spheres.
View SolutionA solid copper sphere (density rho and specific heat c) of radius r at an initial temperature `200K` is suspended inside a chamber whose walls are at almost `0K.` The time required for the temperature of the sphere to drop to 100K is …….
View SolutionThe thermal powered density u is generated uniformly inside a uniform sphere of radius R and thermal conductivity Find the temperature distribution in the sphere when the steady state temperature at the surface is `T_(0)`.
View SolutionA rof of length `l` with thermally insulated lateral surface consists of material whose heat conductivity coefficient varies with temperature as `x = alpha//T`, where `alpha` is a constant. The ends of the rod are kept at temperatures `T_1` and `T_2`. Find the function `T (x)`, where `x` is the distance from the end whose temperature is `T_1`, and the heat flow density.
View SolutionA hot water radiator at 310 K temperature radiates thermal radiation like a black body. Its total surface area is `1.6m^(2).` Find the thermal power radiated by it.
View SolutionA flat bottomed metal tank of water is dragged along a horizontal floor at the rate of `20 ms^(-1)`. The tank is of mass 20kg and contains 1000 kg of water and all the heat produced in the dragging is conducted to the water through the bottom plate of the tank. If the bottom plate has an effective area of conduction 1 m^2 and a thickness 5 cm and the temperature of the water in the tank remains constant at `50^(@)C`, calculate the temperature of the bottom surface of the tank, given the coefficient of friction between the tank and the floor is 0.343 and K for the material of the tank is `25 cal m^(-1) s^(-1) K^(-1).`
View SolutionTwo solid spheres, one of aluminium and the other of copper, of twice the radius are heated to the same temperature and are allowed to cool under the identical conditions. Given that specific heat of aluminium is `900 J//kg` K and that ofcopper is `390 J//kg` K. Specific gravity of aluminium and copper are `2.7` and `8.9` respectively.
initial rates of fall of temperature, and
the initial rates of loss of heat
View SolutionEstimate the rate that heat can be conducted from the interior of the body to the surface. Assume that the thickness of tissue is 4.0 cm, that the skin is at `34^(@)C` and the interior at `37^(@)C,` and that the surface area is `1.5m^(2).` Compare this to the measured value of about `225 W` that must be dissipated by a person working lightly. This clearly shows the necessity of conveclive cooling by the blood. Given that the thermal conductivity of the blood cells is `0.2 W//mK`
View SolutionThe temperature of the filament of `100-`watt lamp is `4000^(@)C` in the steady state and the radius of the glass bulb is 4 cm and the thickness of the wall is `0.4 mm.` Assuming that there is no convection, calculate the thermal conductivity of glass. The temperature of the outside air is `27^(@)C.`
View SolutionA thin pipe having outside diameter of 3 cm is to be covered with two layers of insulation each having thickness of 2.5 cm. The thermal conductivity of one material is five times that of the other. Assuming that the inner and outer surface temperatures of the composite wall are fixed, find the percentage reduction in heat transfer when the better insulating material is next to the thin pipe than when it is outside.
View SolutionA spherical metal ball of radius 1 cm is suspended in a room at 300 K temperature. Inside the sphere there is a battery operated heater which maintains the temperature of the ball at 1000 K. Find the power of the heater if emissivity of the metal ball is 0.3.
View SolutionA block of copper of radius `r = 5.0 cm` is coated black on its outer surface. How much time is required for block to cool down from 1000 K to 300 K? Density of copper, `p = 9000 kg//m^(3)` and its specific heat, `c = 4 kJ//kg. K.`
View SolutionOne end of a rod of length 20 cm is maintained at 800 K. The temperature of the other end of the rod is 750 K in steady state and this end is blackened to radiate thermal radiations like a black body. If temperature of the surrounding is 300 K, find the thermal conductivity of the rod. Assume no energy loss takes place through the lateral surface of the rod during conduction through its length.
View SolutionIn a pitcher 10 kg water is contained.Total surlace area of pitcher walls is `2 xx 10^(-2) m^(2)` and its wall thickness is `10^(-3) m.` If surrounding temperature is `42^(@)C,` find the temperature of water in the pitcher when it attains a steady value. Given that in steady state 0.1 gm water gets evaporated per second from the outer surface of pitcher through its porous walls. The thermal conductivity of the walls of pitcher is `0.8 W//m^(@)C` and latent heat of vaporization of water is `2.27 x 10^(6) J//kg.`
View SolutionA uniform copper rod 50 cm long is insulated on the sides, and has its ends exposed to ice and steam, respectively. If there is a layer of water 1 mm thick at each end, calculate the temperature gradient in the bar. The thermal conductivity of copper is `436 W m^(-1) K^(-1)` and that of water is `0.436 Wm^(-1) K^(-1)`.
View SolutionFind the temperature distribution in a substance palced between two parallel plates kept at temperatures `T_1` and `T_2`. The plate separation is equal to `l`, the heat conductivity coefficient of the substance `x overline prop V overline T`.
View SolutionFour spheres A, B, C and D of different metals but all same radius are kept at same temperature. The ratio all their densities and specific heats are `2:3:5:1` and `3:6:2:4`. Which sphere will show the fastest rate all cooling (initially) (assume black body radiation for all of them)
View SolutionA closed cubical box made of perfectly insulating material has walls of thickness 8 cm and the only way for heat to enter or leave the box is through two solid, cylindrical, metallic plugs, each of cross-sectional area `12 cm^(2)` and length 8 cm in opposite walls of the box. The outer surface of one plug is kept at `100^(@)C` while the outer surface of the other plug is maintained at `4^(@)C.` The thermal conductivity of the plug is `50 cal s^(-1) m^(-1) K^(-1).` A source of energy generating `36 cal s^(-1)` is enclosed inside the box. Find the equilibrium temperature of the inner surface of the box, assuming that it is the same at all points on the inner surface.
View SolutionThe solar energy received by the Earth persquare metre per minute is `8.315 xx 10^(4)Jm^(-2)min^(-1)`. If the radius of the Sun is` 7.5 xx 10^(5)` km and the distance of the Earth from the Sun is `1.5 xx10^(8)` km, calculate the surface temperature of Sun. Assume the Sun as a perfect black body. Given that Stefen constanta ` sigma = 5.7 xx 10^(-8)Wm^(-2)K^(-4).`
View SolutionTwo solid spheres are heated to the same temperature allowed to cool under identical conditions Compare (i) initial rates of fall of temperatre, and (ii) initial rates of loss of heat Assume that all the surfaces have the same emissivity and ratios of their radii specific heats and densities are respectively `1: alpha 1: beta, 1 : gamma` .
View SolutionA metal block with a heater in it is placed in a room at temperature 293 K. When the heater is switched on it is observed that the temperature of the block rises at the rate of `2^(@)C//s` and when its temperature rises to `30^(@)C,` it is switched off. Just after when heater is switched off, it is observed that the block cools at `0.2^(@)C//s.` If Newton's law of cooling is assumed to be valid, find the power of the heater. Also find the thermal power radiated by the block when it was at `30^(@)C` and at `25^(@)C.` Given that the heat capacityof the block is `80 J//^(@)C.`
View SolutionIn a cylindrical metallic vessel some water is taken and is put on a burner. The bottom surface area of the vessel is `2.5 xx 10^(-3)m^(2)` and thickness `10^(-3)m.` The thermal conductivity of the metal of vessel is `50 W//m^(@)C.` When water boils, it is observed that 100 gm water is vaporized per minute. Calculate the temperature of the bottom surface of the vessel. Given that the latent heat of vaporization of water is `2.26 xx 10^(6)J//kg.`
View SolutionA system `S` receives heat continuously from an electric heater of power `10 W`. The temperature of `S` becomes constant at `50^(@)C` when the surrounding temperature is `20^(@)C`. After the heater is switched off, `S` cools from `35.1^(@)C` to `34.9^(@)C` in `1 minute`. the heat capacity of `S` is
View SolutionIn winters ice forms on the surface of a lake. Due to abnormal expansion of water the temperature of the water at the bottom of the lake remains constant at `4^(@)C` and we assume that the amount of heat required to maintain this temperamre of the bottom layer of water may come from the bed of the lake. If surrounding temperature is - `10^(@)C` Prove that ice formed from the surface of the lake attains a maximum thickness. Find the maximum depth from surface upto which ice is formed if the depth of lake is I m. Given that thermal conductivity of ice is `0.5 W//m^(@) C.`
View SolutionTwo spheres of the same material have radii `1m ` and `4m` and temperatures `4000K ` and `2000K ` respectively. The energy radiated per second by the first sphere is greater than that by the second.
View SolutionThe temperature of the tungsten filament of a 40 watt lamp is `1655^(@)C.` The effective surface area of the filament is `0.85 cm^(2).` Assuming that the energy radiated from the filament is 60% of that of a black body radiator at the same temperature, find the value of Stefan's constant.
View SolutionA cubical tank of water of volume `1m^(3)` is kept at a constant temperature of `65^(@)C` by 1 KW heater. At time `t = 0,` the heater is switched off. Find the time taken by the tank to cool down to `50^(@)C,` given the temperature of the room is steady at `15^(@)C.` Density of water `= 10^(3) kg m^(-3)` and specific heat of water `= 1.0 cal g^(-1)^(@)C^(-1).` (Do not assume average temperature during cooling). Take `1 KW= 240 cal s^(-1).`
View SolutionFind the temperature distribution in the space between two coaxial cylinders of radii `R_1` and `R_2` filled with a uniform heat conducting substance if the temperatures of the cylinders are constant and are equal to `T_1` and `T_2` respectively.
View SolutionA cube `a = 3.0` cm on each side radiates energy at the rate of `P = 20 J//s` when its temperature is `727^(@)C` and surrounding temperature `27^(@)C.` Determine its emissivity.
View SolutionSolve the foregoing problem for the case of two concentric spheres of radii `R_1` and `R_2` and temperatures `T_1` and `T_2`.
View SolutionA long tungsten heater wire is rated at `3 kWm^(-1)` and is `5.0 xx 10^(-4) m` in diameter. It is embedded along the axis of a ceramic cylinder of diameter `0.12 m.` When operating at the rated power, the wire is at `1500^(@)C`, the outside of the cylinder is at `20^(@)C.` Find the thermal conductivity of the ceramic.
View SolutionA constant electric current flows along a uniform wire with cross-sectional radius `R` and heat conductivity coefficient `x`. A unit volume of the wire generates a thermal power `omega`. Find the temperature distribution across the wire provided the steady-state temperature at the wire surface is equal to `T_0`.
View SolutionAn iron boiler with walls 1.25 cm thick contains water at atmospheric pressure. The heated surface is `2.5 m^(2)` in area and the temperature of the underside is `120^(@)C.` Thermal conductivity of iron is `20 cal s^(-1) m^(-1) K^(-1)` and the latent heat of evaporation of water `536 xx10^(3) cal kg^(-1) `. Find the mass of water evaporated per hour.
View SolutionA thin wire of length `l=50cm` and area of cross-section `S=3xx10^(-4)m^(2)` is heated to `727^(@)C`. How much electri power `P` is needed to maintain the wire at this temperature ? Assume that emmissivity of the wire's surface is `e=0.25`.
View SolutionIn the figure-4.51 shown here, S is a source of heat supplying energy at a constant rate `75 J s^(-1)` and S' is a sink maintained at `10^(@)C`. The two conductors joining S to S' are each 20 cm long, `1 cm^(2)` in cross-section and of thermal conductivity `385 W m^(-1) K^(-1) s^(-1).` Calculate the temperature of the point S.

View Solution
Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO questions and complete solutions for chapter HEAT TRANSFER of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 12
All Exercises from PHYSICS GALAXY Class 12 PHYSICS HEAT TRANSFER
- Advance MCQs with One or More Option Correct
- Conceptual MCQs Single Option Correct
- Discussion Question
- Illustrative Example 4.1
- Illustrative Example 4.10
- Illustrative Example 4.11
- Illustrative Example 4.12
- Illustrative Example 4.13
- Illustrative Example 4.14
- Illustrative Example 4.15
- Illustrative Example 4.16
- Illustrative Example 4.17
- Illustrative Example 4.18
- Illustrative Example 4.19
- Illustrative Example 4.2
- Illustrative Example 4.20
- Illustrative Example 4.21
- Illustrative Example 4.22
- Illustrative Example 4.23
- Illustrative Example 4.24
- Illustrative Example 4.25
- Illustrative Example 4.26
- Illustrative Example 4.27
- Illustrative Example 4.28
- Illustrative Example 4.29
- Illustrative Example 4.3
- Illustrative Example 4.4
- Illustrative Example 4.5
- Illustrative Example 4.6
- Illustrative Example 4.7
- Illustrative Example 4.8
- Illustrative Example 4.9
- Numerical MCQs Single Options Correct
- Practice Exercise 4.1
- Practice Exercise 4.2
- Practice Exercise 4.3
- Practice Exercise 4.4
- Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO