Complete Solutions to Sample problem of chapter MAGNETIC FORCE of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | MAGNETIC FORCE |
| RESNICK AND HALLIDAY | Sample problem |
| Number Of Questions | 14 |
| Academic Year | 2023-24 |
A proton of energy `200 MeV` enters the magnetic field of `5 T`. If direction of field is from south to north and motion is upward, the force acting on it will be
View SolutionAn electron moving with a kinetic energy of `1.5 xx 10^(3)` eV enters in a region of uniform magnetic field of induction `4 xx 10^(-3) Wb//m^(2)` at right angles to the direction of motion of the electron . The radius of circular path of the electron in the magnetic field is
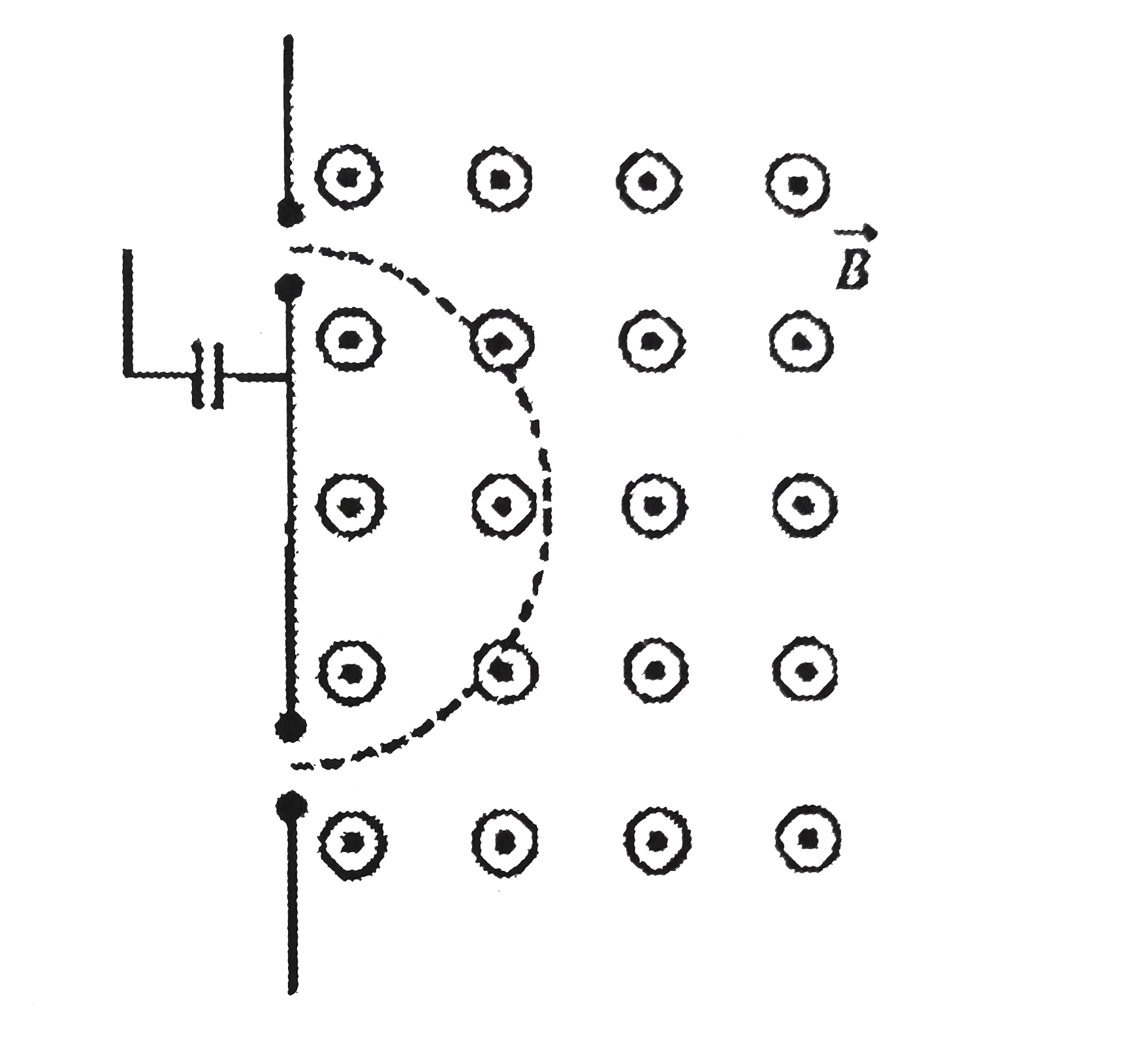
View SolutionA mass spectrometer is a device which selelct particle of equal mass. An ion with an electric charge `qgt0`. Starts at rest from s ource S and is accelerated through a potential difference V. it passes thorugh a hole into a region of constant magnetic field `vecB` perpendicular to the plane of the paper as shown in the figure. The particle is deflected by the magnetic field and emerges thorugh the bottom hole at a distance d from the top hole. The mass of the particle is:
View SolutionA particle of mass `m=1.6xx10^-27` kg and charge `q=1.6xx10^-19C` enters a region of uniform magnetic field of stregth `1 T` along the direction shown in figure. The speed of the particle is `10^7 m//s`

a. The magnetic field is directed along the inward normal to the plane of the paper. The particle leaves the region of the fiedl at the point `F`. Find the distasnce `EF` and the angle theta.
b. If the direction of the field is along the outward normal to the plane of the paper find the time spent by the particle in the regin of the magnetic field after entering it at `E`.
View SolutionA cyclotron is operated at an oscillator frequency of 12 MHz and has a dee radius R 50 cm. What is the magnitude of the magnetic field needed for a proton to be accelerated in the cyclotron?
View SolutionA cyclotron is operated at an oscillator frequency of 12 MHz and has a dee radius R 50 cm. What is the magnitude of the magnetic field needed for a proton to be accelerated in the cyclotron?
View SolutionA particle of mass m and charge q is moving in a region where uniform, constant electric and mangetic fields `vec E and vec B` are present. `vec E and vec B` are parallel to each other. At time `t=0,` the velocity `vec v_0` of the particle is perpendicular to `vec E` (Assume that its speed is always `lt lt c`, the speed of light in vacuum). Find the velocity `vec v` of the particle at time `t`. You must express your answer in terms of `t, q, m,` the vector `vec v_0, vec E` and `vec B` and their magnitudes `vec v_0, vec E` and `vec B`.
View SolutionA positively charged disk is rotated clockwise as shown in Fig. The direction of hte magnetic field at a point A in the plane of the disk is
View SolutionA straight horizontal copper wire carries a current i = 30 A. The linear mass density of the wire is 45 g/m. What is the magnitude of the magnetic field needed to balance its weight?
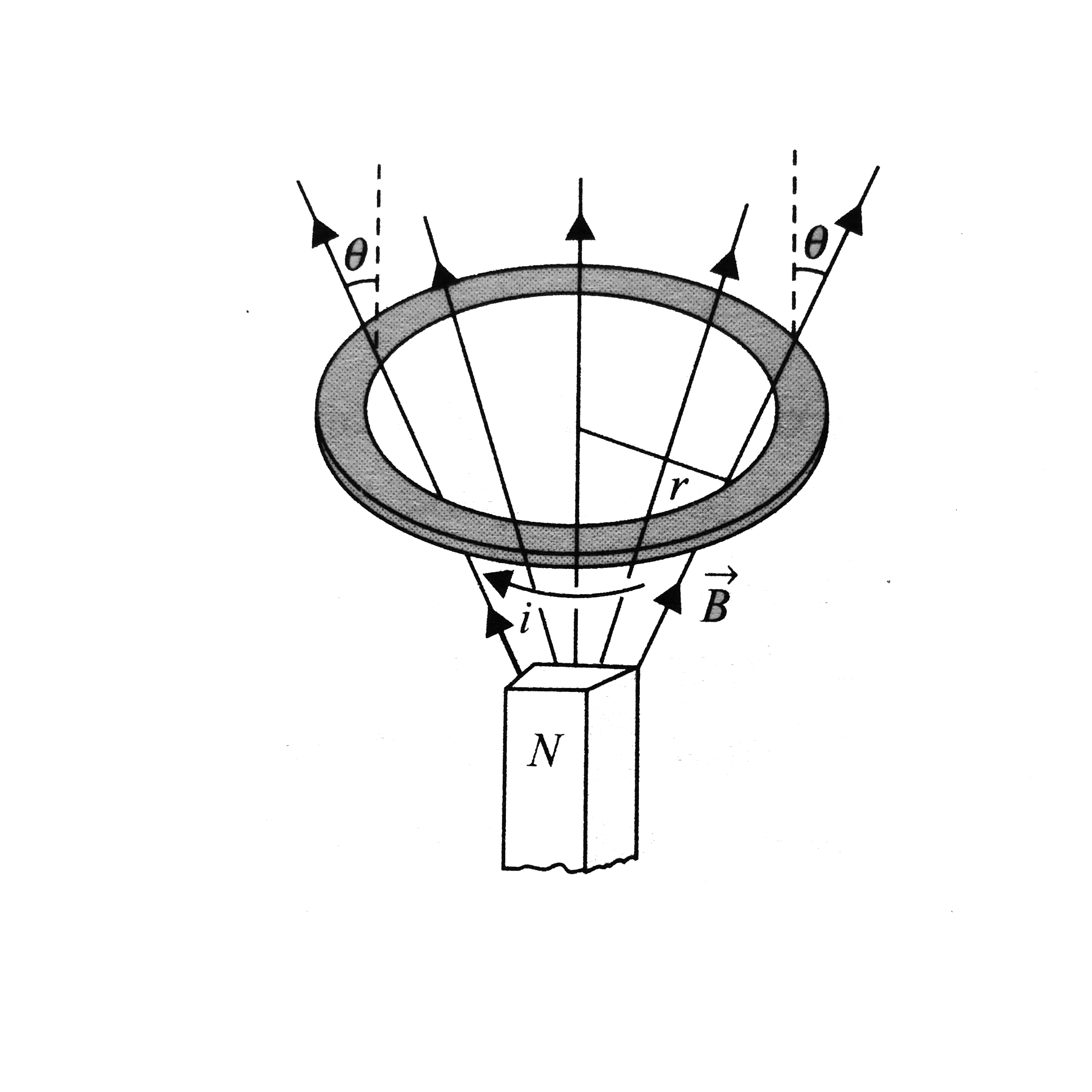
View SolutionA strong magnet is placed under a horizontal conducting ring of radius r that carries current i as shown in Fig. If the magnetic field makes an angle `theta` with the vertical at the ring's location, what are the magnitude and direction of the resultant force on the ring?
View SolutionA flat coil of `n` turns, area `A` and carrying a current `I` is placed in a uniform magnetic field of magnitude `B`. The plane of the coil makes an angle `theta` with the direction of the field. The torque acting on the coil is
View SolutionThe radius of a circular coil having 100 turns is 5 cm and a current of `0.5A` is flowing through this coil. If it is placed in a uniform magnetic field of stregth `0.001` T, then what torque will act on the coil, when the plane of the coil is inclined at `30^(@)` with the magnetic field,
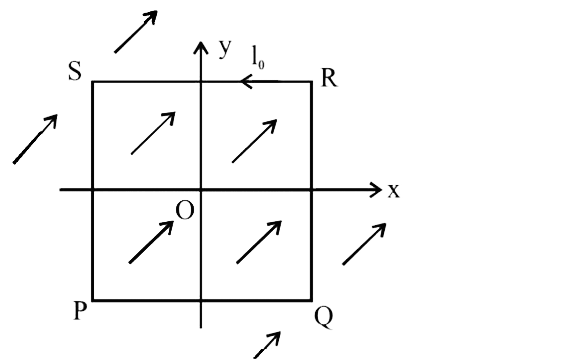
View SolutionA uniform constant magnetic field `B` is directed at an angle of `45^(@)` to the `x axis` in the ` xy`- plane . ` PQRS` is a rigid, square wire frame carrying a steady current `I_(0)`, with its centre at the origin `O`. At time ` t = 0`, the frame is at rest in the position as shown in figure , with its sides parallel to the ` x and y` axis. Each side of the frame is of mass `M` and length `L`.
(a) What is the torque `tau` about `O` acting on the frame due to the magnetic field?
(b) Find the angle by which the frame rotates under the action of this torque in a short interval of time `Deltat`, and the axis about this rotation occurs .`( Deltat is so short that any variation in the torque during this interval may be neglected .) Given : the moment of interia of the frame about an axis through its centre perpendicular to its about an axis through its centre perpendicular to its plane is `(4)/(3) ML^(2)`.
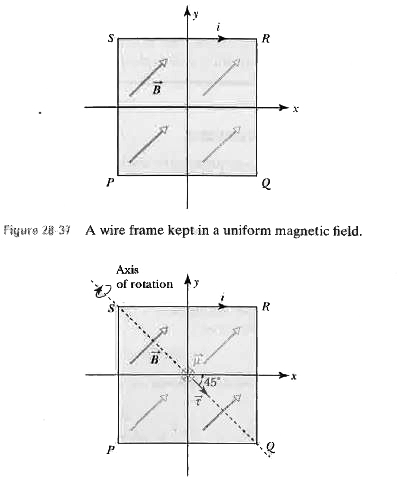
View SolutionA uniform constant magnetic field B is directed at an angle of `45^(@)` to the x-axis in the xy plane. PQRS is a rigid square wire frame carrying a steady current I (clockwise), with its center at the origin O. At time t = 0, the frame is at rest in the position shown in fig. With its sides parallel to the x and y axes. Each side of the frame is of mass M and length L.
Find the initial angular acceleration of the frame. Assume that the frame is in a gravity free space free from any other influence.
View Solution
Sample problem questions and complete solutions for chapter MAGNETIC FORCE of RESNICK AND HALLIDAY PHYSICS (HINGLISH) of Class 12