Complete Solutions to Exercise 5.2 of chapter TRIANGLES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | TRIANGLES |
| SUBHASH PUBLICATION | Exercise 5.2 |
| Number Of Questions | 7 |
| Academic Year | 2023-24 |
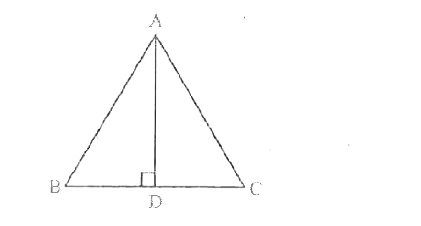
In `Delta ABC`, AD is the perpendicular bisector of BC. Show that `Delta ABC` is an isoscelss tringle in which AB = AC.
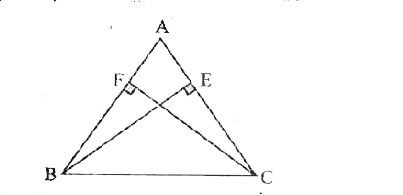
View SolutionABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively. Show that these altitudes are equal.
View SolutionABC is a triangle in which altitude BE and CF to sides AC and AB are equal. Show that
`Delta ABE ~= Delta ACF`
(ii) AB = AC, i.e., ABC is an isosceles triangle.

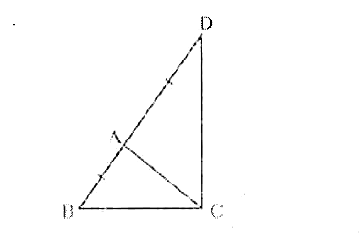
View SolutionABC and DBC are two isosceles triangles on the same base BC. Show that ABD = ACD.

View Solution`Delta ABC` is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB. Show that BCD is a right angle.
View SolutionABC is right angled triangle in which `/_ A = 90^(@)` and AB = AC. Find B and C.
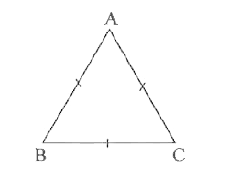
View SolutionShow that the angles of an equilateral triangle are `60^(@)` each
View Solution
Exercise 5.2 questions and complete solutions for chapter TRIANGLES of JEEVITH PUBLICATIONS MATHS (KANNADA ENGLISH) of Class 12