Complete Solutions to EXERCISE (MATHCING TYPE PROBLEMS) of chapter CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION |
| VIKAS GUPTA | EXERCISE (MATHCING TYPE PROBLEMS) |
| Number Of Questions | 3 |
| Academic Year | 2023-24 |
Let a function `f (x) =[x] {x} -|x| ` where `[.], {.}` are greatest integer and fractional part respectively then match the following List-I with List-II.


View Solution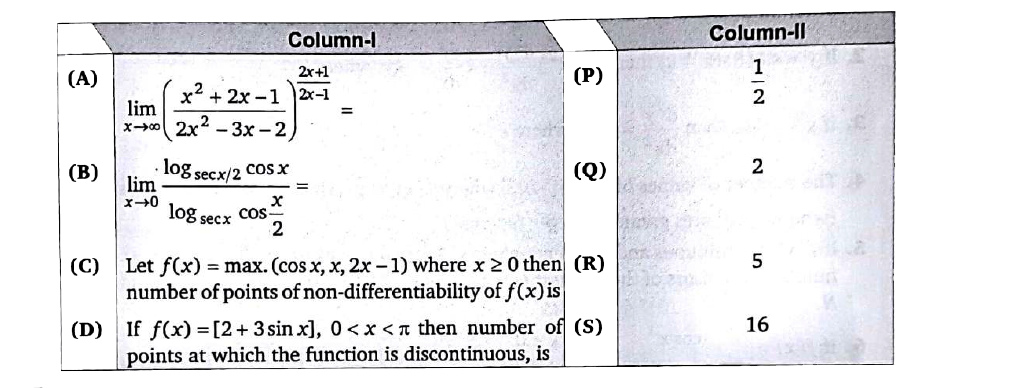
View SolutionThe function `{:(f (x) =ax (x-1)+b, x lt 1),( =x-1, 1 le x le 3),( =px ^(2)+qx +2, x gt 3):}`
if (i) f (x) is continous for all x
(ii) ` f'(1)` does not exist
(iii) `f '(x)` is continous at `x=3,` then

View Solution
EXERCISE (MATHCING TYPE PROBLEMS) questions and complete solutions for chapter CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION of SHRI BALAJI MATHS (ENGLISH) of Class 12