Complete Solutions to EXERCISE (ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT) of chapter CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION |
| VIKAS GUPTA | EXERCISE (ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT) |
| Number Of Questions | 36 |
| Academic Year | 2023-24 |
If `f (x)= tan ^(-1) (sgn (n ^(2) -lamda x+1))` has exactly one point of discontinuity, then the value of `lamda ` can be:
View Solution`f (x)= {{:(2(x+1),,, x le -1),( sqrt(1- x^(2)),,, -1 lt x lt 1), (|||x|-1|-1|,,, x ge1 ):}`, then:
View SolutionLet `f (x)= [{:((x(3e^(1//x)+4))/(2 -e^(1//x)),,,( x ne 0)),(0 ,,, x=0):} x ne (1)/(ln 2)` which of the following statement (s) is/are correct ?
View SolutionLet `|f (x)| le sin ^(2) x, AA x in R,` then
View SolutionLet `f (x)=[{:((a (1-x sin x)+ b cos x +5)/(x ^(2)),,, x lt 0), ((1+ ((dx + dx ^(3))/(dx ^(2))))^(1/x),,, x gt 0):}`
If f is continous at `x=0` then correct statement (s) is/are:
View SolutionIf `f (x)=|||x|-2|+p|` have more than 3 points non-derivability then the value of p can be :
View SolutionIdentify the options having correct statement:
View SolutionA twice differentiable function f(x)is defined for all real numbers and satisfies the following conditions `f(0) = 2; f'(0)--5 and f '' (0) = 3`. The function `g(x)` is defined by `g(x) = e^(ax) + f (x) AA x in R`, where 'a' is any constant If `g'(0) + g''(0)=0`. Find the value(s) of 'a'
View SolutionIf `f(x) = |sinx|`, then
View SolutionLet `[.]` represent the greatest integer function and `f (x)=[tan^2 x]` then :
View SolutionLet f be a differentiable function satisfying `f'(x)=f' (-x) AA x in R.` Then which of the following is correct regarding f:
View SolutionLet `f :R to R` be a function, such that `|f(x)|le x ^(4n), n in N AA n in R` then `f (x)` is:
View SolutionLet `f (x) =[x] and g (x) =0` when x is an integer and `g (x) =x ^(2)` when x is not an integer ([] is ghe greatest integer function) then:
View Solutionlet the function f be defined by `f (x)= {{:(p+ qx+ x^(2)"," , x lt 2),( 2 px+3qx ^(2)"," , x ge 2):},` Then:
View SolutionLet `f (x) =|2x-9|+|2x+9|.` Which of the following are true ?
View SolutionLet `f (x)= max (x,x ^(2) x ^(3)) in -2 le x le 2.` Then:
View SolutionLet `f(x)` be a differentiable function satisfying `f(y)f(x/y)=f(x)` `AA`, x,y`in`R, `y!=0` and `f(1)!=0`, `f'(1)=3` then
View SolutionLet `f(x) =(x^(2)-3x+ 2) (x ^(2) + 3x +2) and alpha, beta, gamma` satisfy `alpha lt beta gamma` are the roots of `f '(x)=0` then which of the following is/are correct ([.] denots greatest integer function) ?
View SolutionLet `f:RtoR` is given by
`f(x)={(p+qx+x^(2),xlt2),(2px+3qx^(2),xge2):}` then:
View SolutionLet `y=e^x s in x^3+(t a n x)^xdotF in d(dy)/(dx)dot`
View SolutionLet `f(x)=x +(1-x) x ^(2) +[1- x) (1- x^(2)) +…..+ (1-x) …….(1-x ^(n-1))x ^(n),(n ge 4)` then:
View SolutionLet `f (x)= [{:(x ^(2)+a,0 le x lt 1),( 2x+b,1le x le 2):}and g (x)=[{:(3x+b,0 le x lt 1),(x ^(3), 1 le x le 2):}`
If derivative of `f(x)` w.r.t. `g (x ) at x =1` exists and is equal to `lamda,` then which of the followig is/are correct?
View SolutionIf `f(x) = {{:((sin[x^(2)]pi)/(x^(2)-3x - 18)+ax^(2)+b",","for",0 le x le 1),(2 cos pi x + tan^(-1)x",","for",1 lt x le 2):}` differentiable function in [0, 2], where `[*]` denotes the greatest integer function, then
View SolutionLet `f (x) [ {:(1+x"," , 0 le x le 2),( 3-x"," ,2 lt x le 3):}:`
` g(x) =f (f (x)):`
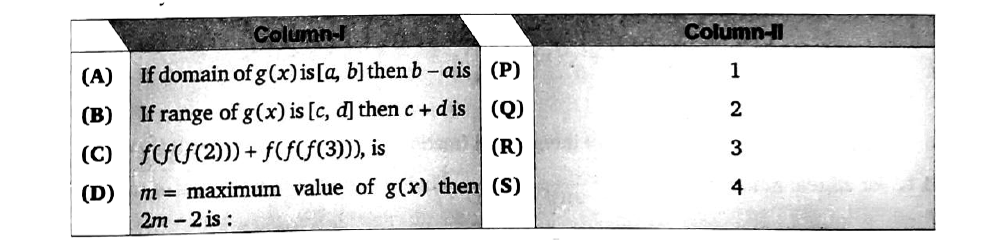
View SolutionLet `f (x)=(x+1) (x+2) (x+3)…..(x+100) and g (x) =f (x) f''(x) -f'(x) ^(2).` Let n be the numbers of real roots of `g(x) =0,` then:
View SolutionLet `f(x)={{:(,|x|-3,x lt 1),(,|x-2|+a,x ge 1):}`
`g(x)={{:(,2-|x|,x lt 1),(,Sgn(x)-b,x ge 1):}` If h(x)=f(x) is discontinuous at exactly one point, then which of the following are correct?
View SolutionLet f (x) be a continous function in `[-1,1]` such that
`f (x)= [{:((ln (ax ^(2)+ bx +c))/(x ^(2)),,, -1 le x lt 0),(1 ,,, x =0),((sin (e ^(x ^(2))-1))/(x ^(2)),,, 0 lt x le 1):}` Then which of the following is/are corrent
View Solutionf (x) is differentiable function satisfying the relationship `f ^(2) (x) + f ^(2) (y)+2 (xy-1) = f^(2) (x+y) AA x, y in R`
Also `f (x) gt 0 AA x in R and f (sqrt2)=2.` Then which of the following statement (s) is/are correct about `f (x)` ?
View Solution
The function `f (x)=[sqrt(1-sqrt(1-x ^(2)))],`(where [.] denotes greatest integer function):
i)has domain [−1,1]
ii)is discontinous at two points in its domain
iii) is discontinous at x=0
iv)is discontinous at x = 1
View SolutionA function f(x) satisfies the relation `f(x+y) = f(x) + f(y) + xy(x+y), AA x, y in R`. If f'(0) = - 1, then
View SolutionThe points of discontinuities of `f (x)= [(6x)/(pi)]cos [(3x)/(pi)]"in" [(pi)/(6), pi]` is/are:(where [.] denotes greattest integer function)
View SolutionCheck the continuity of f(x) = `{{:(x^(2)/2, if 0le x le 1),(2x^(2)-3x+3/2, if 1 lt x le 2):}` at `x = 1`
View SolutionIf `x=phi(t),y=Psi(t)," then "(d^(2)y)/(dx^(2))` is equal to
View Solution`f (x)=[x] and g (x)= {{:( 0"," , x in I ),( x ^(2)"," , cancel(in)I):}` where [.] dentoes the greatest integer function. Then
View SolutionLet `f : R ^(+) to R` defined as `f (x)= e ^(x) + `ln x and `g = f ^(-1)` then correct statement (s) is/are:
View SolutionLet `f (x)=[{:((3x-x ^(2))/(2),,, x lt 2),([x-1],,, 2 le x lt 3),( x ^(2) -8x+17,,, x ge3):}:` then which of the following hold(s) good ?
View Solution
EXERCISE (ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT) questions and complete solutions for chapter CONTINUITY, DIFFERENTIABILITY AND DIFFERENTIATION of SHRI BALAJI MATHS (ENGLISH) of Class 12