Complete Solutions to Part - C of chapter QUARILATERAL of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | QUARILATERAL |
| SUPPORT MATERIAL | Part - C |
| Number Of Questions | 10 |
| Academic Year | 2023-24 |
In figure, P is the mid-point of side BC of a parallelogram ABCD such that `angleBAP=angleDAP`. Prove that AD = 2CD. ltBrgt
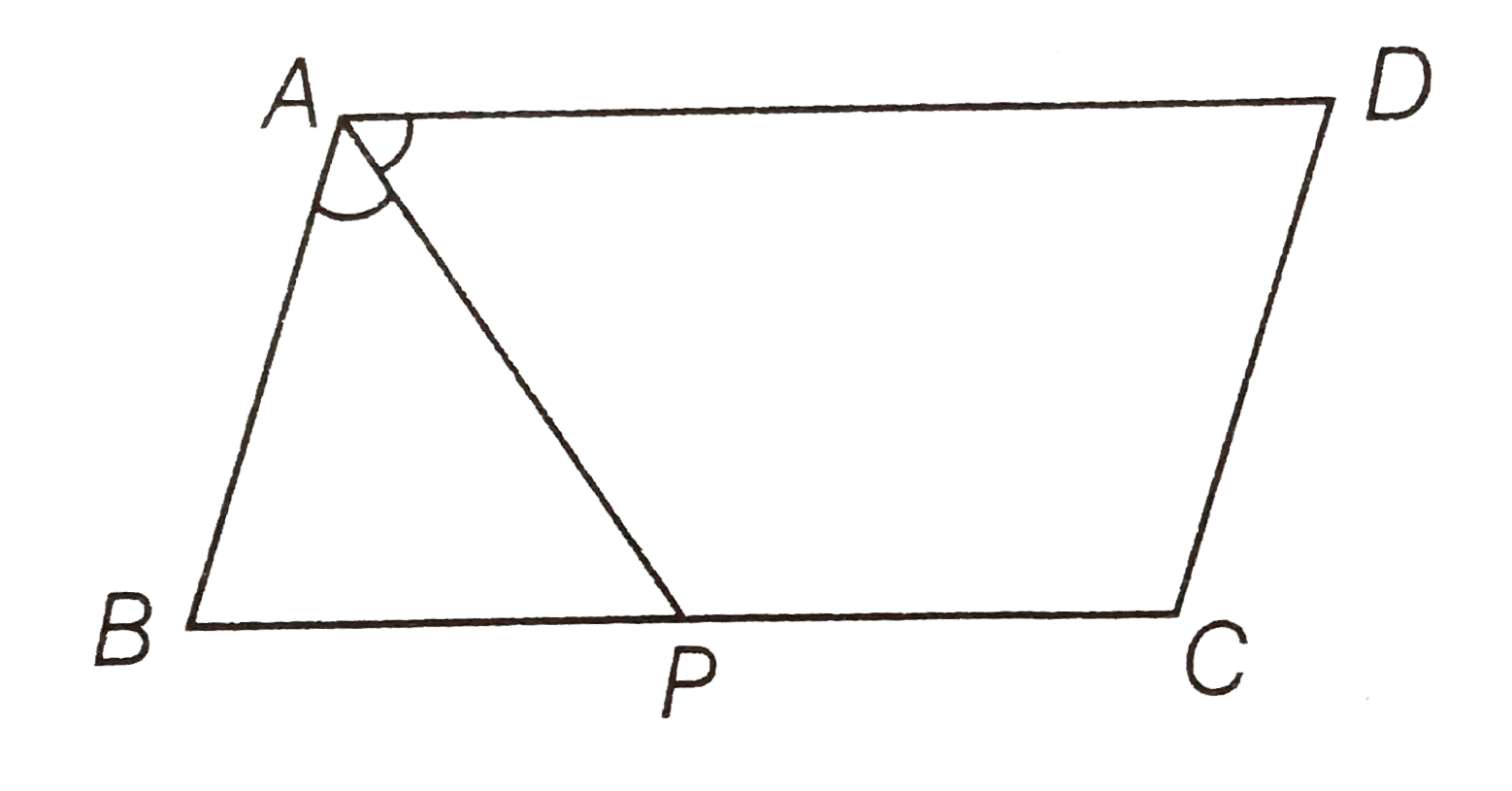 04:06View Solution
04:06View SolutionIn the adjoining figure if PQRS is a parallelogram where `anglePQR=100 and angle SPR=40`. Find `anglePRQ and angleSRQ`.
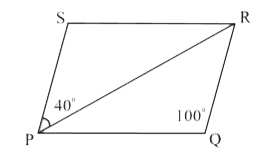 02:49View Solution
02:49View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
05:54View SolutionIn the given figure L, M and N are mid point of the sides PQ, PR and QR respectively of `DeltaPQR`. If PQ = 4.4 cm, QR = 5.6 cm and PR = 4.8 cm then find the perimeter of `DeltaLMN`.
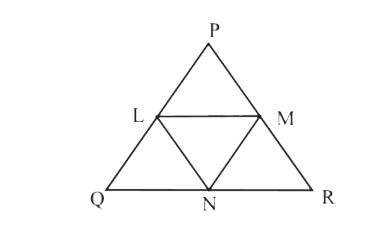 03:48View Solution
03:48View SolutionA quadrilateral is parallelogram; if itsone pair of opposite sides are equal and parallel
02:10View SolutionIf the diagonals of a quadrilateral bisect each other; then the quadrilateral is a parallelogram.
10:20View SolutionIn a parallelograms PQRS, M and N are points on PQ and RS such that PM = RN. Prove that MS || NQ.
07:59View SolutionABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD . Show that(i) `DeltaA P B~=\ DeltaC Q D`(ii) `A P\ =\ C Q`
01:32View SolutionThe diagonals of a rectangle ABCD meet at O. If `angleBOC=50^(@)` then find `angleODA`.
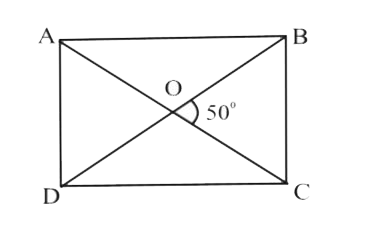 03:19View Solution
03:19View SolutionIn the given figure AD and BE are the medians of `DeltaABC` and BE || DF . Prove that `CF=1//4 AC`.
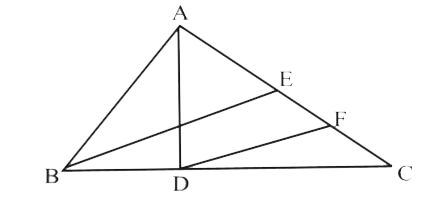 06:13View Solution
06:13View Solution
Part - C questions and complete solutions for chapter QUARILATERAL of CBSE COMPLEMENTARY MATERIAL MATHS (HINGLISH) of Class 9
