Complete Solutions to EXERCISE 11.3 of chapter AREAS OF PARALLELOGRAMS AND TRIANGLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | AREAS OF PARALLELOGRAMS AND TRIANGLES |
| CPC CAMBRIDGE PUBLICATION | EXERCISE 11.3 |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
In the Given figure, E is any point on median AD of a ` triangle ABC` Show that ar (ABE) = ar (ACE)
View SolutionIn a triangle ABC, E is the mid - point of median AD. Show that ar (BED) ` =1/4 ar (ABC)
View SolutionShow that the diagonals of a parallelogram divide it into four triangles of equal area.
View SolutionIn the given figure, ABC and ABD are two triangles on the same base AB . If line - segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD)
View SolutionD,E and F are respectively the mid-points of the sides BC, CA and AB of ` triangle ABC` show that
(i) BDEF is a parallelogram.
(ii) ar (DEF) = `1/4` ar (ABC)
(iii) ` ar (BDEF) = 1/2` ar (ABC)
View SolutionIn the given figure, diagonals AC and BD of quadrilateral ABCD interset at O such that OB = OD. If AB = CD, then show that :
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram .
View SolutionD and E are points on sides AB and AC respectively of `tirangle ABC` such that ar (DBC) = ar (EBC) . Prove that DE || BC
View SolutionXY is a line parallel to side BC of a triangle ABC. If BF ||AC and CF || AB meet XY at E and F respectively, show that ar (ABE) = ar (ACF)
View SolutionThe side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed . Show that ar (ABCD) = ar (PBQR)
View SolutionDiagonals AC and BD of a trapezium ABCD with AB || DC interseet each other ar at O. Prove that ar (AOD) = BOC.
View SolutionIn the figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)
View SolutionA villager Itwaari has a plot of land of the shape of a quadrillateral. The Gram Panchayat of the village decieded to take over some portion of his plot from one of the corners to construct a Health Centre. Itwaari agrees to the above proposal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as form a triangular plot. Explain how this proposal will be implemented.
View SolutionABCD is a trapezium with AB || DC,. A line parallel to AC interseets AB at X and BC at Y. Prove that ar (ADX) = ar (ACY)
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC) . Prove that ABCD is a trapezium.
View SolutionIn the given figure , ar (DRC) = ar ( DPC) and ar(BDP) = ar(ARC) . Show that both the quadrillaterals ABCD and DCPR are trapeziums.
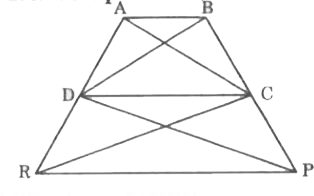
View Solution
EXERCISE 11.3 questions and complete solutions for chapter AREAS OF PARALLELOGRAMS AND TRIANGLES of CAMBRIDGE MATHS (KANNADA ENGLISH) of Class 9