Complete Solutions to EXERCISE 11.4 of chapter AREA OF PARALLELOGRAMS AND TRIANGLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | AREA OF PARALLELOGRAMS AND TRIANGLES |
| SUBHASH PUBLICATION | EXERCISE 11.4 |
| Number Of Questions | 3 |
| Academic Year | 2023-24 |
Parallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
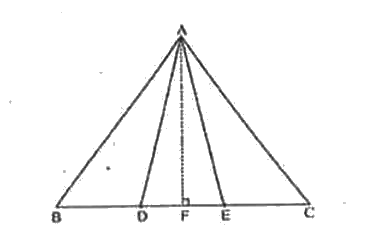
View SolutionIn, D and E are two points on BC such that BD = De = EC. Show that ar (ABD) = ar (ADE) = ar (AEC).
Can you now answer the question that you have left in the 'introduction' of this chapter, whether the field of Budhia has been actually divided into three parts of equal area?
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that ar (APB) `xx` ar (CPD) = ar (APD) `xx` ar (BPD).
[Hint : From A and C, draw perpendiculars to BD.
View Solution
EXERCISE 11.4 questions and complete solutions for chapter AREA OF PARALLELOGRAMS AND TRIANGLES of VIDHYASANGAM - RAO'S ACADEMY MATHS (KANNADA ENGLISH) of Class 9