Complete Solutions to EXERCISE 1.5 of chapter SET LANGUAGE of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | SET LANGUAGE |
| SURA PUBLICATION | EXERCISE 1.5 |
| Number Of Questions | 19 |
| Academic Year | 2023-24 |
Using the adjacent venn diagram, find the following sets :
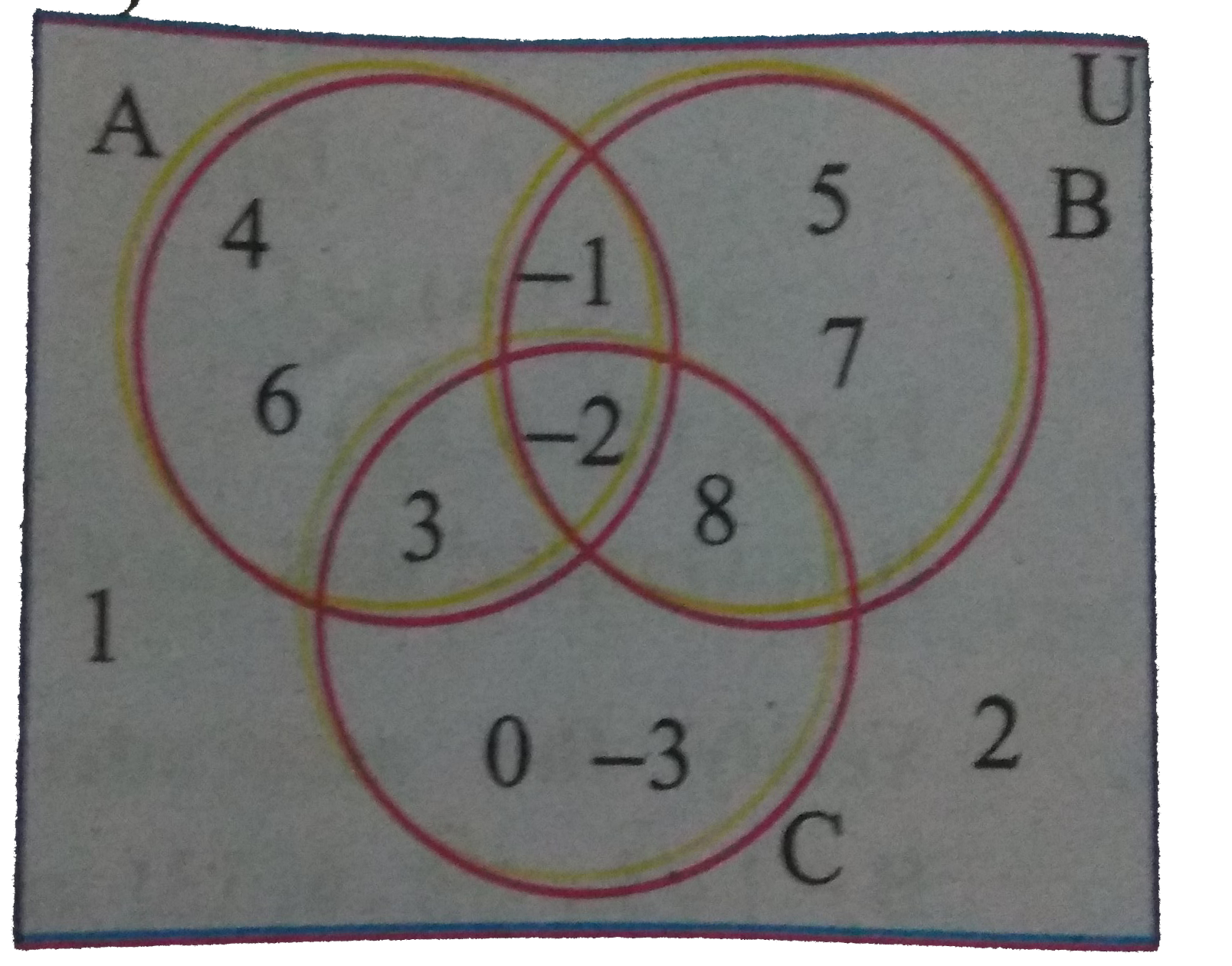
(i) A - B
(ii) B - C
(iii) `A'cupB'`
(iv) `A'capB'`
(v) `(BcupC)'`
(vi) `A-(BcupC)`
(vii) `A-(BcapC)`
View SolutionIf K = {a, b, d, e, f}, L = {b, c, d, g} and M = (a, b, c, d, h} then find the following:
`Kcup(LcapM)` and verify distributive laws.
View SolutionIf K = {a, b, d, e, f}, L = {b, c, d, g} and M = (a, b, c, d, h} then find the following:
`Kcap(LcupM)` and verify distributive laws.
View SolutionIf K = {a, b, d, e, f}, L = {b, c, d, g} and M = (a, b, c, d, h} then find the following:
`(KcupL)cap(KcupM)` and verify distributive laws.
View SolutionIf K = {a, b, d, e, f}, L = {b, c, d, g} and M = (a, b, c, d, h} then find the following:
`(KcapL)cup(KcapM)` and verify distributive laws.
View SolutionIf `A={x:x inZZ,-2ltxle4},B={x:x inW,xle5}`, C = {-4, -1, 0, 2, 3, 4}, then verify `Acup(BcapC)=(AcupB)cap(AcupC)`.
View SolutionVerify `Acup(BcapC)=(AcupB)cap(AcupC)` using Venn diagrams.
View SolutionIf A = {b, c, e, g, h}, B = {a, c, d, g, i} and C = {a, d, e, g, h}, then show that `A-(BcapC)=(A-B)cup(A-C)`.
View SolutionIf `A={x:x=6n,ninWandnlt6},B={x:x=2n,ninNNand2ltnle9}andC={x:x=3n,ninNNand4lenlt10}`, then show that `A-(BcapC)=(A-B)cup(A-C)`
View SolutionIf A = {-2, 0, 1, 3, 5}, B = {-1, 0, 2, 5, 6} and C = {-1, 2, 5, 6, 7}, then show that `A-(BcupC)=(A-B)cap(A-C)`.
View SolutionIf `A={y:y=(a+1)/2,ainWandale5},B={y:y=(2n-1)/2,ninWandnlt5}andC={-1,-1/2,1(3)/(2),2}`, then show that `A-(BcupC)=(A-B)cap(A-C)`.
View SolutionVerify `A-(BcapC)=(A-B)cup(A-C)` using Venn diagrams.
View SolutionIf U = {4, 7, 8, 10, 11, 12, 15, 16}, A = {7, 8, 11, 12} and B = {4, 8, 12, 15}, then verify De Morgan's Laws for complementation.
View SolutionVerify `(AcapB)'=A'cupB'` using Venn diagrams.
View SolutionIf A = {1, 3, 5, 7, 9}, B = {x : x is a composite number and `xlt12`} and C = `{x:x inNand6ltxlt10}` then verify `Acup(BcapC)=(AcupB)cap(AcupC)`.
View SolutionIf A, B and C are overlapping sets, draw venn diagram for : `AcapB`
View SolutionDraw Venn diagram for `AcapBcapC`
View SolutionIf P = `{x:x inNNand1ltxlt11},Q={x:x=2n,ninNNandnlt6}` and R = {4, 6, 8, 9, 10, 12}, then verify `P-(QcapR)=(P-Q)cup(P-R)`.
View SolutionIf `U={x:x inZZ,-3lexle9},A={x:x=2P+1,P inZZ,-2lePle3},B={x:x=q+1,qinZZ,0le q le3}`, verify De Morgan's laws for complementation.
View Solution
EXERCISE 1.5 questions and complete solutions for chapter SET LANGUAGE of SURA MATHS (TAMIL ENGLISH) of Class 9