Complete Solutions to QUESTION BANK of chapter Parallel Lines of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | Parallel Lines |
| V PUBLICATION | QUESTION BANK |
| Number Of Questions | 49 |
| Academic Year | 2023-24 |
Draw an 8 centimetres long line and divide it in the ratio 2.: 3.
01:59View SolutionDraw a rectangle of perimeter 15 cm and sides are in the `3:4`.
03:52View SolutionDraw triangles specified below, each of perimeter 10 centimetres.
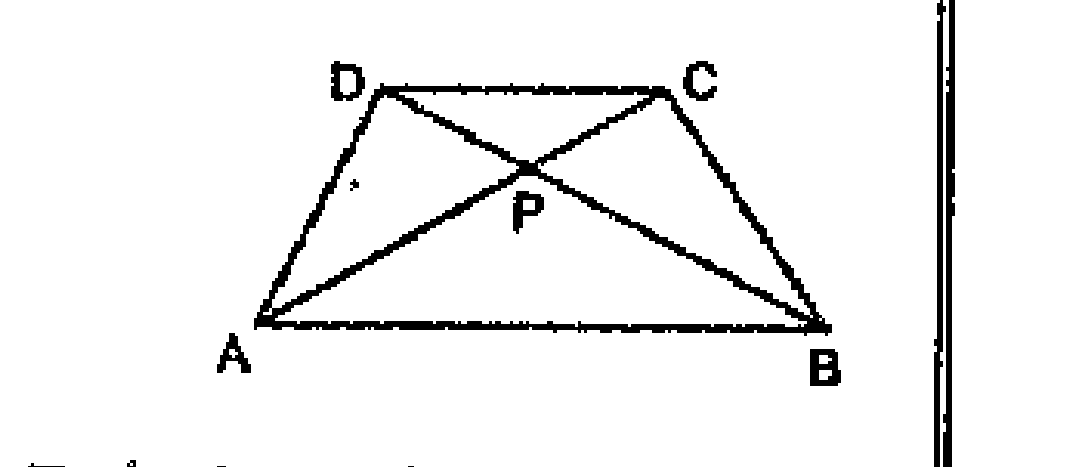
i) Equilateral.triangle ii) Sides in the ratio 3: 4: 5 iii) Sides in the ratio 2: 3: 402:39View SolutionIn the picture below, the diagonals of the trapezium `ABCD` intersect at P.
Prove that
`PAtimesPD=PBtimesPC`.
 .01:53View Solution
.01:53View SolutionDraw a triangle of perimeter 15 centimetres and sides are in the ratio 3:3:4
04:26View SolutionDraw a rectangle of perimeter 20 centimetres and sides in the ratio3:4
04:26View SolutionProve that diagonals of trapezium cut each other proportionally.
03:43View SolutionDraw a triangle of perimeter 13.7 cm and sides are in the ratio 2: 3: 4.
04:26View SolutionDraw a triangle of perimeter 14 cm and sicies in the ratio 3: 4: 5.
05:19View SolutionIn the picture, the perpendicular is drawn from the midpoint of the hypotenuse of a right triangle. to the base. Calculate the length of the third side of the large right triangle and the lengths of all three sides of the small right triangle.
04:33View SolutionDraw a right triangle and the perpendicular from the midpoint of the hypotenuse to the base.
i) Prove that this perpendicular is half the perpendicular side of the large triangle.
ii) Prove that this perpendieular bisects the bottom side of the larger triangle.
iiii) Prove that in the large triangle, the distances from the midpoint of the hypoteniuse to all the vertices are equal.
iv) Prove thàt the circumcentre of a right triangle is the midpoint of its hypotenuse.01:13View SolutionIn the parallelogram `ABCD`, the line drawn through `Q` point `P` on `AB`, parallel to `BC` meets `AC` at `Q`. The line through `Q`, parallel to `AB` meets `AD` `R`.
Prove that
`(AP)/(PB)=(AR)/(RD)`.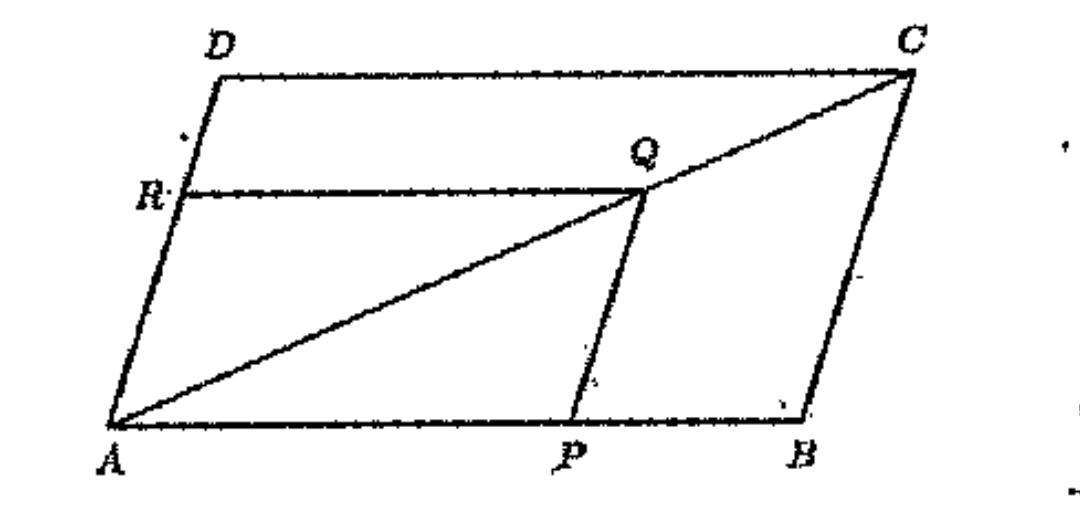 .02:29View Solution
.02:29View SolutionIn the picture below, two vertices of a parallelogram are joined to the mid points of two sides.
Prove that these lines divides the diagonal in the picture into three equal parts.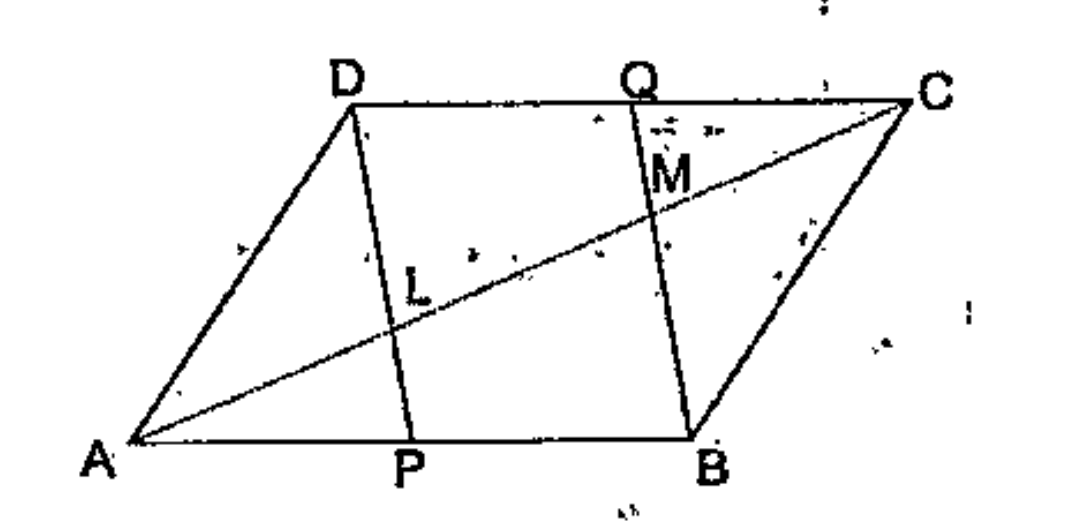 .03:11View Solution
.03:11View SolutionProve that the quadrilatral formed by joining the mid points of the sides of a quadrilateral is a parallelogram.
03:44View SolutionIn trapezium ABCD, CD is parallel to AB. E and F are points on the non parallel sides. If EF is parallel to AB, then
prove thatAE/ED=BF/FC04:39View SolutionIn ABC,AB=6cm . P is a point on AB.The line drawn parallel to BC through P meets AC at Q. If the length AP is double the length of PB and the length of AQ is 1 cm more than QC, what is the length of AC ?
03:57View SolutionIn triangle ABCthe length of AB is 6 centimetres and the length of AC is 5 centimetres. The length of AP is 4 centimetres. The line PQ is parallel to BC.
Find the lengths of AQ and QC.03:22View SolutionIn triangle ABC, a line parallel to BC cuts AB and AC at P and Q. Show that AP/AB= AQ/A C
02:56View SolutionABCD is a quadrilateral in which P,Q, R and S are midpoints of the sides AB , BC, CD and DA .AC is a diagonal. Śhow that
i) SR is parallel to AC, and SR=`1/2` AC.
ii) P Q=SR
iii) PQRS is a parallelogram.08:57View SolutionGiven triangle ABC, lines are drawn through A, B and C parallel respectively to the sides BC, CA and AB forming PQ .
Show that BC=`1/2`QR04:45View SolutionIn ABC, the line through a point P on. BC, parallel to AC meets AB at Q. The line through Q, parallel to AP, meet BC at R
06:29View SolutionAB and CD are parallel lines in the picture.
Prove that AP .PC=BP. PD02:52View SolutionThe sides of the triangle are.in the ratio 2: 2: 3 and its perịmeter is 12cm. Draw the triangle.
02:40View SolutionIn the figure, MN is parallel to QR. PM =6 centimetres, P Q=10 centimetres.
a) What is the length of MQ?
b) What is PN : NR?03:08View SolutionIn the figure, PQ is parallel to BC PA: PB=2:3. AB=5centimetres,AQ=4 centimeters.
a) Find AQ: QC.
b) What is the length of QC ?
c) Find AQ: AC04:00View SolutionIn the figure, 0 is the centre of the clrcle. angle P=angle R=90, OP=2 centimetres PR=RB.
a) What is the measure of angle B ?b) What is the length.of BC?
c) Find the length of QR.03:45View SolutionPQR are the mid polints of șides of tiangle ABC
a) if BC=10centimetres, what is the length of PQ?
b) If the area of triangle APQ is x, what is the area of triangle ABC? c) Find area of parallelogram PQRB. d) Draw a triangle and then draw a parallelogram with area half the area of fhe friangle.01:11View Solutionin the figure, the vertical lines are parallel. AR:RP:PB=1:2:2 AS=TS, QC=QT
a) What is the ratio of sides of triangle TSQ?
b) Find the perimeter of the triangle if Sa =6 centimeters.
c) What is the length of AC?03:25View SolutionThe sidcs of rectrigle die 6 centimetres and 2 centimetres.
a) Dira the rectangle.
b) Drew another rectangle of the same perimefer with sides in the ratio 5: 404:26View SolutionDraw a rectangle of perimeter 18 centimetres and sides in the ratio 3: 4.
04:26View SolutionIn the figure, BC=10 cm, AD=6 cm < PQB = < ABD, < PRC = < ACD, AP = 3 cm, then
a) What is the area of triangle ABC?
b) What is the ratio A D: P D ?
c) Calculate the area of triangle PQR.09:55View SolutionIn the figure, XY is parallel to Q R. P X=2 cm, Q X=4 cm, P R=9 cm.
a) Find PY:YR.
b) Find the length of PY.04:33View SolutionDraw a line of length 13 centimetres and divide it in the ratio 1: 2: 3
01:59View SolutionThe midpoints of sides of triangleABC are P, Q, R, If the perimeter of triangle PQR is 18 centlimetres.
a) How many times of PQ is BC ?
b) What is the perimeter of triangle ABC ?03:07View SolutionIn the figure, the horizontal Iines are. paraliel. The distance between them are3 centimetres, 4 centimetres, 5 centimetres respectively. If Q R=8 centimetres then
a) Find the ratio P Q: Q R: RS
b)if RS=RT,PQ=QT,calculate perimeter of triangle QRT.02:58View SolutionIn the following figure, the sides AB, AD and the diagonal AC. of the quadrilateral ABCD are extended and marked the points C, E, F. Also EF, FG are parallel to the 'sides DC, CE respectlyely and A C: C F=2: 1.
a) Find the ratio AB:BG.
b) If A B=10 centimetres, what is AG?
c) How maniy times the perimeter of quadrilateral ABCD?03:30View SolutionThe indpolnts of the sides of the quadrilateral ABCD are P, R,S respectively. When they are joined a. rectingle is obtained :
o) Which the lines parallel to BD?
b) What is the angle between the diagonals of quadrilateral ABCD? Why?
c) What types of quadrilaterals make a rectangle when the midpoints of Its sldes are joined?01:50View SolutionDraw a triangle of perimater 11 centimetres and sides In the ratio 2: 3: 3.
04:26View SolutionIn the figure, AB, CD and EF are paralIel: PC=4 cm, CE=2cm PD=6 cmFind DF and PF.
04:19View SolutionDraw a triangle of perimeter 12 centimetres and sides in the ratio 1: 3: 3.
04:26View SolutionIn the figure, P and Q are midpoints of sides AC and B C of the triangle A B C. R and s are midpoints of lines BC and Ac rospectively.
I) Find A C: C Q wf
ii) Prove that PQRS is a parallelogram.04:57View SolutionIn triangle A B C, angle C=`90^circ`, A C=12 cm, BC=16 cm and CD Is perpendicular to AB.
i) Find the length of AB.
ii) Find the length of A D and B D.
Iii) Find the length of CD.08:56View SolutionDraw a rectangle of sides 7 centimetre. and 5 centimetre. Construct another rectarigle of. Iength 8 centimetre without. changing the ratio of their sides.
02:28View SolutionIn triangle ABC, AP=PQ,angle C=angle Q AP=6 centimetre, P B=8 centimetre, A Q =9 centimetre.
i) Write the pairs of equal angles from the figure.
ii) Prove that triangle A B C is isosceles.
iii) Find the perimeter of the trapezium PBCQ.05:06View SolutionDraw a triangle of perimeter 15 centime tres and sides are in the ratio 2: 3: 3.
04:26View SolutionIn the figure, AB, CD and EF are parallel. PC=6 cm, CE=3cm, PD=8cm. Find DF and PF.
05:02View SolutionIn triangle PQR, angle R=90 , PR=12cm, QR=16cm and RS is perpendicular to PQ.
1) . Find the length of PQ.
ii) Find the length of PS and SQ.
ii) Find the length of RS.05:42View SolutionIn triangle ABC, a line parallel to BC meets AB and AC at D and E respectively. If AE=4.5, cm, AD/DB =2/5.What is the length of EC ?
02:35View SolutionIn the figure, ABCD is a trapezium, AB and DC are parallel lines. P is the midpoint of AD. If PQ is parallel to AB, prove thatQ is the midpoint of BC.
05:00View Solution
QUESTION BANK questions and complete solutions for chapter Parallel Lines of V PUBLICATION of Class 9