Exercise (B) from BHARATI BHAWAN PHYSICS Chapter 18 LAWS OF MOTION, FREE BODY DIAGRAM for Class 11
| Class 11 | PHYSICS |
| Chapter | LAWS OF MOTION, FREE BODY DIAGRAM |
| BHARATI BHAWAN PHYSICS | Exercise (B) |
| Medium | English |
| Academic Year | 2023-2024 |
Question 1
A train of mass M is travelling with a uniform velocity on a level line . The last carriage whose mass is m gets detached from the train . The driver of the train discovers it only after travelling a distance l and then he shuts off steam . Show that when both parts come to rest the distance between them is (MlM−m) . Assume resistance of motion to be uniform and proportional to mass and the pull of the engine to be a constant . View SolutionQuestion 2
A cannon and a supply of cannon balls are inside a sealed rail road car. The cannon fires to the right, the car recoils to the left. The canon balls remain in the car after hitting the far wall. Show that no matter how the cannon balls are fired, the rail road car cannot travel more than L. assuming it starts from rest.
 View Solution
View SolutionQuestion 3
A jet airplane is travelling at 200ms−1 . The engine of the plane takes in 250 m3 of air of mass 32 kg each second . The air is used to burn 3 kg of fuel each second . The energy is used to compress the products of combustion to eject them at the rear of the plane at 500ms−1 relative to the plane . Find the power of the jet plane in H.P.(1 H.P = 746 W).
[Hint : Resultant thrust = forward thrust - backward thrust and power = force × velocity ]View SolutionQuestion 4
Sand drops from a stationary hopper at the rate of 5 kg s−1 on to a conveyor belt moving with a constant speed of 2ms−1 . What is the force required to keep the belt moving and what is the power delivered by the motor moving the belt ?
[ Hint: Tangetial force exerted on the belt = ureldMdt where urel is the tangential relative velocity of the sand .]View SolutionQuestion 5
A right-angled triangular block of mass M rests on a horizontal table . The inclination of the block is 30∘ with the table . A cubical block of mass m is placed on the incline of the block . What horizontal force must be applied on M so that m may remain stationery relative to the block ?
[Hint: Apply d' Alember's principal and consider free-body diagrams of m and the system ( m+ M).]View SolutionQuestion 7
A wedge of mass M rests with one face in contact with horizontal , smooth surface . A particle of mass m is placed on the smooth face on it , inclined at an angle αto the horizontal and released . Find the velocity of the wedge and that of the particle relative to the wedge when the particle has slipped a distance x down the face of the wedge . Also calculate the acceleration of the wedge and that of the particle relative to the wedge and the smooth surface .
[Hint : Apply principal of conservation of momentum along the horizontal and energy conservation principle . See Example 8 )View SolutionQuestion 8
Two identical buggies 1 and 2 with one man in each move without friction due to inertia along the parallel rails toward each other. When the buggies get opposite each other, the men exchange their places by jumping in the direction perpendicular to the motion direction. As a consequence, buggy 1 stops and buggy 2 keeps moving in the same direction, with its velocity becoming equal to v. Find the initial velocities of the buggies v1 and v2 if the mass of each buggy (without a man) equals M and the mass of each man m.View SolutionQuestion 9
Two identical buggies move one after the other due to inertia (without friction) with the same velocity v0. A man of mass m rides the rear buggy. At a certain moment the man jumps into the front buggy with a velocity u relative to his buggy. Knowing that the mass of each buggy is equal to M, find the velocities with which the buggies will move after that. View SolutionQuestion 10
Assuming that the car in problem 12 is initially at rest and holds n men, each of mass m, prove that if the men run with relative velocity vrel and jump in succession they can impart to the car a greater velocity than if they all run and jump simultaneously.
[Hint : When the men run together, the effect is the same as for one man of mass nm.
∴Δv=nmM+nmvrel.
When they run and jump in succession,
Δv=i=n∑i=1=mvrelM+im=mvrel(1M+m+1M+2m+1M+3m+...+1M+nm)] View SolutionQuestion 11
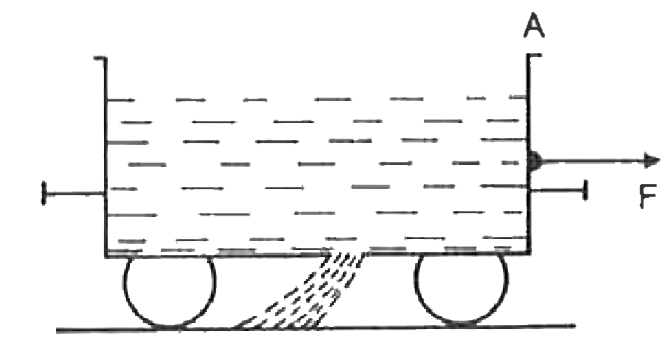
Find an expression for, the acceleration and speed of a trolley A under the action of a constant force F if it contains sand that pours out through the floor at the rate of μ per second. The speed of the trolley at t=0 was v=0 and the initital mass of sand and trolley was M0
 View Solution
View SolutionQuestion 14
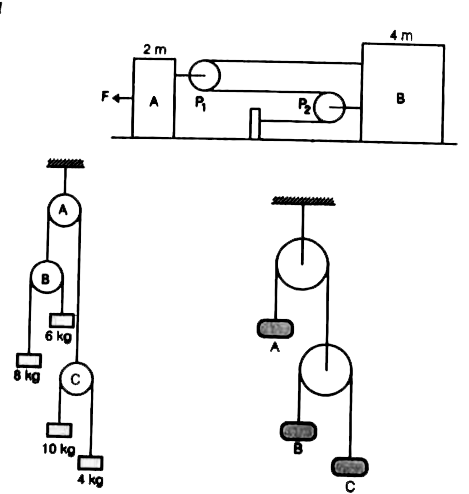
A body of mass m is drawn up a smooth inclined plane (Figure 5.21) of height h and length l by means of a string passing over the vertex of the plane. From the other end of the string hangs a mass m'. Show that in order that m may just reach the top of the plane, m' msut be deteched after m has moved through a distance m+m'm'hlh+l
 View Solution
View SolutionQuestion 19
A rope is stretched between two stationary boats on the surface of a lake. A man in the first boat pulls the rope with a constant force of 100 N. The distance between the boats is 100 m in the beginning. The mass of the first boat with the man is 250 kg ,while the mass of the other is 500 kg. Find :(i) the velocity of approach of the two boats 15 s after the start,(ii) the time taken for the two boats to meet each other. [Hint: Consider relative acceleration and apply kinematic equations.]View SolutionQuestion 21
A mass M attached to the end of a small flexible rope of diameter d=1 cm is raised vertically by winding the rope on a reel. If the reel is turned uniformly ay the rate of n=2 rps, what will be the tension T in the rope? Neglect inertia of the rope and slight lateral motion of the suspended mass. (Fig.5.25)
 View Solution
View SolutionQuestion 24
A simple pendalum is suspended from a peg on a verticle wall . The pendulum is pulled away from the well is a horizental position (see fig) and released . The bell his the well the coefficient of resitution being 2√5
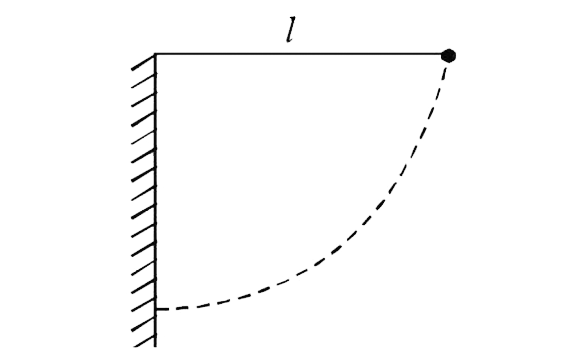
what is the miximum number of colision after which the amplitube of secillections between less that 60 digree ? View SolutionQuestion 25
A small metal ball falls vertically and strickes a smooth plane inclined at an angle θ to the horizontal (θ<45∘). The ball rebounds horizontally. Show that a fraction (l-e) of the kinetic energy is lost during the impact and that if m is the mass and μ the speed of the ball before impact, the impulse on the plane is μsecθ. Here e isi the coefficient of restitutuon. View SolutionQuestion 29
A particle of mass 1.0g moving with velocity v1=3.0i−2.0j experiences a perfectly inelastic collision with another particle of mass 2.0g and velocity v2=4.0j−6.0k. Find the velocity of the formed particle (both the vector v and its modulus), if the components of the vectors v1 and v2 are given in the SI units. View SolutionQuestion 30
A ball moving translationally collides elastically with another, stationary, ball of the same mass. At the moment of impact the angle between the straight line passing through the centres of the balls and the direction of the initial motion of the striking ball is equal to α=45∘. Assuming the balls to be smooth, find the fraction η of the kinetic energy of the striking ball that turned into potential energy at the moment of the maximum deformation. View SolutionQuestion 32
To raise a weight which is half as much again as his weight a man fastens a rope to it and passes over a smooth pulley, he then climbs up the rope with an acceleration 6g7 relative to the rope. Show that the weight rises with acceleration 87, and find the tension of the rope.View Solution