In the network of Fig. determine the current flowing through the branch of 4 Ω resistance using nodal analysis.
We will find voltages VA and VB by using Nodal analysis and then find the current through 4Ω resistor by dividing their difference by 4.V21/5 + 1/4 + 1/j2 - VB/4 - 50∠30º/5 = 0∴ VA9 −j10 − 5VB = 200 ∠30º ...i Similarly from node B we haveVB1/4 + 1/2 + 1/-j2 - VA/4 - 50 ∠ 90º/2 = 0∴ VB 3 + j2 −VA = 100 ∠90º = j 100 ...iiVA can be eliminated by multiplying. Eq. ii by 9−j10 and adding the result. ∴ VB42 −j12 = 1173 + j1000 or VB = 1541.4 ∠ 40.40º/43.68 ∠-15.9º = 35.29∠56.3º = 19.58 + j29.36Substituting this value of VB in Eq. ii we get VA = VB3 + j2 −j100 = 19.58 + j29.36 3 + j2 −j100 = j27.26 ∴ VA −VB = j 27.26 − 19.58 −j29.36 = − 19.58 −j2.1 = 19.69∠186.12º ∴ I2 = VA −VB/4 = 19.69∠186.12º/4 = 4.92∠186.12º
Related Solutions
Determine the value of R, in the following network, if no current flows through the branch AB.
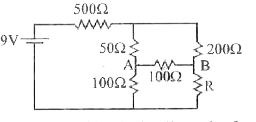 View Solution
View SolutionDetermine the current in each branch of the network shown in Fig.
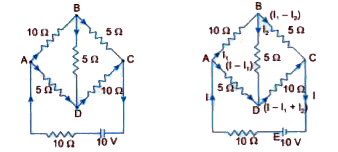 View Solution
View SolutionDetermine the current in each branch of the network shown in fig.
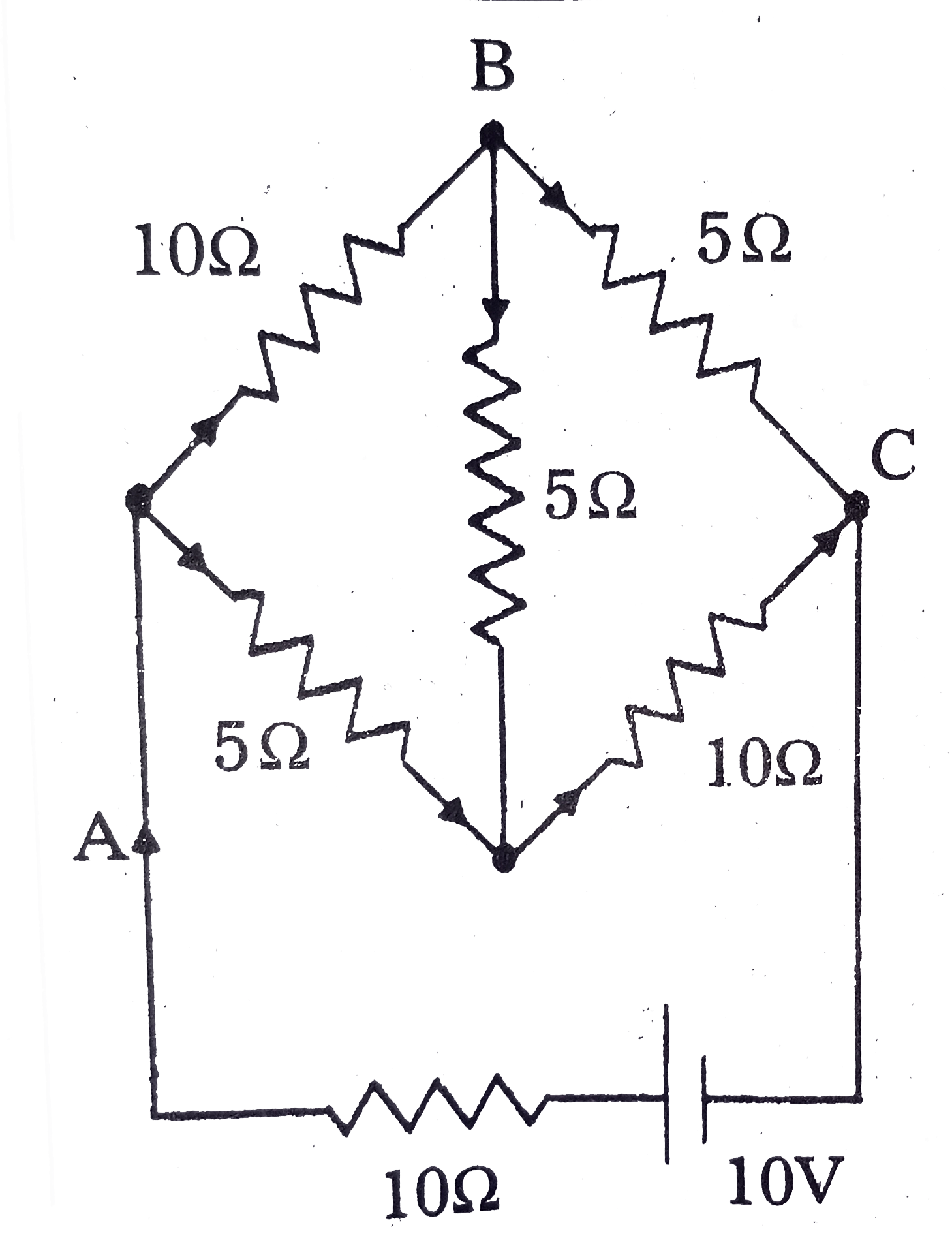 View Solution
View SolutionDetermine the current in each branch of the network shown in Fig.
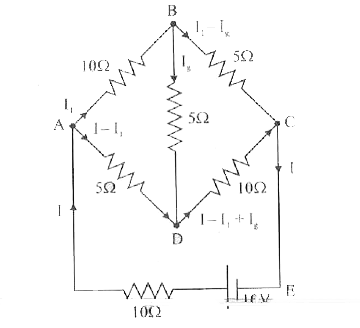 View Solution
View SolutionDetermine the current in each branch of the network shown in fig.
View SolutionDetermine the current in each branch of the network shown in fig.
View Solution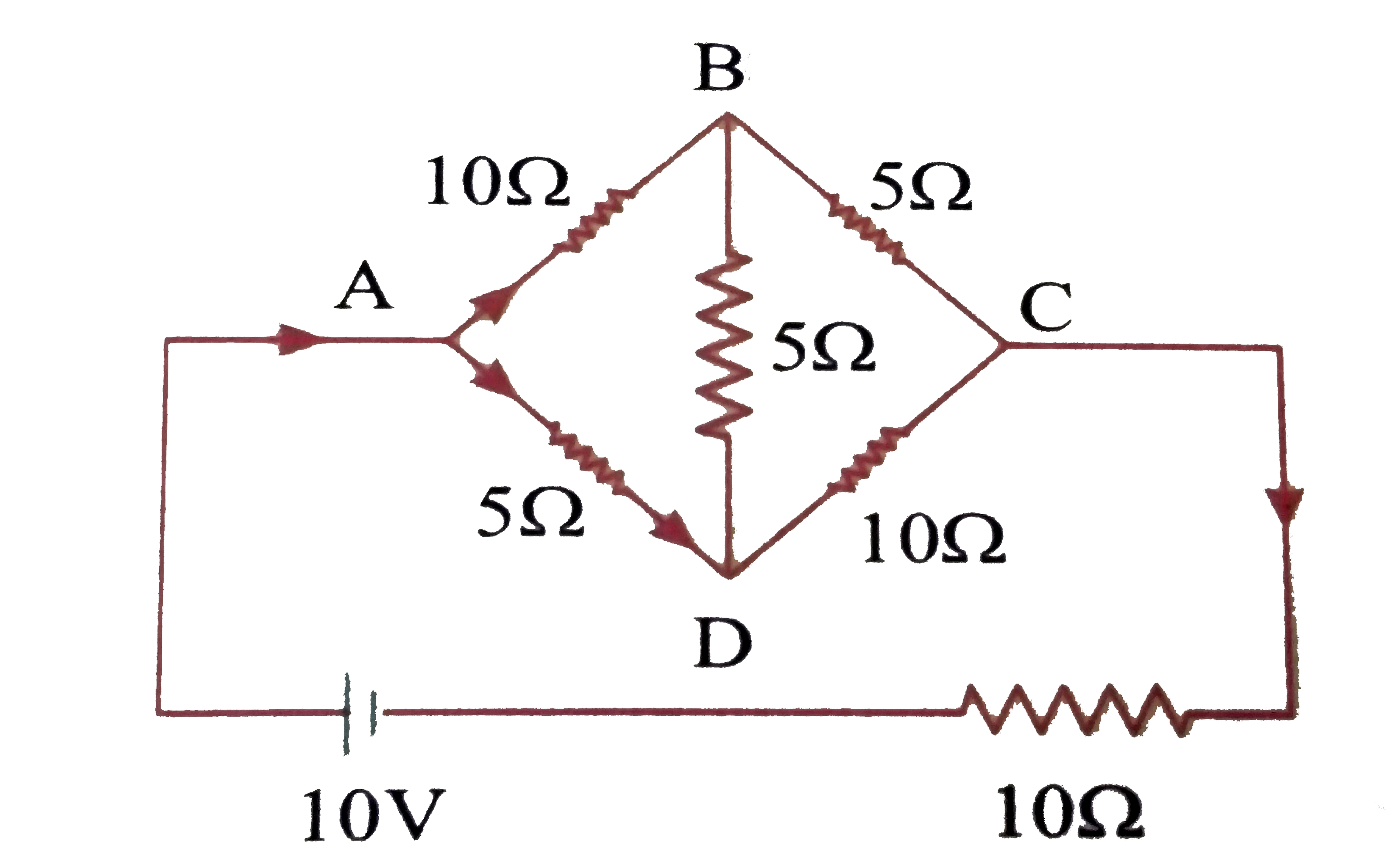
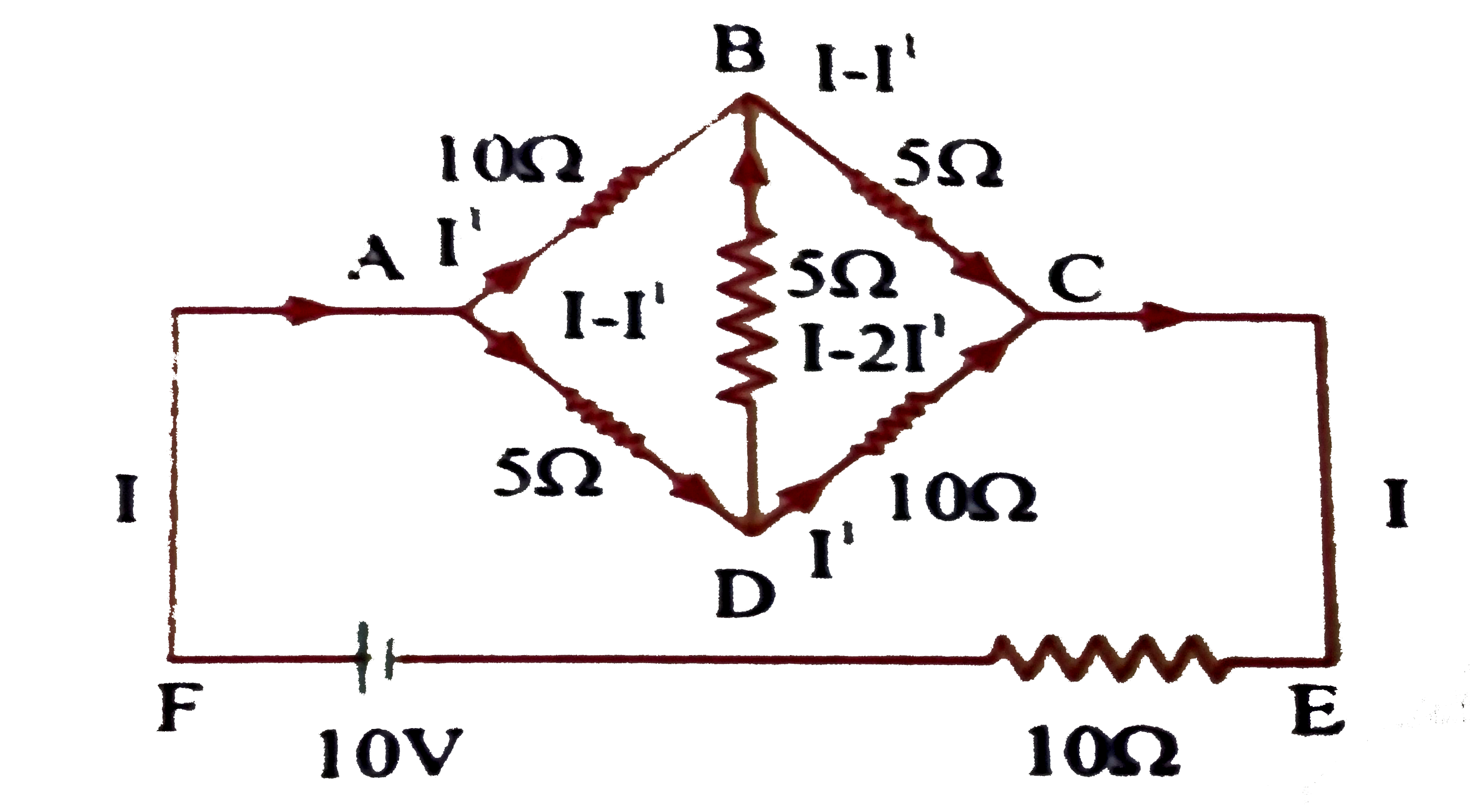
Determine the current in each branch of the network shown in fig.View SolutionDetermine the current in each branch of the network shown in Fig.
 View Solution
View SolutionDetermine the current in branches AB and BC of the network shown in Fig.
View SolutionDetermine the current in each branch of the network shown in Fig. 3.24
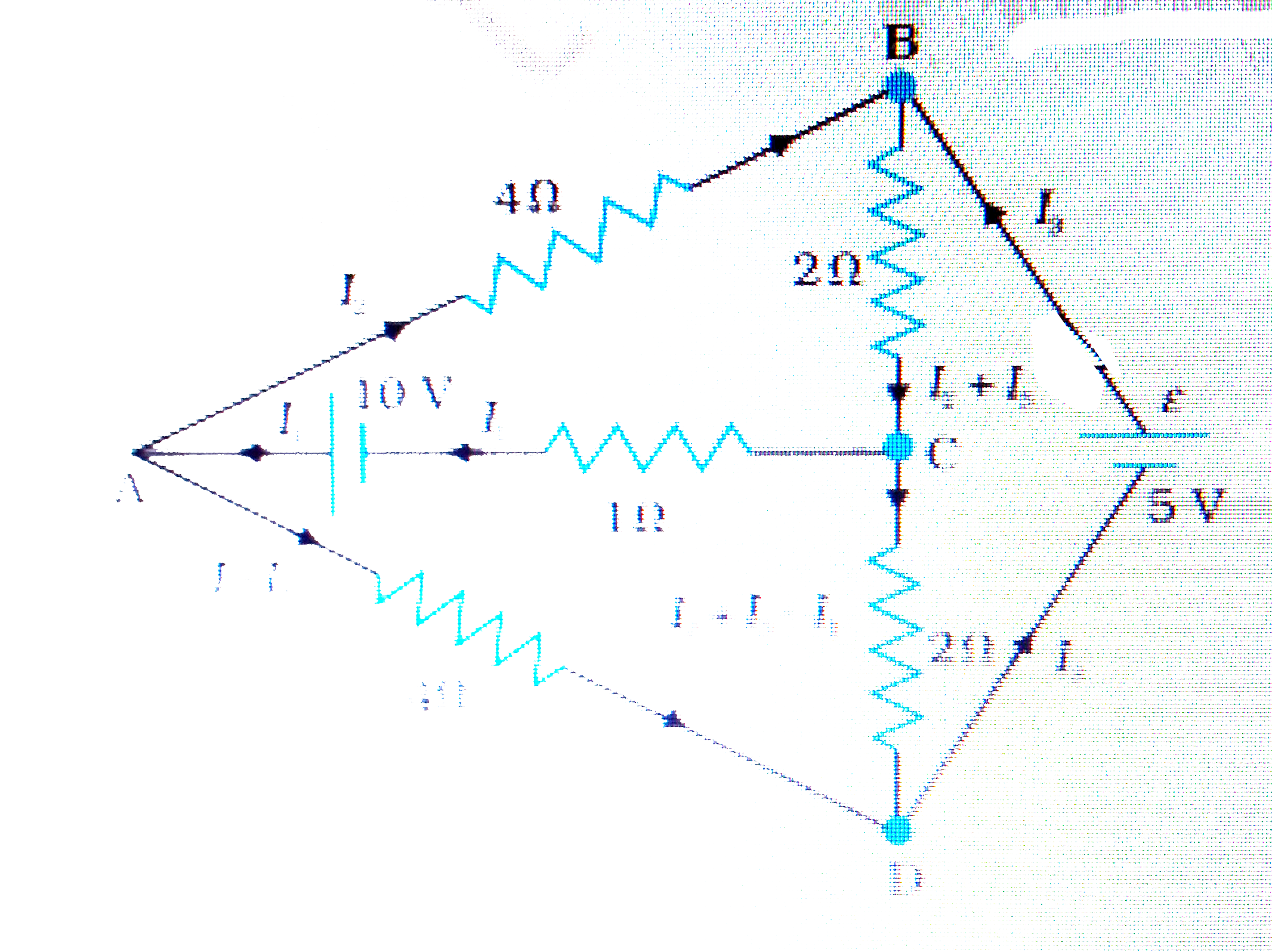 View Solution
View Solutionઆકૃતિમાં દર્શાવેલ નેટવર્કમાં દરેક શાખામાંથી વહેતો પ્રવાહ શોધો.
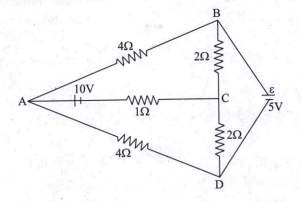 View Solution
View Solutionઆકૃત્તિમાં દર્શાવેલ નેટવર્ક માટે દરેક શાખમાંથી વહેતો પ્રવાહ શોધો.
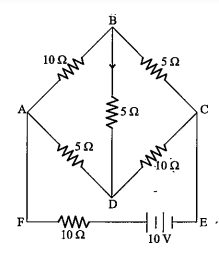 View Solution
View SolutionDetermine the currents through the resistance of the circuits shown in fig.
View SolutionFor the network shown in figure Determine the value of R and the current through it, if the current through the branch AO is zero.
View Solutionfor the network shown in figure, determine the value of R and the current through it, if the current through the branch AO is zero.
View SolutionDetermine the current in each branch of the network shown in
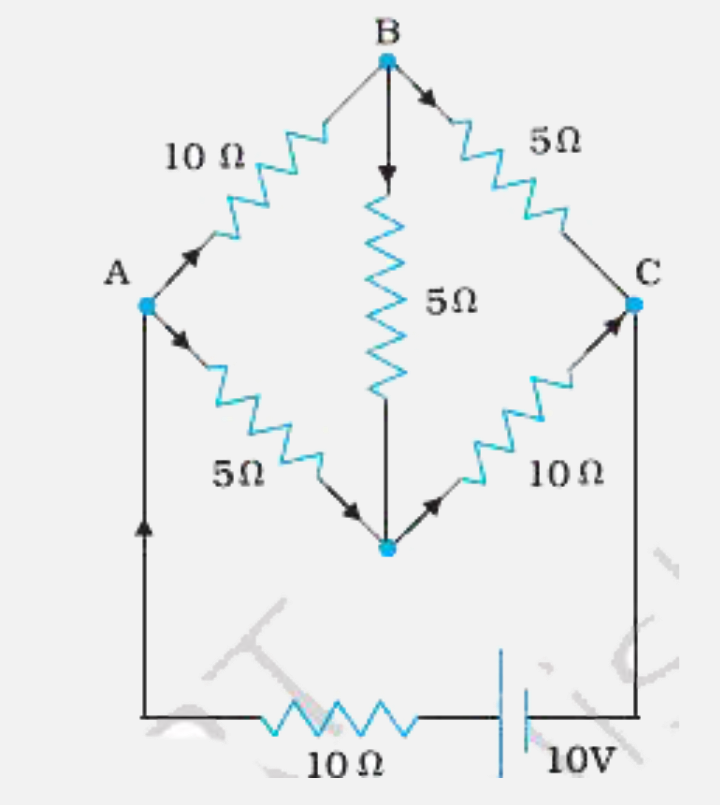 View Solution
View SolutionDetermine the current in each branch of the network shown in figure.
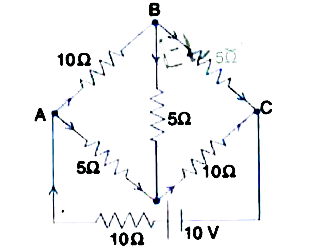 View Solution
View SolutionDetermine current in each branch of the network shown in figure
 View Solution
View SolutionDetermine the current in each branch of the network as shown in the figure
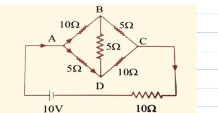 View Solution
View SolutionDetermine the current in each branch of the following network:
View Solution
