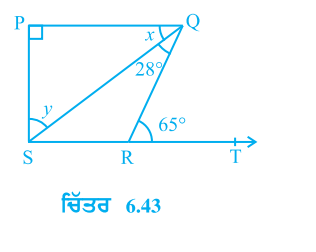
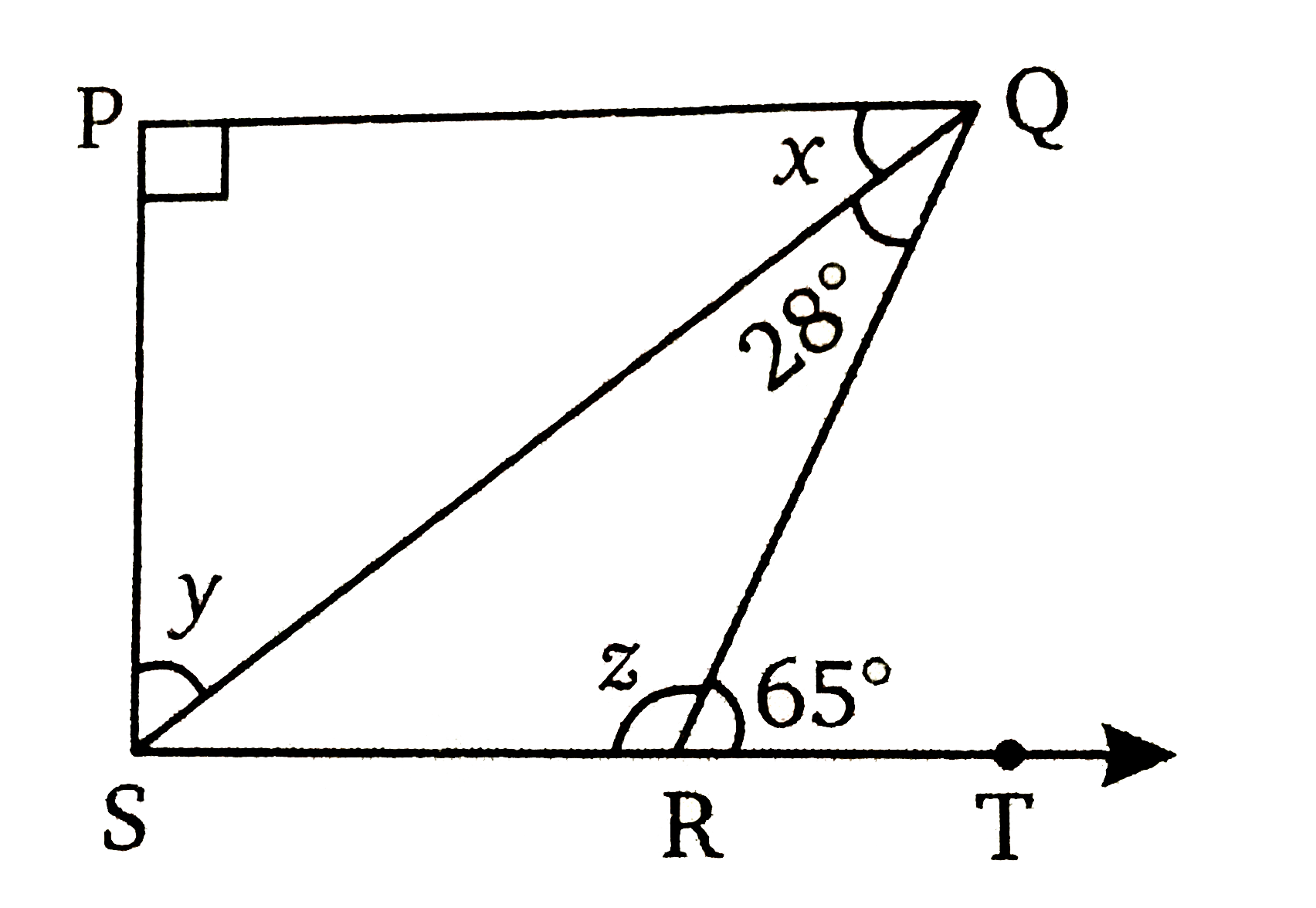
In the figure if PT ⊥ PS PQ || SR ∠SQR = 28° and ∠QRT = 65° then find the values of x and y.
In the given figure lines PQ ⊥ PS PQ || SR ∠SQR = 28° and ∠QRT = 65° ∠PQR = ∠QRT [Alternate angles] ⇒ x + 28° = 65° ⇒ x = 65° – 28° = 37° In ∆PQS ∠SPQ + ∠PQS + ∠QSP = 180° [Angle sum property of a triangle] ⇒ 90° + 37° + y = 180° [∵PQ ⊥ PS ∠PQS = x = 37° and ∠QSP = y ⇒ 127° + y = 180° ⇒ y = 180° – 127° = 53° Hence x = 37° and y = 53° Ans.
Related Solutions
ਚਿੱਤਰ 6.43 ਵਿਚ,ਜੇ , PQ || SR, ਅਤੇ ਹੈ, ਤਾਂ x ਅਤੇ y ਦੇ ਮੁੱਲ ਪਤਾ ਕਰੋ।
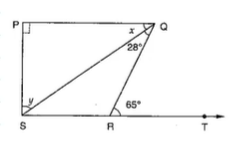
View SolutionIn the figure given below if PQ bot PS,PQ || SR, angle SQR=28^(@) and angle QRT=65^(@) find x and y
View SolutionIn Fig. 6.43, if , PQ || SR, and , then find the values of x and y.
View Solutionआकृति में, यदि और है, तो x और y के मान ज्ञात कीजिये ।

View SolutionIn figure, if and then find the values of and
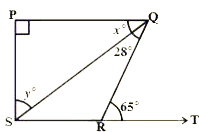
View SolutionIn the given figure if PQ PS, PQ || SR, and , then find the values of x and y
View Solutionआकृति में , यदि और है , तो x और y के मान ज्ञात कीजिए ।
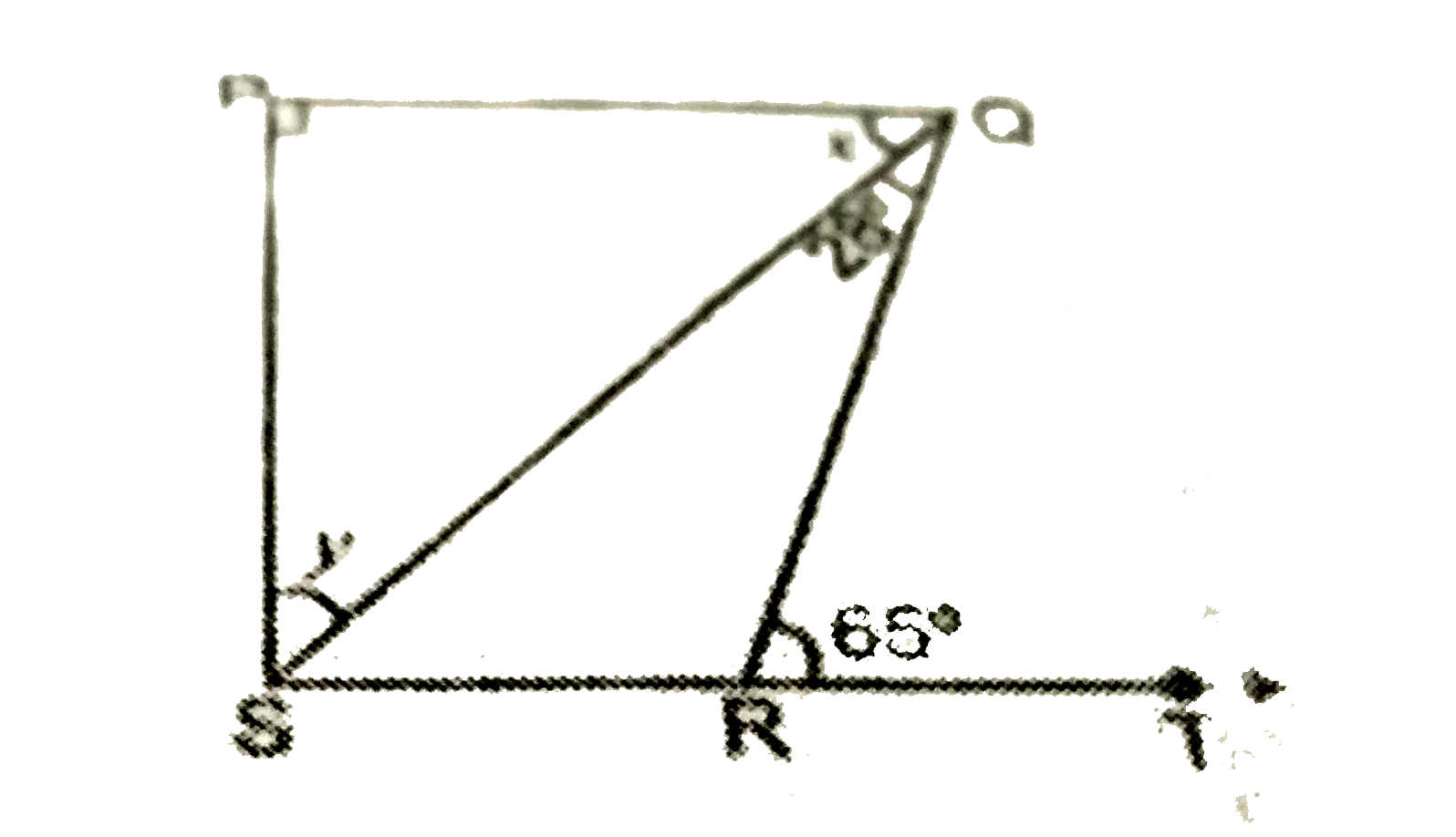
View SolutionIn the given figure if PQ PS, PQ || SR, and , then find the values of x and y
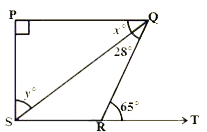
View SolutionIn the given figure if PQ PS, PQ || SR, and , then find the values of x and y
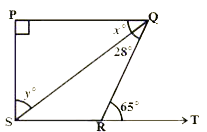
View SolutionIn the given figure if PQ PS, PQ || SR, and , then find the values of x and y
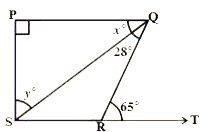
View SolutionIn figure, if , then find the values of x and y.
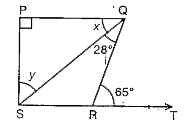
View SolutionIn the given figure QP bot PS, PQ ||SR, angleSQR=28^(@) And angleQRT=65^(@) If so, find the value of x and y.
View Solutionसंलग्न चित्र में यदि तथा हो तो x और y के मान ज्ञात कीजिए ।
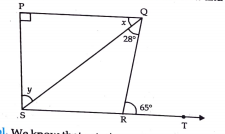
View SolutionIn fig. , and then find the values of x and y
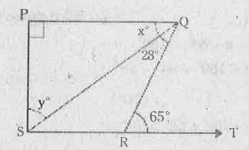
View Solutionఇచ్చిన పటంలో ,PQ |\| SR, మరియు అయిన x,y విలువలు కనుగొనుము.
View SolutionIn fig.
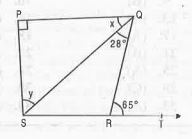 , if , , and then the values of x and y are :
, if , , and then the values of x and y are :
View Solutionआकृति में, यदि और है, तो x और y के मान ज्ञात कीजिय |
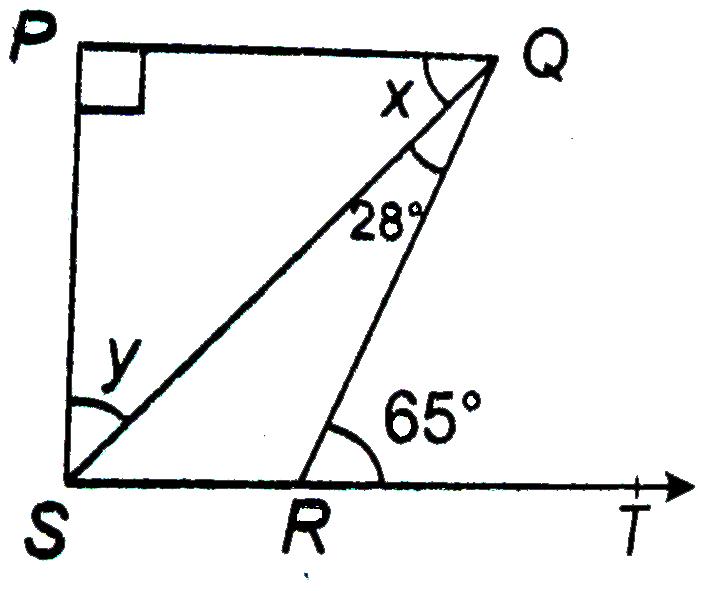
View SolutionIn the given figure, if and , then find the values of x and y.

View SolutionIn the given figure, if and , then find the values of x and y.

View SolutionIn the given figure, if and , then find the values of x and y.
View Solution