The diagram shows particles A and B of masses 0.2kg and mkg respectively connected by a light inextensible string which passes over a fixed smooth peg. The system is released from rest with B at a height of 0.25m above the floor. B descends hitting the floor 0.5s later. All resistance to motion may be ignored. a Find the acceleration of B as it descends. b Find the tension in the string while B is descending and find also the value of m. c When B hits the floor it comes to rest immediately and the string becomes slack. Find the length of time for which B remains at rest on the ground before being jerked into motion again.
Related Solutions
A block A of mass is held at rest on a smooth horizontal floor. A light frictionless, small pulley is fixed at a height of from the floor. A light inextensible string of length , connected with A passes over the pulley and another identical block B is hung from the string. Initial height of B is from the floor as shown in figure
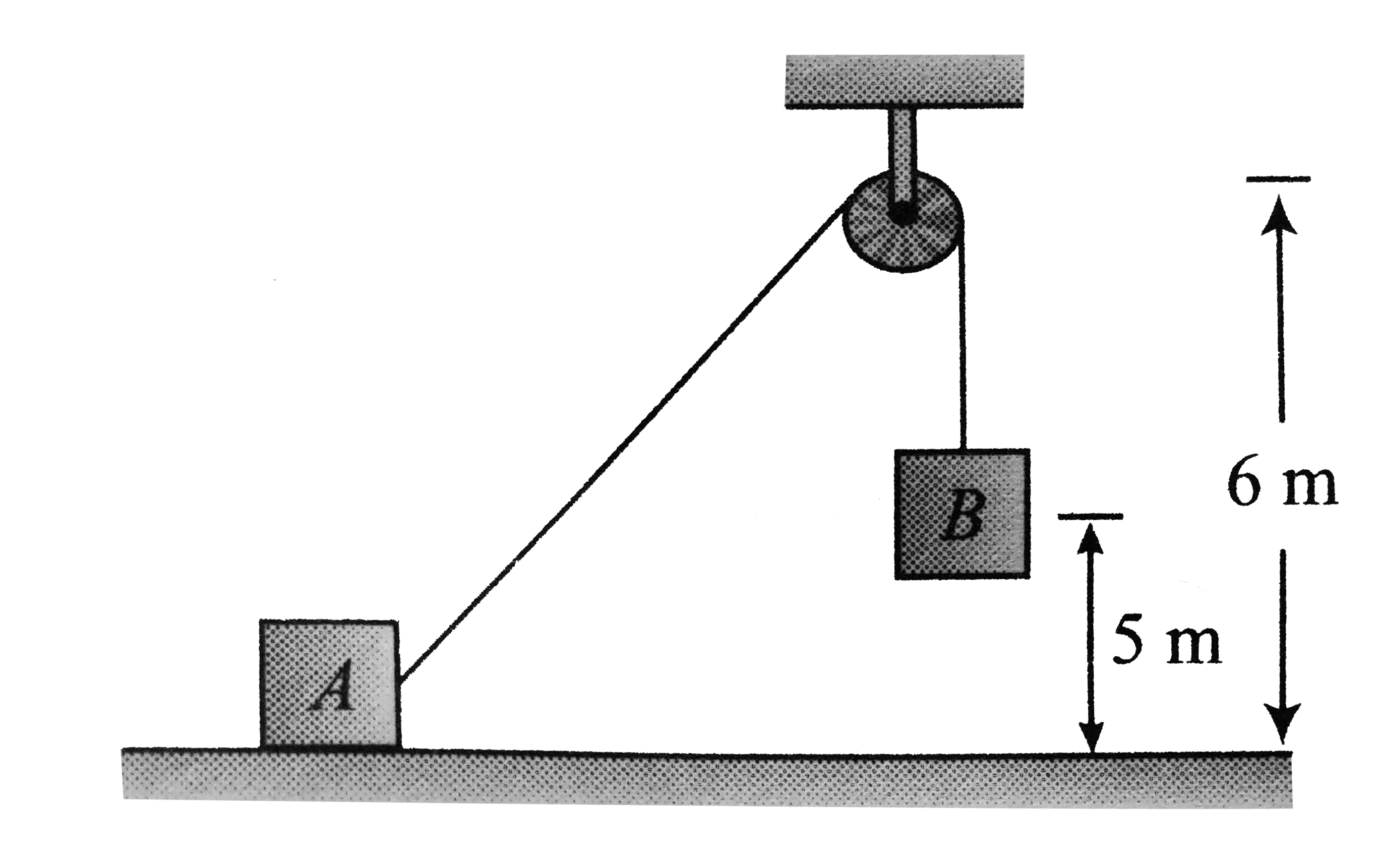
When the system is released from rest, B starts to move vertically downwards and A slides on the floor towards right.
a. If at an instant string makes an angle with horizontal, calculate relation between velocity of A and of B.
b. Calculate when B strikes the floor. ().View SolutionA block A of mass m is held at rest on a smooth horizontal floor. A light frictionless, small pulley is fixed at a height of 6m from the floor. A light inextensible string of length 16m , connected with A passes over the pulley and another identical block B is hung from the string. Initial height of B is 5m from the floor as shown in figure When the system is released from rest, B starts to move vertically downwards and A slides on the floor towards right. a. If at an instant string makes an angle theta with horizontal, calculate relation between velocity u of A and v of B. b. Calculate v when B strikes the floor. ( g=10ms^-2 ).
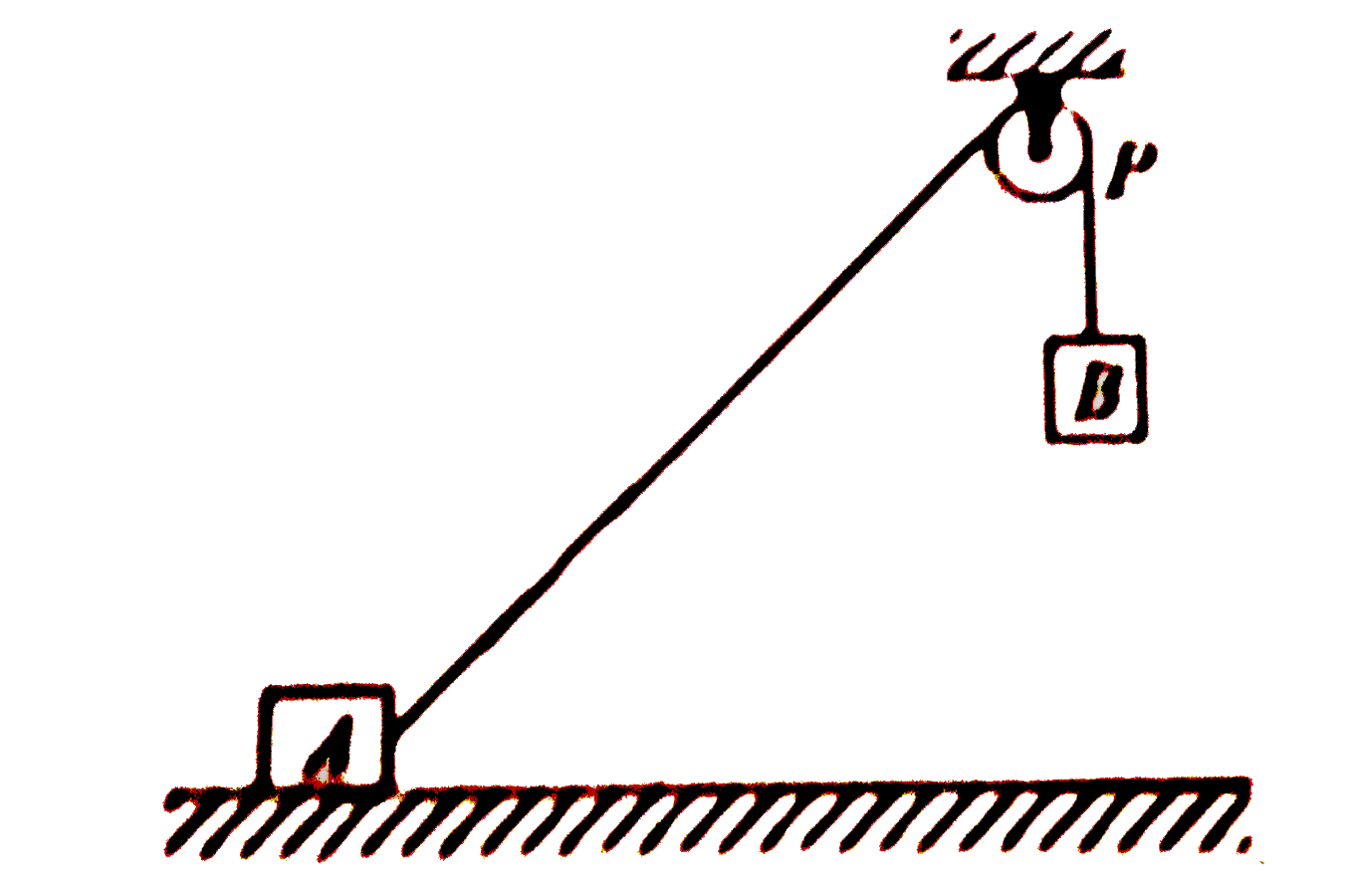
View SolutionA block of mass m is held at rest on a smooth horizontal floor. A light frictionless, small pulley is fixed at a height of 6m from the floor. A light inextensible string of length 16m, connected with A passes over the pulley and another identical block B is hung from the string initial height of B is 5m from the floor as shown in figure -3.130 When the system is released from rest, B starts to move vertically downwards and A slides on the floor towards right
(a) if at an instant string makes an angle with horizontal, calculate relation between velocity u of A and v of B
(b) Calculate c when B strikes the floor.
 View Solution
View SolutionIn the system shown in figure, all surfaces are smooth, pulley and strings are massless. Mass of both A and B are equal. The system is released from rest. (a) Find the veca_(A). veca_(B) immediately after the system is released. veca_(A) "and" veca_(B) are accelerations of block A and B respectively. (b) Find veca_(A) immediately after the system is released.
View SolutionTwo particles A and B of mass and m respectively are attached to the ends of a light inextensible string of length which passes over a small smooth peg at a height from an inelastic table. The system is released from rest with each particle at a height a from the table. Find -
(i) The speed of B when A strikes the table
(ii) The time that elapses begore A first hits the table.
(iii) The time for which A is resting on the table after the first collision & begore it is first jerked off.View SolutionTwo particles A and B of mass and m respectively are attached to the ends of a light inextensible string of length which passes over a small smooth peg at a height from an inelastic table. The system is released from rest with each particle at a height a from the table. Find -
(i) The speed of B when A strikes the table
(ii) The time that elapses begore A first hits the table.
(iii) The time for which A is resting on the table after the first collision & begore it is first jerked off.View SolutionA pillar having square cross section of side length L is fixed on a smooth floor. A particle of mass m is connected to a corner A of the pillar using an inextensible string of length 3.5 L. With the string just taut along the line BA, the particle is given a velocity v perpendicular to the string. The particle slides on the smooth floor and the string wraps around the pillar.
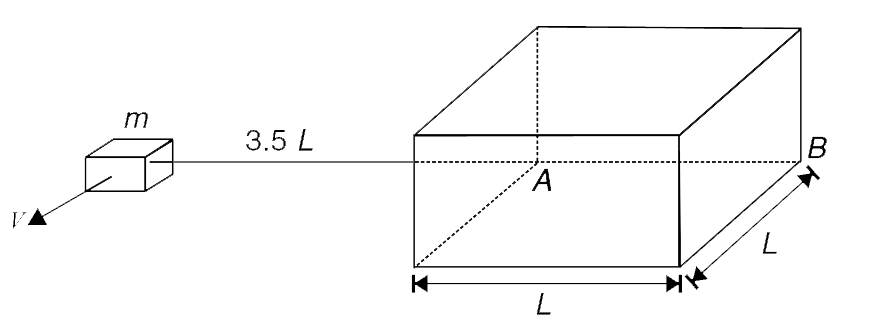
(a) Find the time in which the particle will hit the pillar. (b) Find the tension in the string just before the particle hits the pillar. Neglect any energy loss of the particle.View SolutionTwo masses are attached to the ends of a light inextensible string and the string is made to pass over the surface of a smooth fixed pulley. When the masses are released from rest, the acceleration of the system is
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionIn fig.block A and B are connected together by a string and placed on a smooth inclined plane. B is connected to C(which is suspended vertically) by another string which passes over a smooth pulley fixed to th plane. The mass A is m_(A)=1 kg and mass of B is m_(B)=2 kg . (a) If the system is at rest, find the mass of C. (b) If the mass of C is twice the mass calculate in (a) then find the acceleration of the system.
View SolutionIn fig.block A and B are connected together by a string and placed on a smooth inclined plane. B is connected to C(which is suspended vertically) by another string which passes over a smooth pulley fixed to th plane. The mass A is kg and mass of B is .
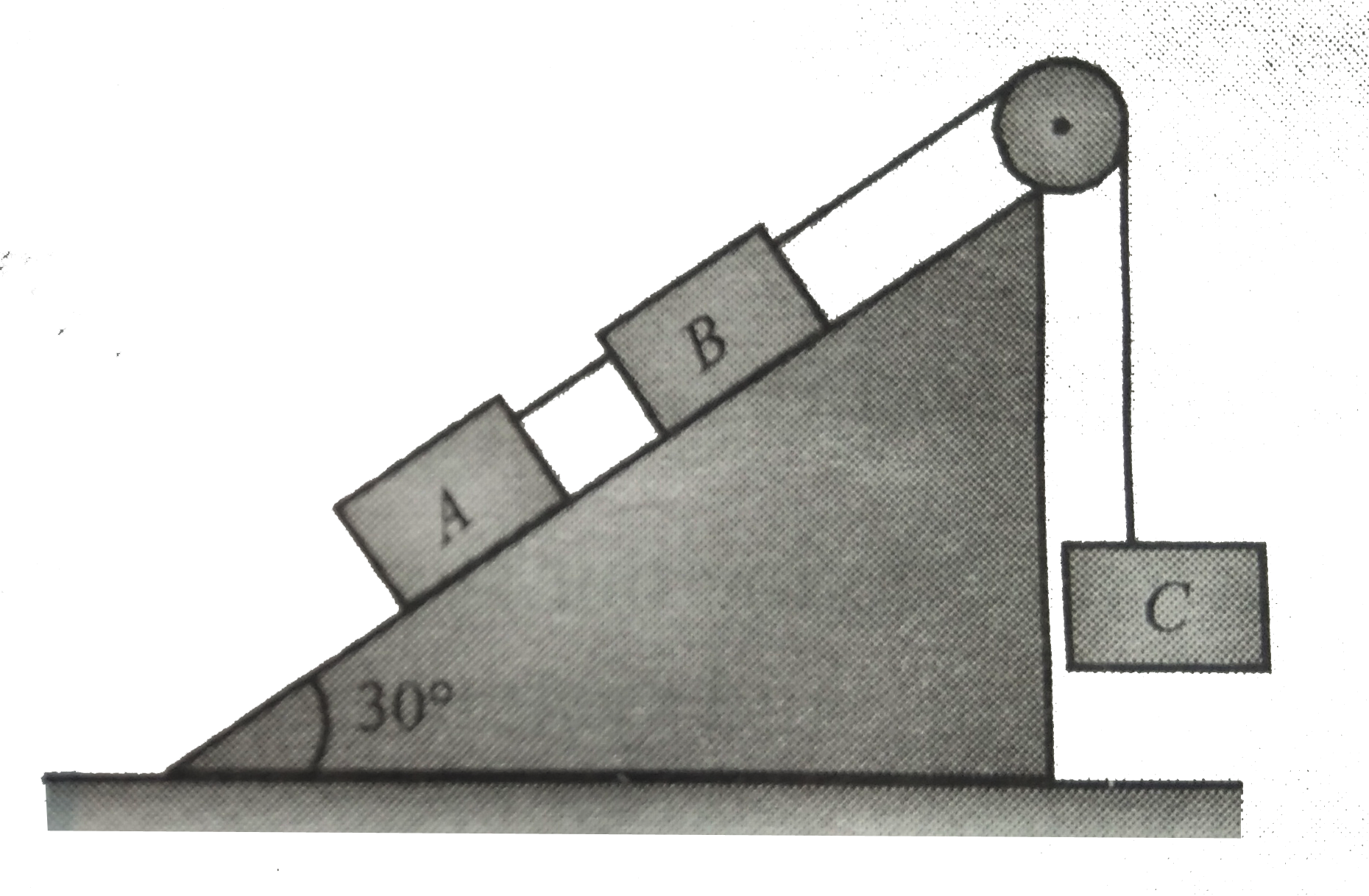
(a) If the system is at rest, find the mass of C.
(b) If the mass of C is twice the mass calculate in (a) then find the acceleration of the system.View SolutionTwo blocks A and B of equal masses are attached to a string passing over a smooth pulley fixed to a wedge as shown in figure. Find the magnitude of acceleration of centre of mass of the two blocks when they are released from rest. Neglect friction.
View SolutionTwo blocks A and B of equal masses are attached to a string passing over a smooth pulley fixed to a wedge as shown in figure. Find the magnitude of acceleration of centre of mass of the two blocks when they are released from rest. Neglect friction.
View SolutionTwo blocks A and B of equal masses are attached to a string passing over a smooth pulley fixed to a wedge as shown in figure. Find the magnitude of acceleration of centre of mass of the two blocks when they are released from rest. Neglect friction.
View SolutionTwo particles A and B having equal mass are interconnected by a light inextensible string that passes over a smooth pulley. One of the amsses is pulled downward by a constant force 'F' as shown in diagram, then find the acceleration of the centre of mass of the system (A+B) .
View SolutionTwo particles A and B having equal mass are interconnected by a light inextensible string that passes over a smooth pulley. One of the amsses is pulled downward by a constant force 'F' as shown in diagram, then find the acceleration of the centre of mass of the system (A+B) .
View Solution
