In ΔABC ∠B = ∠C and ∠A = 100° ; find ∠B.
∠A + ∠B + ∠C = 180°⇒ 100° + ∠B + ∠B = 180°⇒ 2∠B = 180° - 100°∠B = 80/2 °∠B = 40°∠C = ∠B = 40°
Related Solutions
If in ΔABC, ∠C = 90°, then sin (A + B) =
View SolutionConsider the matrix
Find a=1b=10c=100View SolutionFind all the three angles of the
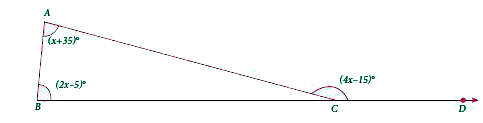 View Solution
View SolutionGiven that ΔABC∼ΔDEF,BC=3cm,EF=4cm ans the area of ΔABC is 54sq.cm.Then find the area of ΔDEF.
View SolutionIn figure ΔABC is circumscribing a circle. Find the length of BC
View SolutionExpress in the form of
Find the value ofView SolutionGiven positive angles (in degree) A,B and C with A+B+C=90^(@) .Find the value of x if 100 tan A=200tan B=300tan C=x
View Solutionif sum_(k+1)(k)/(k+1)*^(100)C_(k)=(a*2^(100)+b)/(c) where a,b,c in N then find the least value of a+b+c
View SolutionΔABC is isosceles with AB=AC=7cm and BC=9cm. The height AD from A to BC, is 6cm. Find the area of ΔABC. What will be the height from C to AB i.e., CE?
View SolutionFind the number of terms in the expansion of
View SolutionFind the number of terms in the expansion of
View SolutionIf , then find
View SolutionThe incircle of ΔABC touches the sides, AB, BC and AC in the point P, Q and R respectively. If AP=7cm, BC=13cm, find the perimeter of ΔABC.
View SolutionIf n(S) =700 ,n(A)=200,n(B)=300 , then find the value of
View SolutionIn a ΔABC, a semicircle is inscribed, whose diameter lies on the side c. Then find the radius of the semicircle.(Where Δ is the area of the triangle ABC)
View SolutionIn ΔABC are extended to respectively and . Prove that .
View Solutiona^(2) +b^(2)=100 And ab=48, (a+b)//(a-b) Find the value of.
View SolutionIn ΔABC and ΔDEF AB = DE, BC = EF and BC // EF. The vertices A, B and C are connected to D, E and F respectively AC = DF
View SolutionIn ΔABC and ΔDEF AB = DE, BC = EF and BC // EF. Show that the vertices A, B and C are ,ABC≅ΔDEF which are connected to D, E and F respectively.
View SolutionEvaluate the following: {:|(b+c,a,a),(b,c+a,b),(c,c,a+b)|
View Solution
