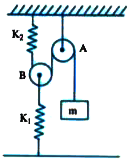
A block of mass m is attached to one end of a light inextensible string passing over a smooth light pulley B and under another smooth light pulley A as show in the figure. The other end of the string is fixed to a ceiling. A and B are held by springs of spring constant k1 and k2. Find angular frequency of small oscillations of the system.
Let F be the restoring force extra tension on block m when displaced by x from its equilibrium position.
Related Solutions
A block of mass 'm' is attached to one end of a light inextensible string passing over a smooth light Pulley B and under another smooth light Pulley A as shown is figure. The other end of a string is fixed to a ceilling . A and B are held by springs of spring constant K_1 and K_2 . Find the angular frequency of small oscillation of the system
View SolutionA block of mass is attached to one end of a light inextensible string passing over a smooth light pulley and under another smooth light pulley as shown in the figure. The other end of the string is fixed to a ceiling. and are held by spring of spring constants and . Find angular frequency of small oscillations of small oscillations of the system.

View SolutionA block of mass m is attached to one end of a light inextensible string passing over a smooth light pulley B and under another smooth light pulley A as shown in the figure. The other end of the string is fixed to a ceiling. A and B are held by spring of spring constants k_(1) and k_(2) . Find angular frequency of small oscillations of small oscillations of the system.
View SolutionA block of mass 'm' is tied to one end of string which passes over a smooth pulley 'A' and under a light smooth movable pulley B. The other end of the string is attached to lower end of a string constant . Find the period of small oscillations of mass 'm' about its equilibrium position.
View SolutionIn the shown figure mass of the pulley is m and radius 2R. A light concentric spool of radius R is rigidly attached with the pulley. Two blocks A and B having masses m & 4m respectively are attached with the pulley by means of light strings. Lower surface of the block B is attached to a spring of stiffness ka and block B rests on a smooth inclined plane inclined with horizontal. Other end of the spring is fixed to the ground. The block A is stretched a small distance and released then find the frequency of the oscillation of block B. (The strings do not slip on the pulley).
View SolutionIn the shown figure mass of the pulley is m and radius 2R. A light concentric spool of radius R is rigidly attached with the pulley. Two blocks A and B having masses m & 4m respectively are attached with the pulley by means of light strings. Lower surface of the block B is attached to a spring of stiffness ka and block B rests on a smooth inclined plane inclined with horizontal. Other end of the spring is fixed to the ground. The block A is stretched a small distance and released then find the frequency of the oscillation of block B. (The strings do not slip on the pulley).

View SolutionTwo blocks are connected by an inextensible light string, the string is passing over smooth, light pulley as shown. Find the acceleration of blocks and tension in the string, reaction in pulley.
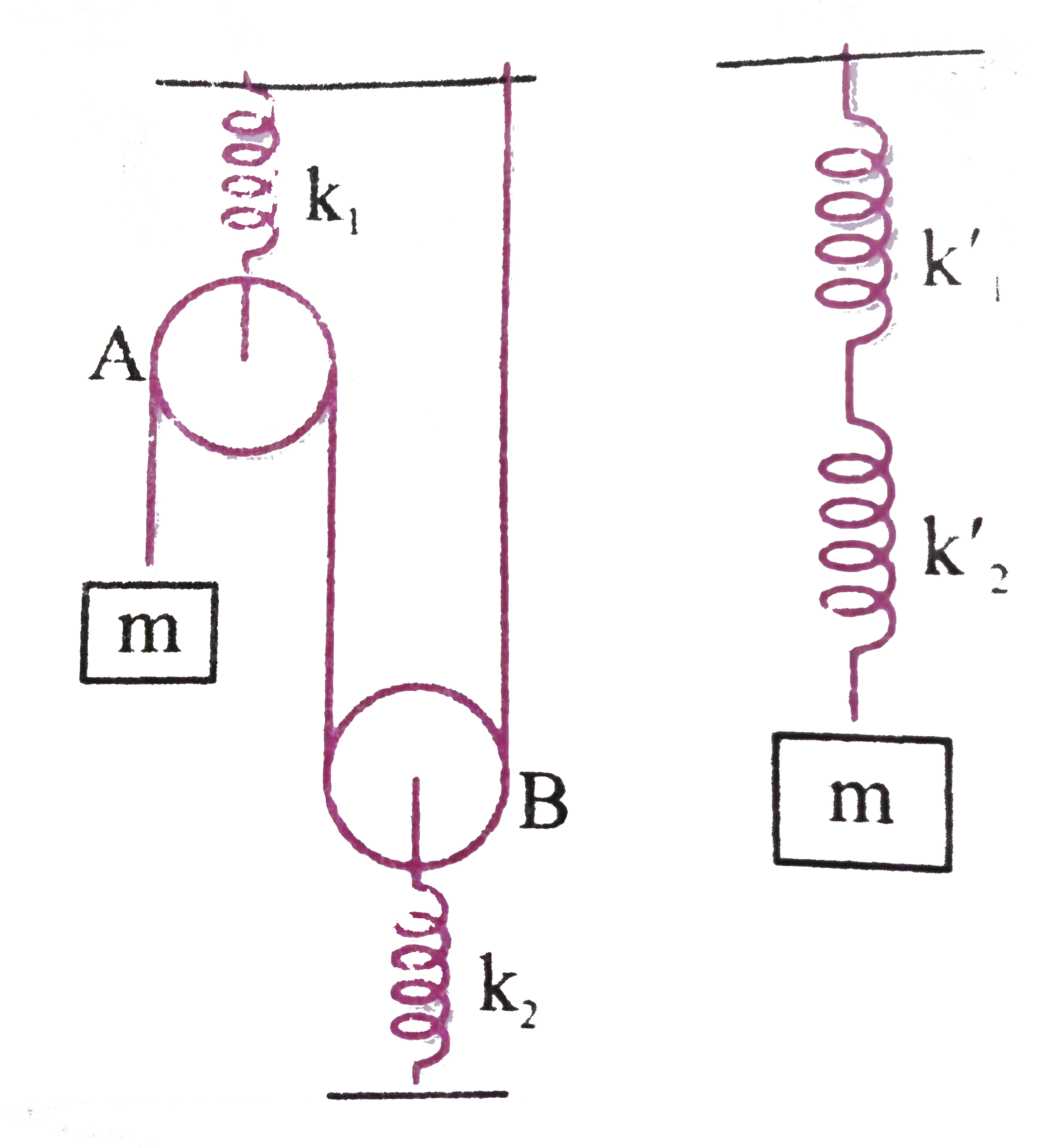
View SolutionA light pulley is suspended at the lower end of a spring of constant k_(1) , as shown in figure. An inextensible string passes over the pulley. At one end of string a mass m is suspended, the other end of the string is attached to another spring of constant k_(2) . The other ends of both the springs are attached to rigid supports, as shown. Neglecting masses of springs and any friction, find the time period of small oscillations of mass m about equilibrium position.
View SolutionA light pulley is suspended at the lower end of a spring of constant k_(1) , as shown in figure. An inextensible string passes over the pulley. At one end of string a mass m is suspended, the other end of the string is attached to another spring of constant k_(2) . The other ends of both the springs are attached to rigid supports, as shown. Neglecting masses of springs and any friction, find the time period of small oscillations of mass m about equilibrium position.
View SolutionA block of mass is attached to one end of a light intextensible string passing over a smooth light pulled and under another smooth light pulled as shown in Fig. The other end of a springs is fixed to a ceiling. and are held by spring of force constant and . Find the period of small oscillations of system.
View Solution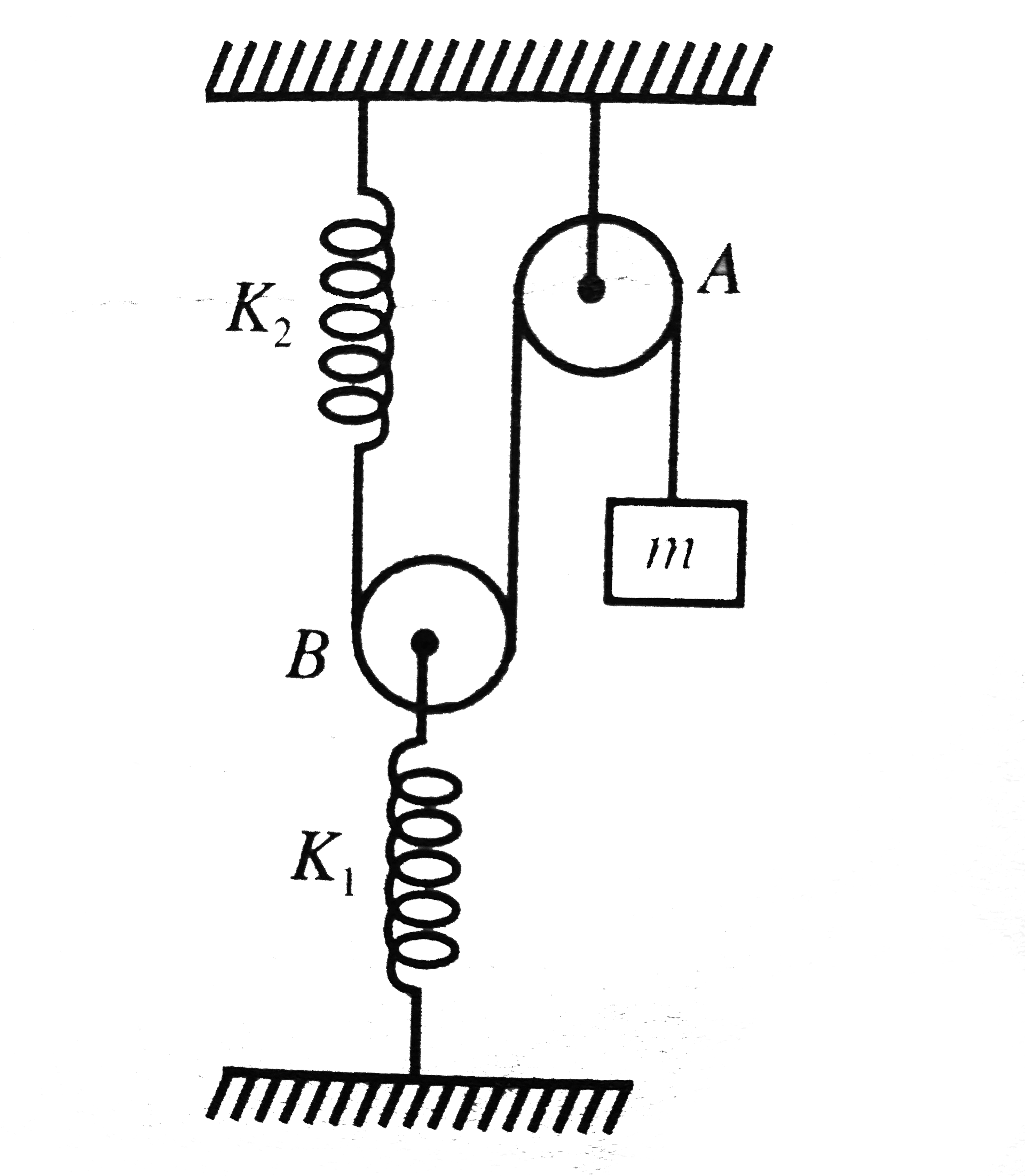
A block of mass is tied to one end of a spring which passes over a smooth fixed pulley and under a light smooth movable pulley . The other end of the string is attached to the lower end of a spring of spring constant . Find the period of small oscillation of mass about its equilibrium position (in second). (Take ,,)
View Solution
A block of mass is tied to one end of a spring which passes over a smooth fixed pulley and under a light smooth movable pulley . The other end of the string is attached to the lower end of a spring of spring constant . Find the period of small oscillation of mass about its equilibrium position (in second). (Take ,,)
View SolutionTwo masses are attached to the ends of a light inextensible string and the string is made to pass over the surface of a smooth fixed pulley. When the masses are released from rest, the acceleration of the system is
View SolutionThe masses (M+m) and (M-m) are attached to the ends of a light inextensible string and the string is made to pass over the surface of a smooth fixed pulley. When the masses are relased from rest the acceleraion of the system is .
View SolutionA uniform cubical block of mass M is attached to a pulley. One end of a string, passing over the pulley is fixed to a light pole while is fixed on the bigger block and its another end is attached to a block of mass m as shown in figure. The maximum value of the mass m so that the block does not topple is : (string and pulley are ideal)

View SolutionA uniform cubical block of mass M is attached to a pulley. One end of a string, passing over the pulley is fixed to a light pole while is fixed on the bigger block and its another end is attached to a block of mass m as shown in figure. The maximum value of the mass m so that the block does not topple is : (string and pulley are ideal)

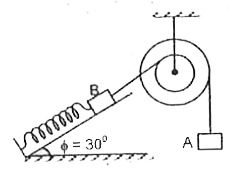
View SolutionA body of mass hangs from a smooth fixed pulley by the inextensible string fitted with the springs of stiffness and . The string passes over the smooth light pulley which is connected with another ideal spring of stiffness . Find the period of oscillation of the body.
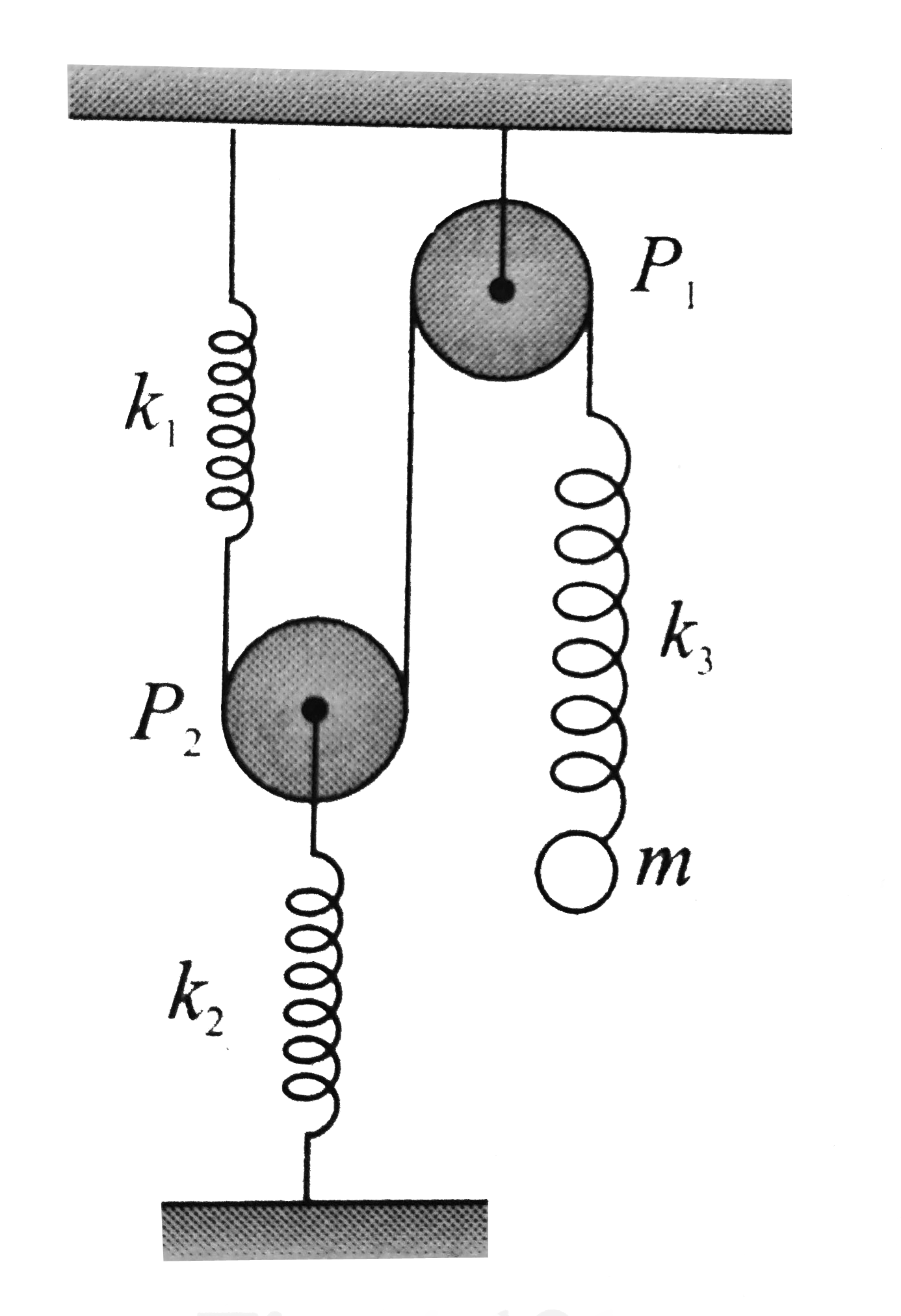
View SolutionA body of mass hangs from a smooth fixed pulley by the inextensible string fitted with the springs of stiffness and . The string passes over the smooth light pulley which is connected with another ideal spring of stiffness . Find the period of oscillation of the body.

View SolutionA particle of mass m is connected to another very heavy particle by means of a light inextensible string that passes over a smooth pulley. The reading of the spring balance is
View SolutionOne end of a string is attached to a 6kg mass on a smooth horizontal table. The string passes over the edge of the table and to its other end is attached a light smooth pulley.Over this pulley passes another string to the ends of which are attached masses of 4kg and 2kg respectively. Show that the 6kg mass moves with an acceleration of .
View Solution