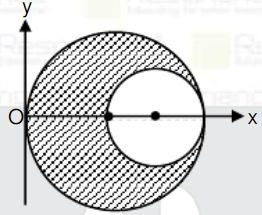
A circular disc of radius R has uniform thickness. A circular hole of diameter equal to the radius of the disc has been cut out from the disc as shown in figure the centre of mass of the remaining portions of the disc lies of the diameter of the disc at a distance x to the left of the centre of the original disc. the value of x is.a Rb R/2c R/4d R/6
Correct option d R/6Explanation:
Related Solutions
From a uniform circular disc of diameter D, a circular disc or hole of diameter and having its centre at a distance of from the centre of the disc is scopped out. Determine the centre of mass of the remaining portion.
View SolutionA uniform circular disc has a radius of 20cm. A circular disc of radius 10 cm is cut concentrically from the original disc. The shift in centre of mass is
View SolutionA uniform circular disc has a radius of 20cm. A circular disc of radius 10cm is cut concentrically from the original disc. The shift in centre of mass is
View SolutionA uniform circular disc has a radius of 20cm. A circular disc of radius 10cm is cut concentrically from the original disc. The shift in centre of mass is
View SolutionFrom a uniform circular disc of diameter d,a circular disc of diameter d//6 and having centre at a distance d//4 from the centre of the disc is scooped out. Determine the centre of mass of remaining portion.
View SolutionA circular hole is cut from a disc of radius 6 cm in such a way that the radius of the hole is 1 cm and the centre of 3 cm from the centre of the disc. The distance of the centre of mass of the remaining part from the centre of the original disc is
View SolutionA circular hole is cut from a disc of radius 6 cm in such a way that the radius of the hole is 1 cm and the centre of 3 cm from the centre of the disc. The distance of the centre of mass of the remaining part from the centre of the original disc is
View SolutionFigure shows a uniform disc of radius R , from which a hole of radius R/2 has been cut out from left of the centre and is placed on the right of the centre of the disc. Find the CM of the resulting disc.
View SolutionFigure shows a uniform disc of radius R , from which a hole of radius R/2 has been cut out from left of the centre and is placed on the right of the centre of the disc. Find the CM of the resulting disc.
View SolutionA circular disc of radius (R)/(4) is cut from a uniform circular disc of radius R. The centre of cut portion is at a distance of (R)/(2) from the centre of the disc from which it is removed. Calculate the centre of mass of remaining portion of the disc.
View SolutionA circular hole of raidus 1 cm is cut off from a disc of radius 6 cm. The centreof the hole is 3 cm from the centre of the disc. Then the distance of the centre of mass of the remaining disc from the centre of the disc is
View SolutionFrom a uniform disc of radius R, a circular disc of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of original disc. Locate the centre of gravity of the resultant flat body.
View SolutionGiven a uniform disc of mass M and radius R . A small disc of radius R//2 is cut from this disc in such a way that the distance between the centres of the two discs is R//2 . Find the moment of inertia of the remaining disc about a diameter of the original disc perpendicular to the line connecting the centres of the two discs
View SolutionGiven a uniform disc of mass M and radius R . A small disc of radius R//2 is cut from this disc in such a way that the distance between the centres of the two discs is R//2 . Find the moment of inertia of the remaining disc about a diameter of the original disc perpendicular to the line connecting the centres of the two discs
View SolutionA disc of radius is cut out from a uniform disc of radius a as shown in figure . Find the X Coordinate of centre of mass of remaining portion
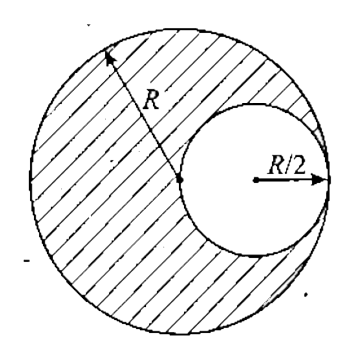
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs touch. The centre of mass of the new disc is at a distance aR from the centre of the bigger disc. The value of a is
View SolutionThe distance of center of mass from the center of a uniform disc of radius R. if a circular plate is removed from the disc as shown in figure will be (C is centre of complete disc)
View SolutionA disc of radius R is cut from a larger disc of radius 2R in such a way that the edge of the hole touches of edge of the disc. The centre of mass of the remaining portion is at
View SolutionA disc of radius R is cut from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. The centre of mass of the remaining portion is at
View SolutionFigure-4.11 shows a circular a disc of radius R from which a small disc is cut such that the periphery of the small disc touch the large disc and whose radius is . Find the centre of mass of the remaining part of the disc.
View Solution