यदि x+y=2,तो फलन xy2का उच्चिष्ट मान ज्ञात कीजिए ।
माना P=xy2
=x(2−x)2 [∵x+y=2,(दियाहै)]
dpdx=x[2(2−x)(−1)]+(2−x)2(1)
=−2x(2−x)+(2−x)2
=(2−x)(−2x+2−x)
=(2−x)(2−3x)
P के उच्छिष्ट या निम्निष्ट मान के लिए dPdx=0
∴ (2−x(2−3x)=0
x=2,23
अब d2Pdx2=(2−x)(−3)+(2−3x)(−1) =−6+3x−2+3x x=2,23
अब x =2 के लिए,
d2Pdx2=12−8
=4
=धनात्मक राशि
∴x=2पर P का मान निनिष्ट है। ltbRgt अब x=23 के लिए, d2Pdx2=6×23−8
=4−8
=−4
" " ऋणात्मक राशि
∴x=23 पर P का मान उच्छिष्ट है।
फलन का उच्छिष्ट मान =23(1−23)2
=13(13)2
13×19=127
Topper's Solved these Questions
- अवकलन के अनुप्रयोग BOOK - MANOHAR RAYCHAPTER - अवकलन के अनुप्रयोग EXERCISE - प्रश्नावली 6(A)
23 Videos
- अवकलन के अनुप्रयोग BOOK - MANOHAR RAYCHAPTER - अवकलन के अनुप्रयोग EXERCISE - प्रश्नावली 6(B)
23 Videos
- अवकल समीकरण BOOK - MANOHAR RAYCHAPTER - अवकल समीकरण EXERCISE - प्रश्नावली 9(F)
25 Videos
- आव्यूह BOOK - MANOHAR RAYCHAPTER - आव्यूह EXERCISE - प्रश्नावली 3 (E)
34 Videos
Similar Questions
किसी क्षण t पर एक कण की स्थिति x=4t2−15t+20मीटर से व्यक्त की गयी है।इससे शून्य समय पर कण की स्थति,वेग और त्वरण ज्ञात कीजिए।
View Solutionदिखाइए की निम्नलिखित फलन R पर वर्धमान है।:
(i)f(x)=3x+1
(ii)f(x)=x3−6x2+12x−16
(iii)f(x)=x3−3x2+4x
(iv)f(x)=x+cosx
View Solutionनिम्नलिखित अवकल समीकरणों को हल कीजिए
2x2dy=(x2+y2)dx
View Solutionआव्यूह [0110] का क्रम होगा
View Solutionयदि 2x+y=1 तो फलन x2y का उच्चिष्ठ मान ज्ञात कीजिए
View Solutionयदि (cosx)y =(sin y) ^(x) , तो(dy)/(dx) का मान ज्ञात कीजिए|
View Solutionयदि 3+5r+7r2+……..∞=449 हो ,तो r का मान ज्ञात कीजिए ।
View Solutionआकृति के अनुसार यदि ∠BAC=80∘ हो तो ∠BCP का मान ज्ञात कीजिए।
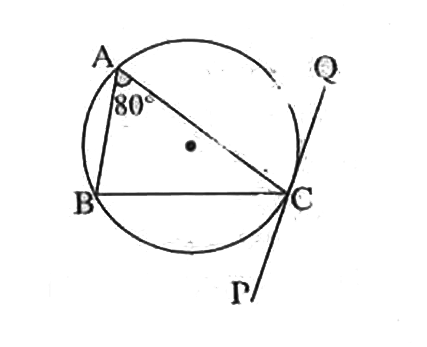
View Solutionयदि f(x)=√x−2 तो ज्ञात कीजिए : fof
View Solutionयदि f(x)=√x−2 तो ज्ञात कीजिए : fofof
View Solutionफलन 3 sin x +4 cos x का उच्चिष्ठ मान ज्ञात कीजिए।
View Solutionयदि xy = 4 तथा x> 0 तो x का वह मान ज्ञात कीजिए जिसके लिए x + y न्यूनतम है।
View Solutionयदि y=1−cos2x1+cos2x है तो dydx का मान ज्ञात कीजिए।
View Solutionनिम्नलिखित फलनों के उच्चतम मान तथा निम्नतम मान, यदि कोई हो, तो ज्ञात कीजिए -
y=(2x−1)2+3
View Solutionनिम्नलिखित फलनों के उच्चतम मान तथा निम्नतम मान, यदि कोई हो, तो ज्ञात कीजिए -
y=−(x−1)2+10
View Solutionफलन y=f(x) के लिए यदि dydx=6(x−2)(x−3) है तो के ty अधिकतम मान के लिए का x मान ज्ञात कीजिये
View Solution
