A rectangular rigid fixed block has a long horizontal edge. A solid homogeneous cylinder of radus R is placed horizontally at rest its length parallel to the edge such that the exis of the cylinder and the endg of the block are in the same vertical plane as shown in the figure below. Ther is sufficinet friction present at the edge s that a very small displacement causes the cylinder to roll off the edge without slipping. Determine:
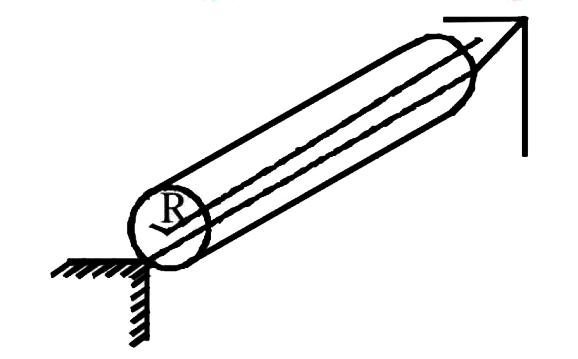
(a) the angle through which the cylinder rotates before it leaves contact with the edge,
(b) the speed of the centre of mass of the cylinder before leaving contact with the edge, and
(c) the ratio of the translational to rotational kinetic energy of the cylinder when its centre of mass is in horizontal line with the edge.
The correct Answer is:A, B, C, D
(a) Let the original position of centre of mass of the
cylinder be O. While rolling down off the edge, let the cylinder
be at such a position that its centre of mass is at a position
. As the cylinder is rolling the c.m. rotates
in a circular path. The centripetal force required for the
circular motion is given by the equation.
Where N is the normal reaction
and m is mass of cylinder.
The conditon for the cylinder
leaving the edge is N = 0
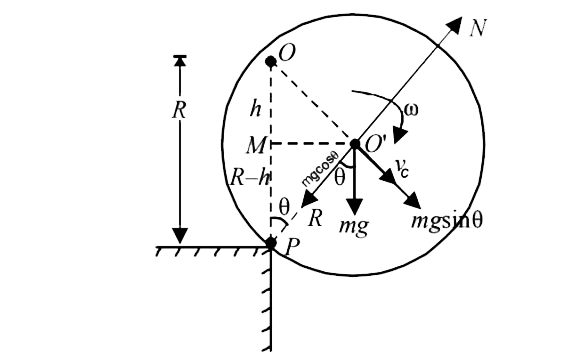
Applying energy conservation from O ot O.
Loss of potential energy of cylinder
=Gain in translation K.E.+Gain in rotational K.E.
Where i is the momentu of inertia of the cylinder about O, its
axis of rotation is the angular speed is the velocity of
centre of mass.
Also for rolling,
From (ii), (iii) and (iv), we get
in
From (i) and (v) we get
(b) From (v) speed of C.M. of cylinder before leaving
contact with edge.
(c ) Before the cylinder's c.m. reaches the horizontal line of
the edge, it leaves contact with the edge as
Therefore the rotational K.E., which the cylinder gains at
the time of leaving contact with the edge remains the same
in its further motion. Thereafter the cylinder gains
translational K.E.
Again applying energy conservation from O to the point
where c.m. is in horizontal line with edge
Also, Rotational K.e. = (1)/(2) Iomega^2 = (mgR)/(7):. (Translational K.E.)/(Rotational K.E.) = 6
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - ROTATIONAL MOTIONEXERCISE - MCQs with one correct answer
1 Videos
- MOTIONBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOTIONEXERCISE - JEE Main And Advanced
63 Videos
- SIMPLE HARMONIC MOTIONBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Main And Advanced
69 Videos
Similar Questions
A rectangular rigid fixed block has a long horizontal edge. A solid homogeneous cylinder of radus R is placed horizontally at rest its length parallel to the edge such that the exis of the cylinder and the endg of the block are in the same vertical plane as shown in the figure below. Ther is sufficinet friction present at the edge s that a very small displacement causes the cylinder to roll off the edge without slipping. Determine:

(a) the angle θc through which the cylinder rotates before it leaves contact with the edge,
(b) the speed of the centre of mass of the cylinder before leaving contact with the edge, and
(c) the ratio of the translational to rotational kinetic energy of the cylinder when its centre of mass is in horizontal line with the edge.
View SolutionTotal number of edges a cylinder has
View Solution
Knowledge Check
A clockwise torque of 6N−m is applied to the circular cylinder as shown in the figure. There is no friction between the cylinder and the block.
 AThe cylinder will be slipping but the system does not move forwardBThe system cannot move forward for any torque applied to the cylinderCThe acceleration of the system will be 1m/s2 forwardDThe angular acceleration of the cylinder is 10rads−2
AThe cylinder will be slipping but the system does not move forwardBThe system cannot move forward for any torque applied to the cylinderCThe acceleration of the system will be 1m/s2 forwardDThe angular acceleration of the cylinder is 10rads−2A hollow cylinder of length L and radius R having surface area A is placed horizontally with its axis parallel to an external field E. The electric flux through the surface of the cylinder is
AZeroB2πRLEC2πR2EDE . AA hollow cylinder of length L and radius R having surface area A is placed horizontally with its axis parallel to an external field E. The electric flux through the surface of the cylinder is
AZeroB2πRLEC2πR2EDE . A
Show that a nonhomogeneous cylinder on a horizontal plane has a position of stable equilibrium when its centre of gravity is vertically below the axis O of the cylinder.
View SolutionAB is a non uniform plank of length L=4R with its centre of mass at C such that AC=R. It is placed on a step with its one end A supported by a cylinder of radius R as shown in figure. The centre of mass of the plank is just outside the edge of the step. The cylinder is slowly rolled on the lower step such that there is no slipping at any of its contacts. Calculate the distance through which the centre of the cylinder moves before the plank loses contact with the horizontal surface of the upper step.
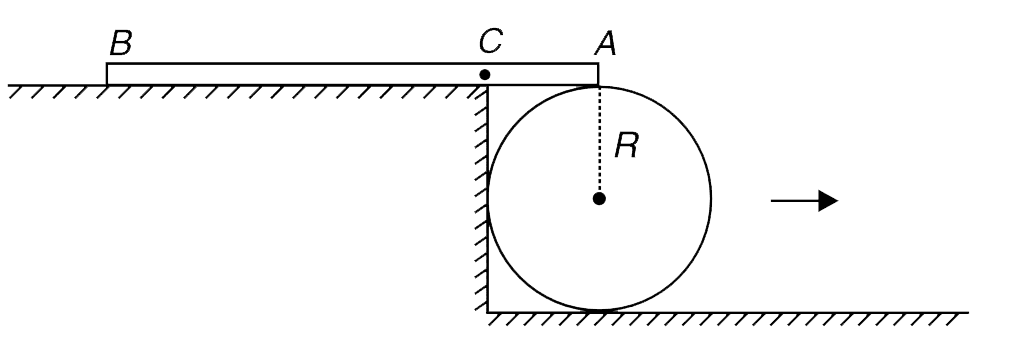
View Solution
A cylinder of mass M and radius R is resting on two corner edges A and B as shown in figure. The normal reaction at the edges A and B are: (Neglect friction)
View SolutionA force F is applied horizontally on a cylinder in the line of centre as shown in the figure. The cylinder is on a rough surface of coefficient of fricition μ. The direction of the friction force acting on the cylinder will be
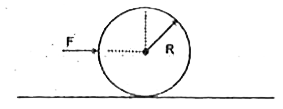
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View Solution
