A large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
(a) L√2π
(b) 2πL
(c) L
(d) L2π
The correct Answer is:A
To solve the problem, we need to use Torricelli's theorem, which states that the speed of efflux of a fluid under the force of gravity through an orifice is given by the equation:
v=√2gh
where v is the velocity of the fluid, g is the acceleration due to gravity, and h is the height of the fluid above the hole.
Step 1: Calculate the velocity of water flowing out of the square hole
For the square hole at depth y:
- The area As of the square hole is given by:
As=L2
- The height of water above the square hole is h=y.
- The velocity vs of water flowing out of the square hole is:
vs=√2gy
Step 2: Calculate the velocity of water flowing out of the circular hole
For the circular hole at depth 4y:
- The area Ac of the circular hole is given by:
Ac=πR2
- The height of water above the circular hole is h=4y.
- The velocity vc of water flowing out of the circular hole is:
vc=√2g(4y)=√8gy
Step 3: Set the flow rates equal
The flow rate Q through each hole can be expressed as:
Qs=Asvs
Qc=Acvc
Since the quantities of water flowing out per second from both holes are the same, we have:
Qs=Qc
Substituting the expressions for Qs and Qc:
L2⋅√2gy=πR2⋅√8gy
Step 4: Simplify the equation
We can cancel √gy from both sides (assuming y≠0):
L2⋅√2=πR2⋅√8
Rearranging gives:
L2⋅√2=πR2⋅2√2
Dividing both sides by √2:
L2=2πR2
Step 5: Solve for R
Now, we can solve for R:
R2=L22π
R=√L22π=L√2π
Thus, the radius R is equal to:
R=L√2π
Final Answer:
R=L√2π
---
For the square hole at depth y:
- The area As of the square hole is given by:
As=L2
- The height of water above the square hole is h=y.
- The velocity vs of water flowing out of the square hole is:
vs=√2gy
For the circular hole at depth 4y:
- The area Ac of the circular hole is given by:
Ac=πR2
- The height of water above the circular hole is h=4y.
- The velocity vc of water flowing out of the circular hole is:
vc=√2g(4y)=√8gy
The flow rate Q through each hole can be expressed as:
Qs=Asvs
Qc=Acvc
Qs=Qc
L2⋅√2gy=πR2⋅√8gy
We can cancel √gy from both sides (assuming y≠0):
L2⋅√2=πR2⋅√8
L2⋅√2=πR2⋅2√2
L2=2πR2
Now, we can solve for R:
R2=L22π
R=√L22π=L√2π
R=L√2π
R=L√2π
Topper's Solved these Questions
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MECHANICAL PROPERTIES OF MATTER AND FLUIDSEXERCISE - Subjective Problems1videos
MECHANICAL PROPERTIES OF MATTER AND FLUIDS
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - LAWS OF MOTIONEXERCISE - JEE Main And Advanced79videos
LAWS OF MOTION
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOMENTUM & IMPULSEEXERCISE - JEE Main And Advanced30videos
MOMENTUM & IMPULSE
Similar Questions
A large open tank has two holes in the wall. One is a square hole of side L at a depth h from the top and the other is a circular hole of radius r at a depth 4h from the top. Whwn the tank is completely filled with water, the quantity of water flowing out per second from both the holes are the same. What is the value of r?
View SolutionA vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '3h' from the top respectively. Hole 'A' is a square of side 'L' and 'B' is circle of radius 'r'. The water flowing out per second from both the holes is same. Then 'L' is equal to
View Solution
Knowledge Check
- Question 1 - Select One
A large open tank has two holes in the wall. One is a square hole of side L at a depth y from the top and the other is a circular hole of radius R at a depth 4y from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then, R is equal to
AL√2πB2πLCL√2πDL2π - Question 2 - Select One
A large open tank has two holes in its wall. Ine is a square hole of side a at a depath of x from the top and the other is a cirular hle of radius r at a depth 4x from the top. When the tank is completely filled with water, the quantities of water flowing out per second from both holes are the same. Then r is equal to
A2πaBaCa2πDa√2π - Question 3 - Select One
A large open tank has two holes in the wall. The top hole is a square hole of side L at a depth y from the surface of water. The bottom hole is a circular hole of radius R at a depth 4y from surface of water . If R=L/2√π, find the correct graph. V1 and V2 are the velocities in top and bottom holes. Area of the square & curcular holes are a1 and a2
A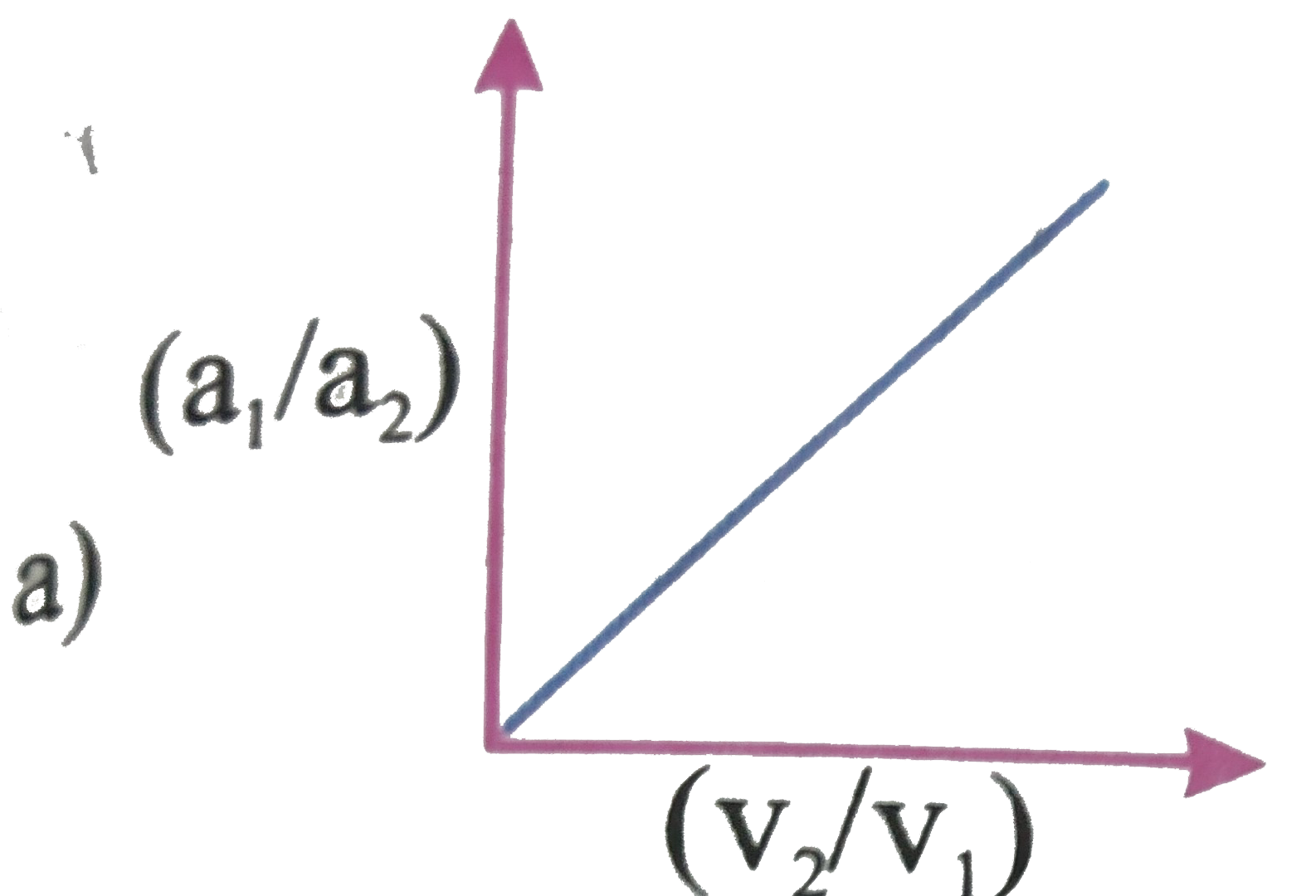 B
B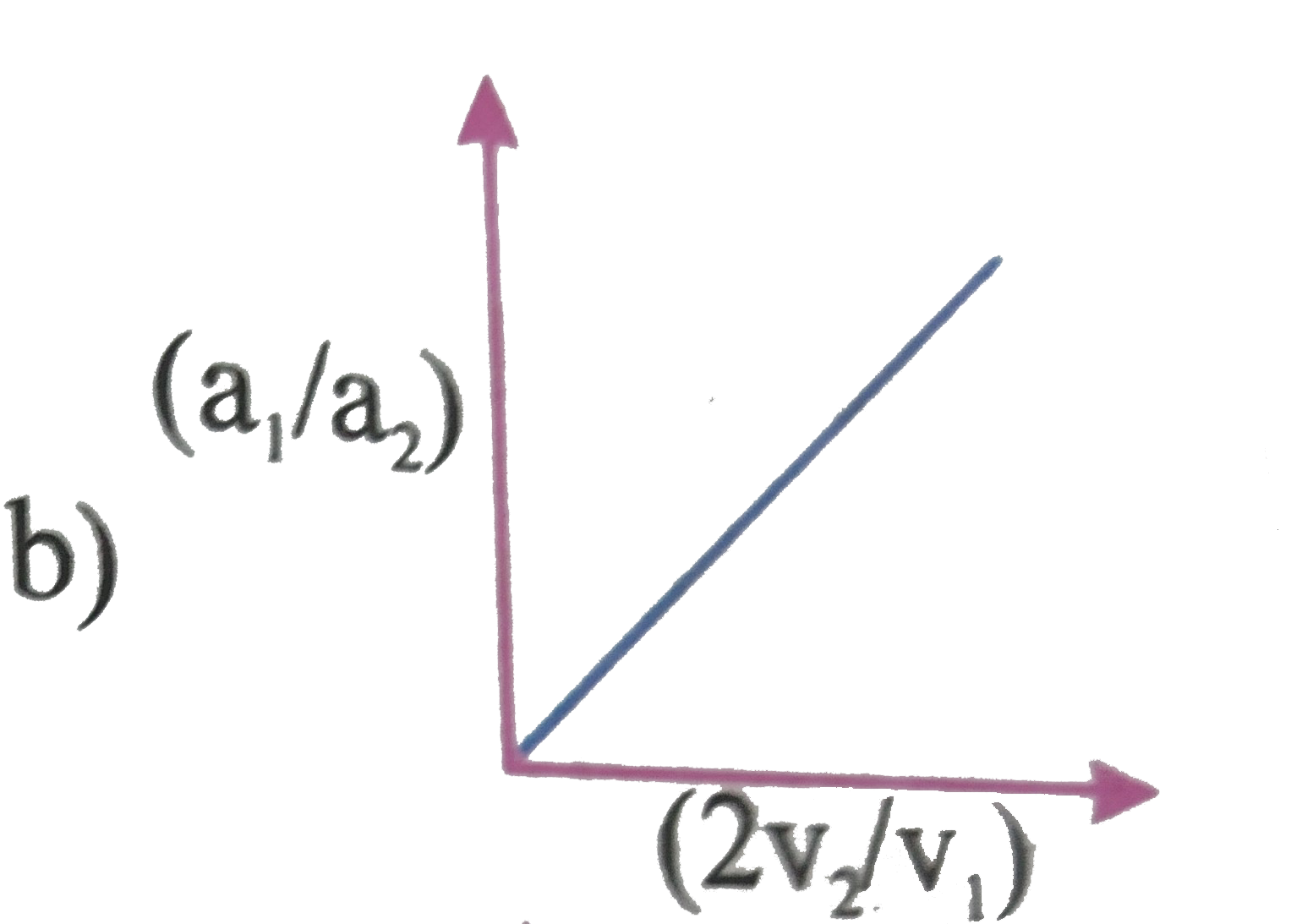 C
C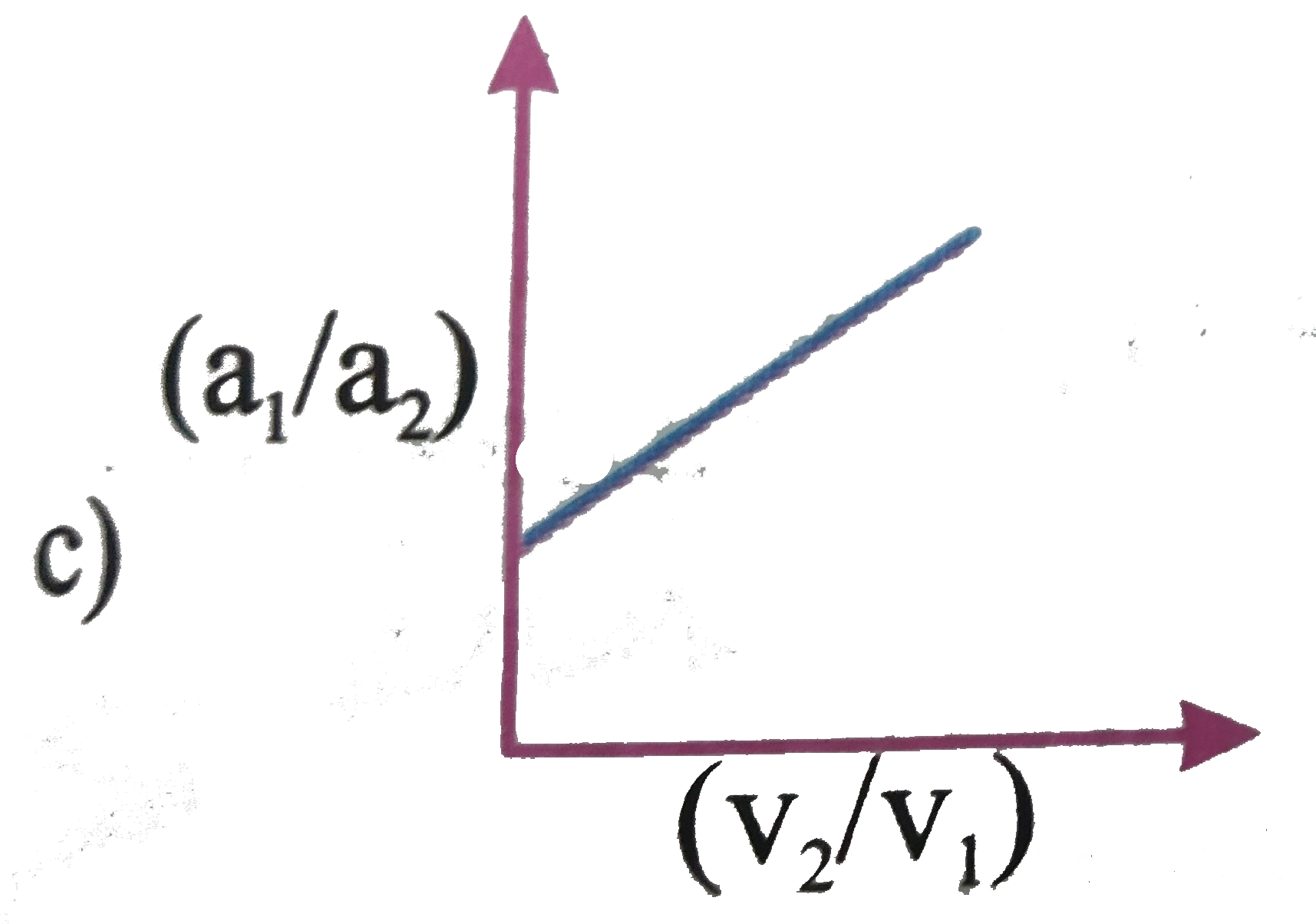 D
D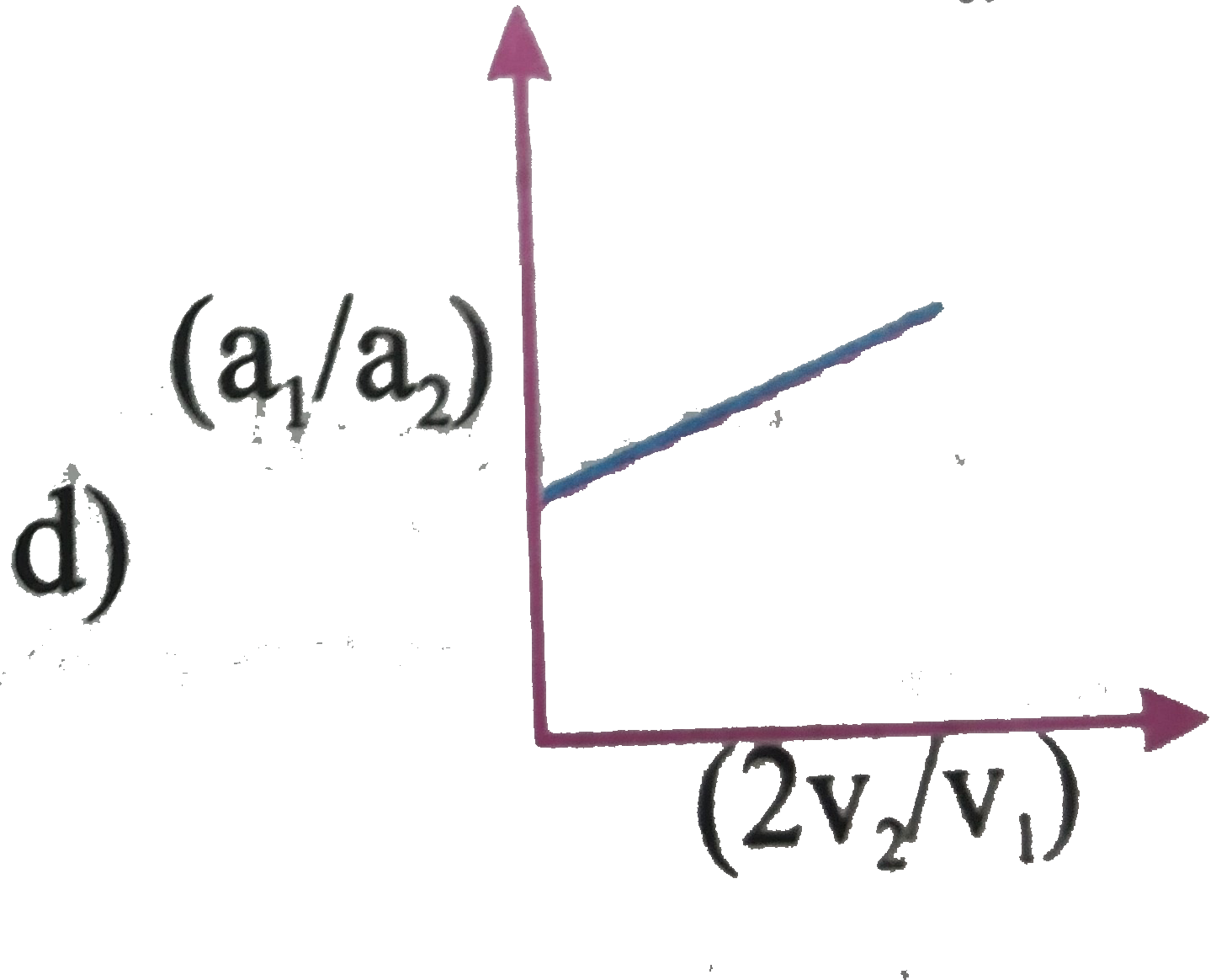
A vessel completely filled with water has holes 'A' and 'B' at depths 'h' and '2h' from the top, respectivel. Hole 'A' is a square of side 'L' and 'B' is circle of radish 'r' The water flowing out per second from both the holes is same Then, 'L'is equal to
View SolutionA tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
View SolutionThere are two holes P and Q at depths h and 4h from the top of a large vessel, completely filled with water. P is a square hole of side L and Q is a circular hole of radius r. If the same quantity of water flows out per second from both the holes, then the relation between L and r is
View SolutionA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionThere is a hole h metre from its top of a tank filled with water. Calculate the distance x where the stream of water will touch the ground from the bottom (in m).
 View Solution
View Solution

