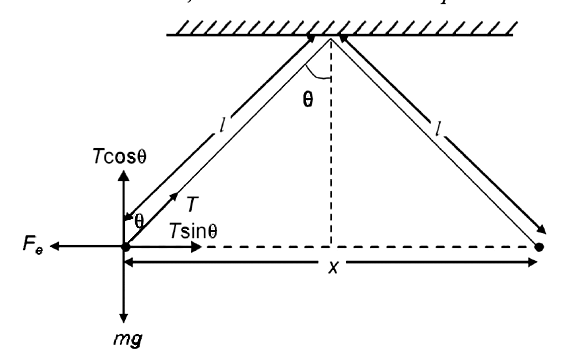
Two identical charged spheres suspended from a common point by two mass-less strings of length $l$ are initially at a distance d ( $d < < l$ ) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity $v$ . Then as a function of distance $x$ between them .

A

(a) $v \propto x^{- 1}$

B

(b) $v \propto x^{\frac{1}{2}}$

C

(c) $v \propto x$

D

(d) $v \propto x^{- \frac{1}{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

At any instant
$T cos θ = m g$ …(i)
$T sin θ = F_{e}$ …(ii)
$\Rightarrow \frac{sin θ}{cos θ} = \frac{F_{e}}{m g} \Rightarrow F_{e} = m g tan θ$
$\Rightarrow \frac{k q^{2}}{x^{2}} = m g tan θ \Rightarrow q^{2} \propto x^{2} tan θ$
$sin θ = \frac{x}{2 l}$
For small $θ, sin θ \approx tan θ$ $∴ q^{2} \propto x^{3}$

$\Rightarrow q \frac{d q}{d t} \propto x^{2} \frac{d x}{d t}$
$∴$ $\frac{d q}{d t} = c o n s t .$
$∴ q \propto x^{2} . v \Rightarrow x^{3 / 2} a x^{2} . v [∵ q^{2} \propto x^{3}]$
$\Rightarrow v \propto x^{- 1 / 2}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two identical charged spheres suspended from a common point by two massless strings of length $l$ are initially a distance d ( d ltlt l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate As a result the charge approach each other with a velocity $v$ . Then as a function of distance $x$ between them .
A $v \propto x^{1 / 2}$
B $v \propto x$
C $v \propto x^{1 / 2}$
D $c \propto x^{- 1}$
Question 2 - Select One
Two identical charged spheres suspended from a common point by two mass-less strings of length $l$ are initially at a distance d ( $d < < l$ ) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity $v$ . Then as a function of distance $x$ between them .
A(a) $v \propto x^{- 1}$
B(b) $v \propto x^{\frac{1}{2}}$
C(c) $v \propto x$
D(d) $v \propto x^{- \frac{1}{2}}$
Question 3 - Select One
Two identical charged spheres suspended from a common point by two mass-less strings of length $l$ are initially at a distance d ( $d < < l$ ) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity $v$ . Then as a function of distance $x$ between them .
A $v \propto x^{\frac{1}{2}}$
B $v \propto x$
C $v \propto x^{\frac{1}{2}}$
D $v \propto x^{- 1}$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-ELECTROSTATICS-Comprehension Based Questions

Home
Ask Doubt
Profile