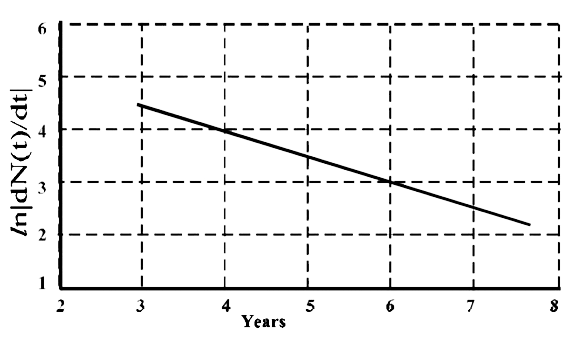
To determine the half life of a radioactive element , a student plot a graph of in $∣ ∣ ∣ \frac{d N (t)}{d t} ∣ ∣ ∣$ versus $t$ , Here $∣ ∣ ∣ \frac{d N (t)}{d t} ∣ ∣ ∣$ is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after $4.16$ year the value of p is

Video Solution

Text Solution

Verified by Experts

we know that $N = N_{0} e^{- λ t}$
:. (dN)/(dt) = N_(0)e^(-u) (-lambda) = -N_(0)e^(-11) $< b r > T a k \in g log o n ⊥ h s s < b r >$ (dN)/(dt) = log_(e) (-N_(0) lambda)) - lambda t $< b r > C o m p a r t \in g i t w i t h t h e g r a p h l \in e < b r > w e \geq t$ lambda= (1)/(2)gamma t^(-1) [(AC)/(BC) = (1)/(2))] $< b r > < i m g a \leq ocr_image l o a d \in g = lazy s r c = https://d10lpgp6xz60nq.cloudfront.net/physics_images/JMA_MP_C17_173_S01.png w h = 80% mx-auto s t y \leq = background:white; > < b r >$ :. T_(1//2) = ((0.693)/(lambda) = 0.693 xx 2 = 1.386 year $< b r > N o w$ (N)/(N_(0)) = ((1)/(2))^(1)/(T_(1//2)) rArr (1)/(p)= ((1)/(2)) ^(4.13)/*(1.386)) = (1)/(8)

|

Updated on:21/07/2023

Class 12 PHYSICS DUAL NATURE OF RADIATION AND MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The $t_{0.5}$ of a radioactive element is related to its average life by the expression
A $T_{12} = 1.44 T_{a v}$
B $T_{12} = 1.36 T_{a v}$
C $T_{12} = 0.693 T_{a v}$
D $T_{12} = \frac{T_{a v}}{0.693}$
Question 2 - Select One
The half life of a radioactive element is T and its initial activity at $t = 0$ is $A_{0}$ and at $t = t$ it is A, then
A $A = A_{0} 2^{t / T}$
B $A = A_{0} {(2 t)}^{T}$
C $A_{0} = A 2^{t / T}$
D $A_{0} = A 2^{- t / T}$
Question 3 - Select One
If $T$ is the half-life of a radioactive material, then the fraction that would remain after a time $\frac{T}{2}$ is
A $\frac{1}{2}$
B $\frac{3}{4}$
C $\frac{1}{\sqrt{2}}$
D $\frac{\sqrt{2} - 1}{\sqrt{2}}$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-MODERN PHYSICS-MCQ (One Correct Answer

Home
Ask Doubt
Profile