If −(dNdt)o is the initial activity and −(dNdt) is the activity at time t in a radioactive disintegration then :
−(dNdt)o=−(dNdt)e−kt
−(dNdt)o=(dNdt)oe−kt
−(dNdt)o=−(dNdt)oe−kt
−(dNdt)o=(dNdt)oe−kt
The correct Answer is:C
To solve the problem, we need to relate the initial activity of a radioactive substance to its activity at a later time using the principles of radioactive decay. Let's break down the solution step by step.
1. Understanding Activity:
The activity of a radioactive substance is defined as the rate of decay of the substance. Mathematically, it can be expressed as:
−dNdt=λN
where λ is the decay constant and N is the number of radioactive nuclei at time t.
2. Initial Activity:
At time t=0, the initial number of nuclei is N0. Therefore, the initial activity can be written as:
−dNdt∣∣∣t=0=λN0
3. Number of Nuclei at Time t:
The number of radioactive nuclei at any time t can be expressed using the exponential decay formula:
N=N0e−λt
4. Activity at Time t:
Substituting N into the activity equation gives us the activity at time t:
−dNdt=λN=λ(N0e−λt)=λN0e−λt
5. Relating Initial Activity to Activity at Time t:
We can express the activity at time t in terms of the initial activity:
−dNdt=−dNdt∣∣∣t=0e−λt
This shows that the activity at time t is equal to the initial activity multiplied by the exponential decay factor.
6. Final Relationship:
Therefore, we can conclude that:
−dNdt=−dNdt∣∣∣t=0e−λt
Conclusion:
The activity at time t is related to the initial activity by an exponential decay factor.
The activity of a radioactive substance is defined as the rate of decay of the substance. Mathematically, it can be expressed as:
−dNdt=λN
where λ is the decay constant and N is the number of radioactive nuclei at time t.
At time t=0, the initial number of nuclei is N0. Therefore, the initial activity can be written as:
−dNdt∣∣∣t=0=λN0
The number of radioactive nuclei at any time t can be expressed using the exponential decay formula:
N=N0e−λt
Substituting N into the activity equation gives us the activity at time t:
−dNdt=λN=λ(N0e−λt)=λN0e−λt
We can express the activity at time t in terms of the initial activity:
−dNdt=−dNdt∣∣∣t=0e−λt
This shows that the activity at time t is equal to the initial activity multiplied by the exponential decay factor.
Therefore, we can conclude that:
−dNdt=−dNdt∣∣∣t=0e−λt
The activity at time t is related to the initial activity by an exponential decay factor.
Similar Questions
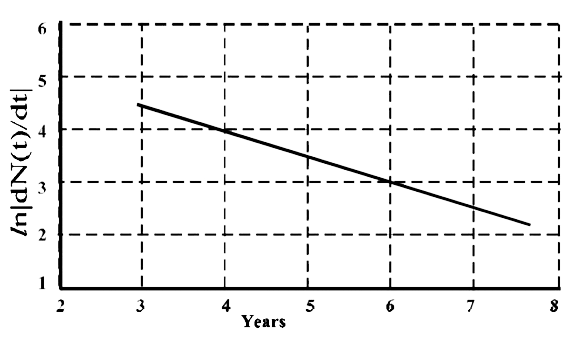
To determine the half life of a radioactive element , a student plot a graph of in ∣∣∣dN(t)dt∣∣∣ versus t , Here ∣∣∣dN(t)dt∣∣∣ is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after 4.16year the value of p is
 View Solution
View SolutionFor a radioactive material, its activity A and rate of change of its activity of R are defined as A=−dNdt and R=−dAdt, where N(t) is the number of nuclei at time t. Two radioactive source P (mean life τ) and Q (mean life 2τ) have the same activity at t=0. Their rates of activities at t=2τ are Rp and RQ, respectively. If RPRQ=ne, then the value of n is:
View Solution
Knowledge Check
- Question 1 - Select One
C14 is radioactive. The activity and the disintegration product are
Aβ−active, .7N14Bα− active, .7Be10CPositron active, .5B14Dγ−active ,C14 - Question 2 - Select One
dNdt=rN(K−NK)
in above equation (K−NK) represent:-ACarrying capacityBEnvironmental resistanceCRate of change in population densityDIntrinsic rate of growth - Question 3 - Select One
If the mass of a radioactive sample is doubled, the activity of the sample and the disintegration constant of the sample are respectively
AIncreases, remains the sameBDecreases, increasesCDecreases, remains sameDIncreases, decreases
Write two units for activity of radioactive element and relate them with number of disintegration per second.
View SolutionFor a radioactive meterial , its activity A and rate of charge of its activity R are defined as A=−dNdtandR=dAdt where N(t) is the number of nuclei at time I .Two radioactive source P(meanlifeτ)andQ(meanlife2τ) have the same activity at t=2τRpandRQ respectively , if RpRQ=ne
View SolutionDraw a graph showing the variation of decay rate (−dNdt) with number (N) of radioactive nuclei.
View SolutionIn a radioactive decay processthe activity is defined as A = - (dN)/dtwhereN(t)istheνmberofradioactiveνc≤iattimet.Tworadioactivesources,S_1andS_2havesameactivityattimet=0.Atalatertime,theactivitiesofS_1andS_2areA_1andA_2,respectively.WhenS_1andS_2havejustcomp≤tedtheir3rdand7thhalf−lives,respectively,theratioA_1/A_2 is.
View SolutionRadioactive isotopes are produced in a nuclear physics experiment at a constant rate dN/dt=R .An inductor of inductance 100 mH, a resistor of resistance 100Ω and a battery are connected to form a series circuit.the circuit is switched on at the instant the production of radioactive isotope starts. It is found that i//N remains constant in time where i is the current in the circuit at time t and N is the number of active nuclei at time t. Find the half-life of the isotope.
View Solution

