A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance 1.21Å between them. The wavelength of the standing wave is
1.21 Å
1.42 Å
6.05 Å
3.63 Å
The correct Answer is:A
Key Idea The given standing wave has segments.
The given standing wave is shown in the figure.

As length of one loop or segment is λ2, so length of 2 segments is 2 (λ2)
∴2λ2=1.21Å
⇒λ=1.21Å
Topper's Solved these Questions
Similar Questions
In a standing wave 4 nodes and 3 antinodes are formed between two fixed end of string of length 6 m. Then the wave length of the standing wave is
View SolutionA standing wave having 3 nodes and 2 antinodes are formed between two rigid supports of length 1m. Then the wavelength of the standing wave is
View Solution
Knowledge Check
- Question 1 - Select One
A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance 1.21Å between them. The wavelength of the standing wave is
A6.05ÅB2.42ÅC1.21ÅD3.63Å - Question 2 - Select One
A standing wave having 3 nodes and 2 antinodes is formed between two atoms having a distance 1.21Å between them. The wavelength of the standing wave is
A1.21ÅB1.42ÅC6.05ÅD3.63Å - Question 3 - Select One
A standing wave, having 5 nodes and 4 antinodes is formed between two atoms having a distance 1.21Å between them. The wavelength of the standing wave is
A1.21ÅB2.42ÅC6.05ÅD0.605Å
The string fixed at both ends has standing wave nodes for which distance between adjacent nodes is x1. The same string has another standing wave nodes for which distance between adjacent nodes is x2. If l is the length of the string then x2/x1=l(l+2x1). What is the difference in numbers of the loops in the two cases?
View SolutionFor a certain transverse standing wave on a long string , an antinode is formed at x=0 and next to it , a node is formed at x=0.10m , the displacement y(t)of the string particle at x=0 is shown in Fig.7.97.
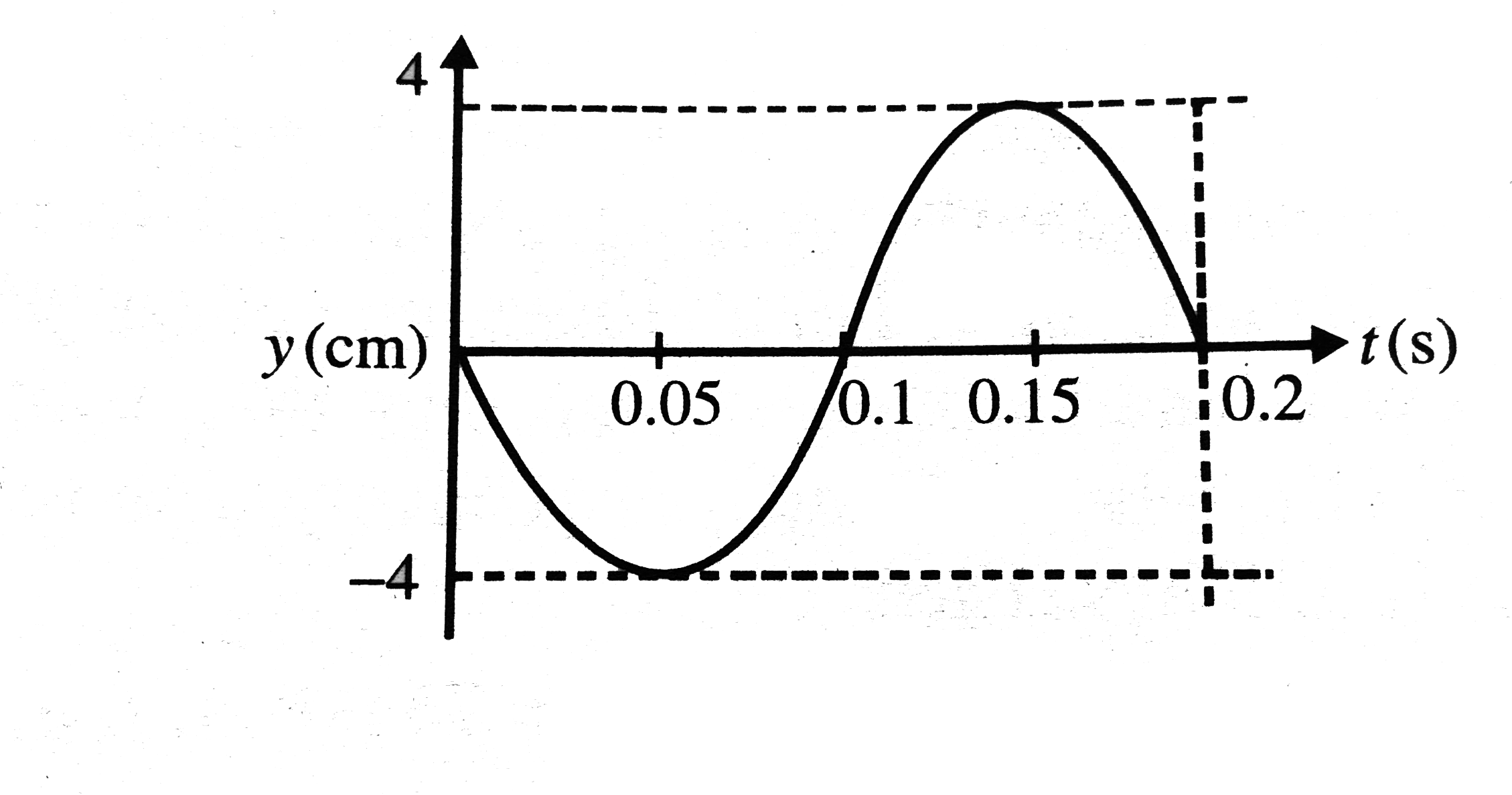 View Solution
View SolutionA string fixed at both ends has consecutive standing wave modes for which the distance between adjacent nodes are 18 cm and 16 cm respectively. The length of the string is -
View SolutionA standing wave in a string 35 cm long has a total of six nodes including those at the ends. Hence, wavelength of the standing wave is
View SolutionA string fixed at both ends has consecutive standing wave modes for which the distances between adjacent nodes are 18 cm and 16 cm respectively. The minimum possible length of the string (in cm) is:
View Solution

