In bringing an electron towards another electron, the electrostatic potential energy of the system
decreases
increases
remains same
becomes zero
The correct Answer is:B
To solve the problem of the electrostatic potential energy of a system when bringing one electron towards another electron, we can follow these steps:
1. Identify the Charges:
- We have two electrons, each with a charge of q=−1.6×10−19 C.
2. Understand the Interaction:
- Since both charges are negative, they will repel each other due to the electrostatic force.
3. Work Done Against Repulsion:
- When bringing one electron closer to another, work must be done against this repulsive force. This work done is stored as electrostatic potential energy in the system.
4. Formula for Electrostatic Potential Energy:
- The electrostatic potential energy U of two point charges is given by the formula:
U=14πϵ0q1q2r
- Here, q1 and q2 are the charges, r is the distance between them, and ϵ0 is the permittivity of free space.
5. Substituting Values:
- For two electrons (where q1=q2=−1.6×10−19 C), the formula becomes:
U=14πϵ0(−1.6×10−19)(−1.6×10−19)r=(1.6×10−19)24πϵ0r
6. Analyzing the Relationship:
- The potential energy U is inversely proportional to r. As r decreases (the electrons get closer), U increases.
7. Conclusion:
- Therefore, as we bring one electron closer to another, the electrostatic potential energy of the system increases.
Final Answer:
The electrostatic potential energy of the system increases when bringing an electron towards another electron.
---
- We have two electrons, each with a charge of q=−1.6×10−19 C.
- Since both charges are negative, they will repel each other due to the electrostatic force.
- When bringing one electron closer to another, work must be done against this repulsive force. This work done is stored as electrostatic potential energy in the system.
- The electrostatic potential energy U of two point charges is given by the formula:
U=14πϵ0q1q2r
- Here, q1 and q2 are the charges, r is the distance between them, and ϵ0 is the permittivity of free space.
- For two electrons (where q1=q2=−1.6×10−19 C), the formula becomes:
U=14πϵ0(−1.6×10−19)(−1.6×10−19)r=(1.6×10−19)24πϵ0r
- The potential energy U is inversely proportional to r. As r decreases (the electrons get closer), U increases.
- Therefore, as we bring one electron closer to another, the electrostatic potential energy of the system increases.
The electrostatic potential energy of the system increases when bringing an electron towards another electron.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion(A): In bringing an electron towards a proton electrostatic potential energy of the system increases
Reason (R ) :Potential due to proton is positive.ABoth (A) and (R) are true and 'R' is the correct explanation of A.BBoth A and R are true and R is not the correct explanation of ACA is true and R is falseDA is false and R is true. - Question 2 - Select One
When we bring two electrons close to each other, the electrostatic potential energy of the system:
AIncreasesBBecomes zeroCRemains unchangedDDecreases
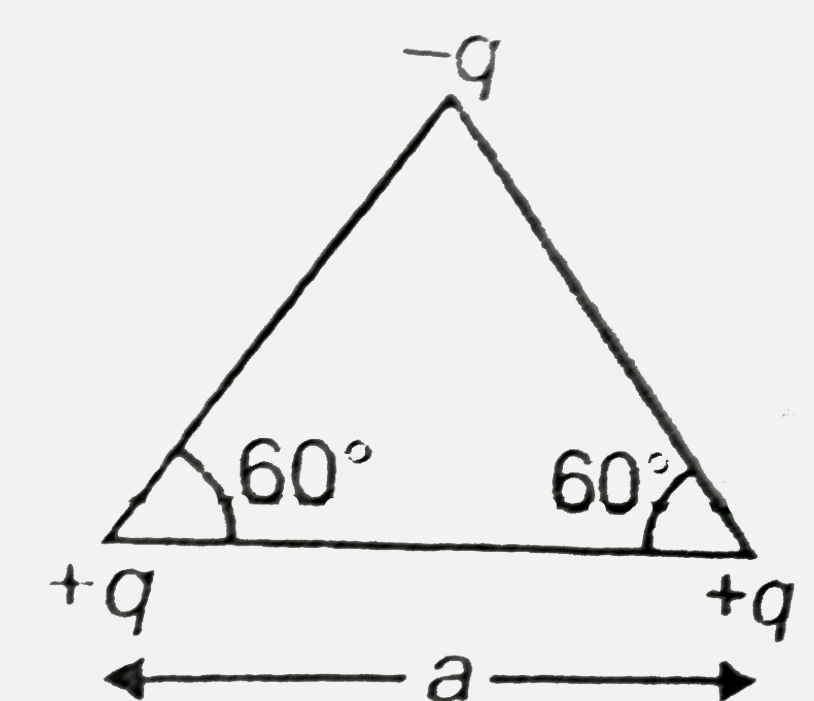
Three charges are placed at the vertex of an equilateral triangle as shown in figure. The value of Q, for which the electrostatic potential energy of the system is zero, is
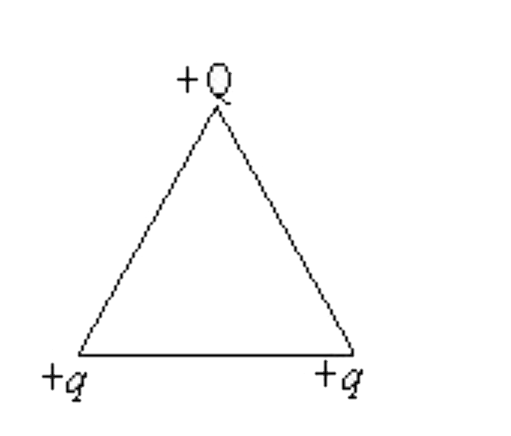 View Solution
View SolutionShow that the energy of hydrogen atom in the ground state is
E=−18πε0e2r
[Hint: Energy in the gound state = kinetic energy of electron in the first orbit + electrostatic potential energy of the electron.]View SolutionTwo electrons are held at some separation. A third charge q is to be placed at the midpoint between these two electrons. What should q be so that electric potential energy of the system becomes zero?
View SolutionThe classical method of a hydrogen atom in its normal, unexcited configuration has an electron that revolves around a proton at a distance of 5.3×1011m.
(a) What is the electric potential due to the proton at the position of electron ?
(b) Determine the electrostatic potential energy between the two particles.View SolutionWhat do you understand by electrostatic potential energy ? Find an expression for electrostatic potential energy of a system of two point charges.
View SolutionWhen a proton approaches another fixed proton, what happens to :
(a) the kinetic energy of the approaching proton
(b) the electric potential energy potential energy of the system and
(c ) the total energy of the system ?View Solution