Question

State and prove theorem of parallel axes.

Explanation

Theorem of parallel axes : The moment of inertia of a body about any axis is equal to the sum of its moment of inertia about a parallel axis passing through its centre of mass and the product of tis mass and the square of the perpendicular distance between the two parallel axes.

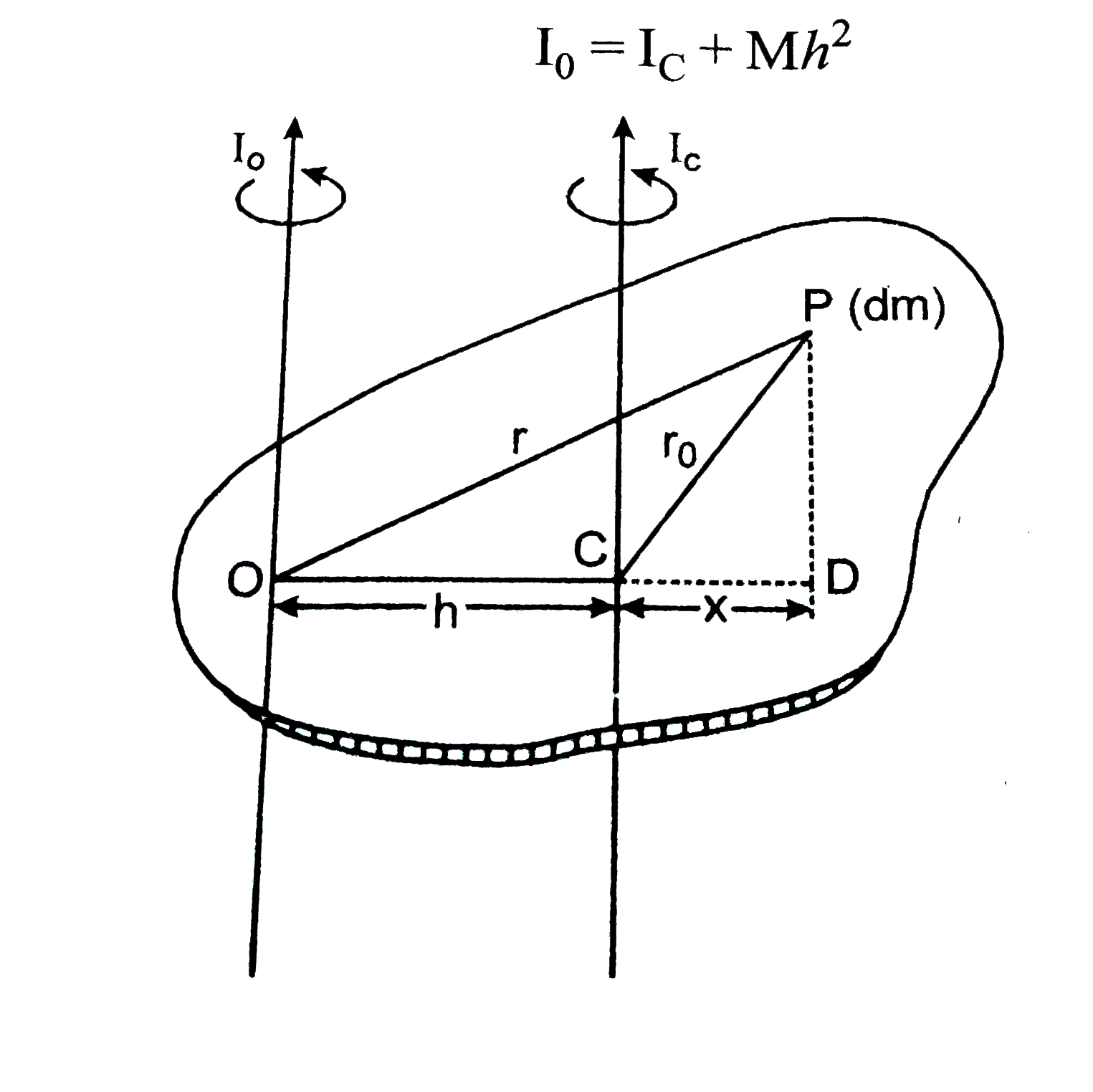
Consider a rigid body of mass M rotating about an axis passing through a point O and perpendicular to the plane of the figure (a).
Let, `I_(0)` be the moment of inertia of the body about an axis passing through point O. Take another parallel axis of rotation passing through the centre of mass of the body.
Let , `I_(C)` be the moment of inertia of the body about point C.
Let, the distance between the two parallel axes be `Oc = h`. Take a small element of body of mass dm situated at a point P and OP =r and `CP = r_(0)`. Join OP and CP, then
From point P draw a perpendicular to OC produced.
Let `CD =x`
From the figure,
`OP^(2) = OD^(2) + PD^(2)`
`:. OP^(2) = (h+CD)^(2) + PD^(2)`
`= h^(2) + CD^(2) + 2hCD + PD^(2)`
`:. OP^(2) = CP^(2) +h^(2) + 2hCD`
`( :' CD^2 + PD^(2) = CP^(2))`
`:. r^(2) = r_(0)^(2) + h^(2) + 2hx`
Multplying the above equation with dm on both the sides and integrating, we get
`intr^(2)dm = intr_(0)^(2) dm + inth^(2) dm + int2h xdm`
`intr^(2) dm = intr_(0)^(2) dm +inth^(2) dm + 2h int xdm`
`int xdm = 0` as C is the centre of mass and algebraic sum of moments of all the particles about the centre of mass is always zero, for body in equilibrium.
`:. int r^(2)dm = int r_(0)^(2) dm +h^(2) int dm + 0 "....." (i)`
But `int dm =` M = Mass of the body,
But `int dm = M` = Mass of the body,
`intr^(2) dm = I_(0)` and `int r_(0)^(2) dm = I_(C)`
Substituing in equation (i), we get
`I_(0) = I_(C) + Mh^(2)`
This proves the theorem of parallel axes about moment of inertia.