Question

State and prove parallel axes theorem.

Explanation

Statement : The moment of inertia of a plane lamina about an axis is equal to the sum of the moment of inertia about a parallel axis passing through the centre of mass and the product of its mass and square of the distance between the two axes i.e., `I=I_(g)+Mr^(2I)`
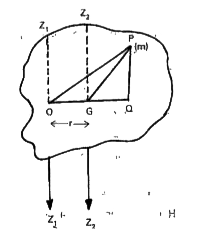
Let `I_(g)` is the moment of inertia of the plane lamina about the axis `Z_(2)` passing through the centre of mass.
`I_(0)` is the moment of inertia of the plane lamina about an axis `Z_(1)`.
Let M be the mass of the lamina and r be the distance between the two axes. Then `I_(0)=I_(g)+mr^(2)`.
Proof : Let a particle of mass m is situated at P. Moment of inertia about the axis pass in through 0(2) is `dl=mop^(2)orI=Sigmamop^(2)`
Join the lines r PO and PG and draw the line PQ and Join with the line extending from OG.
From the triangle POQ, `OP^(2)=OQ^(2)+PQ^(2)`
`OP^(2)=(OG+GQ)^(2)+PQ^(2)becauseOQ=OG+GQ`
`OP^(2)=OG^(2)+2OG.GQ+(GQ^(2)+PQ^(2))`
`OP^(2)=OG^(2)+2OG.GQ+GP^(2)`
`OP^(2)=OG^(2)+GP^(2)+2OG.GQ`
Multiplying with `Sigmam` on both sides `{:(because" From the "Deltal e),("PGQ, "GP^(2) =),(PQ^(2)+GQ^(2)):}`
`SigmamOP^(2)=SigmamOG^(2)+SigmamGP^(2)+SigmamOG.GQ`
but `SigmamOG^(2)=Mr^(2)`
(`because OG` is constant and `Sigmam` = M total mass of the body)
`SigmamGP^(2)=I_(g)`
`SigmamOP^(2)=I_(0)`

`therefore I_(0)Mr^(2)+I_(a)+2rSigmamGQ`
`Sigmam.GQ=0` [`because` The moment of all the particles about the centre of mass is always zero]
`I_(0)=I_(g)+Mr^(2)`
Thus the theorem is proved.