A body of mass 50 kg, suspended by two strings of lengths 30 cm and 40 cm fastend to two points in the same horizontal line 50 cm apart, is in equilibrium. Find the tension (in Newtons) in each string.
To solve the problem of finding the tension in each string when a body of mass 50 kg is suspended and in equilibrium, we can follow these steps:
Step 1: Identify the forces acting on the system
The weight of the body (W) can be calculated using the formula:
W=m⋅g
where:
- m=50kg (mass of the body)
- g=9.8m/s2 (acceleration due to gravity)
Calculating the weight:
W=50⋅9.8=490N
Step 2: Set up the geometry of the problem
We have two strings of lengths 30 cm (0.3 m) and 40 cm (0.4 m) attached to points that are 50 cm (0.5 m) apart horizontally. We can denote the points where the strings are attached as A and B, and the point where the mass is suspended as C.
Step 3: Determine the angles of the strings
Using the right triangle formed by the strings and the horizontal distance:
- For string 1 (length 0.3 m):
- Vertical height (h1) from point A to C = √(0.32−(0.25)2)=√0.09−0.0625=√0.0275≈0.165m
- For string 2 (length 0.4 m):
- Vertical height (h2) from point B to C = √(0.42−(0.25)2)=√0.16−0.0625=√0.0975≈0.312m
Step 4: Resolve the tensions into components
Let T1 be the tension in the string of length 30 cm and T2 be the tension in the string of length 40 cm.
- The vertical components of the tensions must balance the weight:
T1⋅h10.3+T2⋅h20.4=490
- The horizontal components of the tensions must balance each other:
T1⋅0.250.3=T2⋅0.250.4
Step 5: Set up the equations
From the horizontal balance:
T1⋅0.250.3=T2⋅0.250.4
This simplifies to:
4T1=3T2
or
T2=43T1
From the vertical balance:
T1⋅0.1650.3+T2⋅0.3120.4=490
Step 6: Substitute and solve for T1 and T2
Substituting T2 in terms of T1:
T1⋅0.1650.3+(43T1)⋅0.3120.4=490
This leads to:
0.165T10.3+4⋅0.312T13⋅0.4=490
Calculating the coefficients:
0.1650.3≈0.55
4⋅0.3123⋅0.4≈1.04
Combining:
(0.55+1.04)T1=490
1.59T1=490
T1=4901.59≈308.79N
Now substituting back to find T2:
T2=43T1=43⋅308.79≈411.72N
Final Answer
The tensions in the two strings are approximately:
- T1≈308.79N
- T2≈411.72N
The weight of the body (W) can be calculated using the formula:
W=m⋅g
where:
- m=50kg (mass of the body)
- g=9.8m/s2 (acceleration due to gravity)
W=50⋅9.8=490N
We have two strings of lengths 30 cm (0.3 m) and 40 cm (0.4 m) attached to points that are 50 cm (0.5 m) apart horizontally. We can denote the points where the strings are attached as A and B, and the point where the mass is suspended as C.
Using the right triangle formed by the strings and the horizontal distance:
- For string 1 (length 0.3 m):
- Vertical height (h1) from point A to C = √(0.32−(0.25)2)=√0.09−0.0625=√0.0275≈0.165m
- For string 2 (length 0.4 m):
- Vertical height (h2) from point B to C = √(0.42−(0.25)2)=√0.16−0.0625=√0.0975≈0.312m
Let T1 be the tension in the string of length 30 cm and T2 be the tension in the string of length 40 cm.
T1⋅h10.3+T2⋅h20.4=490
T1⋅0.250.3=T2⋅0.250.4
From the horizontal balance:
T1⋅0.250.3=T2⋅0.250.4
This simplifies to:
4T1=3T2
or
T2=43T1
T1⋅0.1650.3+T2⋅0.3120.4=490
Substituting T2 in terms of T1:
T1⋅0.1650.3+(43T1)⋅0.3120.4=490
0.165T10.3+4⋅0.312T13⋅0.4=490
0.1650.3≈0.55
4⋅0.3123⋅0.4≈1.04
(0.55+1.04)T1=490
1.59T1=490
T1=4901.59≈308.79N
T2=43T1=43⋅308.79≈411.72N
The tensions in the two strings are approximately:
- T1≈308.79N
- T2≈411.72N
Topper's Solved these Questions
Similar Questions
A body of mass 50 kg, suspended by two strings of lengths 30 cm and 40 cm fastend to two points in the same horizontal line 50 cm apart, is in equilibrium. Find the tension (in Newtons) in each string.
View SolutionA body of mass mis suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
View Solution
Knowledge Check
A body of 6⋅5 kg is suspended by two strings of lengths 5 and 12 metres attached to two points in the same horizontal line whose distance apart is 13 metre. The tension of the strings in kg wt. are
A3,5B2⋅5,6C4,5D3.4Two identical small bodies each of mass m and charge q are suspended from two strings each of length l from a fixed point. This whole system is taken into an orbiting artificial satellite, then find the tensio in strings
AKq2l2+2mgBKq24l2+2mgCKq2l2DKq24l2A uniform rod of length 15 cm is suspended from a fixed point by two strings of length 9 cm and 12 cm attached at its ends. If the inclination of the rod to the vertical is θ then sinθ is equal to
A25B1225C2425DNone
A block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.

View SolutionA block of mass M si suspended by two strings angles θ1 and θ2 with the horizontal. Find the tensions in the strings.
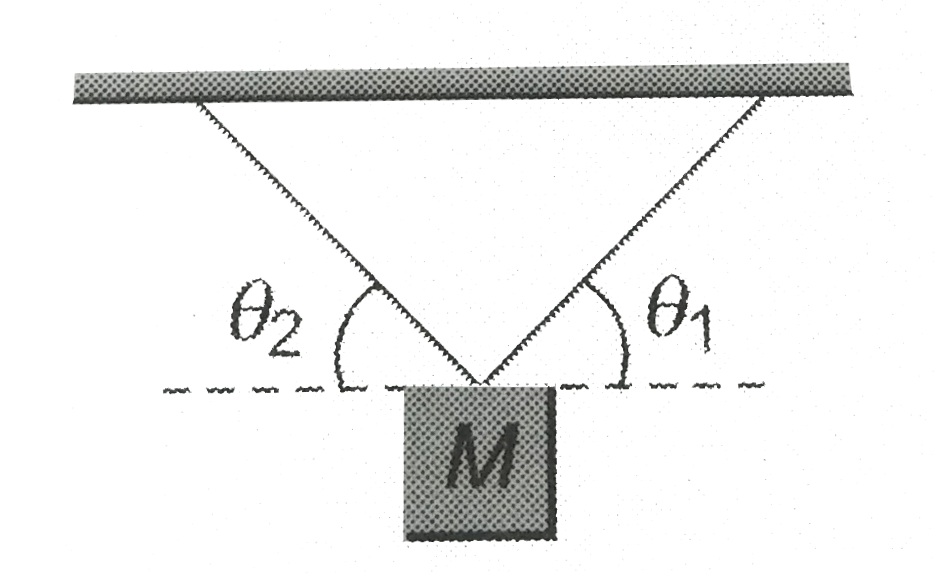
View SolutionA heavy particle of mass 2kg is tied to two strings, each of length 25 cm and they are tied to two points 30 cm apart on the same vertical line. The particle is whirled in a horizontal plane with a speed of 1.8ms−1. Calculate the ratio of the tensions of the two strings.
View SolutionA block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions T1andT2 in the two string.
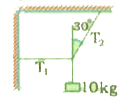
View SolutionTwo identical small bodies each of mass m and charge q are suspended from two strings each of length l from a fixed point. This whole system is taken into an orbiting artificial satellite, then find the tensio in strings
View Solution
