Average velocity of a particle executing SHM in one complete vibration is :
zero
Aω2
Aω
Aω22
The correct Answer is:A
To find the average velocity of a particle executing Simple Harmonic Motion (SHM) in one complete vibration, we can follow these steps:
1. Understanding SHM:
- A particle in SHM oscillates about an equilibrium position. It moves to a maximum displacement (amplitude) on one side, returns to the equilibrium position, moves to the maximum displacement on the opposite side, and then returns to the equilibrium position again.
2. Displacement in One Complete Cycle:
- In one complete vibration (or cycle), the particle starts from the equilibrium position, moves to the maximum positive displacement (amplitude), returns to the equilibrium position, moves to the maximum negative displacement, and finally returns to the equilibrium position.
- The total displacement after one complete cycle is zero because the particle ends up where it started.
3. Average Velocity Formula:
- Average velocity (V_avg) is defined as the total displacement divided by the total time taken for that displacement:
Vavg=Total DisplacementTotal Time
4. Calculating Average Velocity:
- Since the total displacement for one complete cycle is zero, we can substitute this into the average velocity formula:
Vavg=0T=0
- Here, T is the total time taken for one complete vibration.
5. Conclusion:
- Therefore, the average velocity of a particle executing SHM in one complete vibration is 0.
Final Answer:
The average velocity of a particle executing SHM in one complete vibration is 0.
- A particle in SHM oscillates about an equilibrium position. It moves to a maximum displacement (amplitude) on one side, returns to the equilibrium position, moves to the maximum displacement on the opposite side, and then returns to the equilibrium position again.
- In one complete vibration (or cycle), the particle starts from the equilibrium position, moves to the maximum positive displacement (amplitude), returns to the equilibrium position, moves to the maximum negative displacement, and finally returns to the equilibrium position.
- The total displacement after one complete cycle is zero because the particle ends up where it started.
- Average velocity (V_avg) is defined as the total displacement divided by the total time taken for that displacement:
Vavg=Total DisplacementTotal Time
- Since the total displacement for one complete cycle is zero, we can substitute this into the average velocity formula:
Vavg=0T=0
- Here, T is the total time taken for one complete vibration.
- Therefore, the average velocity of a particle executing SHM in one complete vibration is 0.
The average velocity of a particle executing SHM in one complete vibration is 0.
Topper's Solved these Questions
- NEETBOOK - NEET PREVIOUS YEAR (YEARWISE + CHAPTERWISE)CHAPTER - NEETEXERCISE - QUESTION
95 Videos
- NEETBOOK - NEET PREVIOUS YEAR (YEARWISE + CHAPTERWISE)CHAPTER - NEETEXERCISE - MCQ
95 Videos
- NEETBOOK - NEET PREVIOUS YEAR (YEARWISE + CHAPTERWISE)CHAPTER - NEETEXERCISE - MCQ
95 Videos
- MOTION IN TWO AND THREE DIMENSIONBOOK - NEET PREVIOUS YEAR (YEARWISE + CHAPTERWISE)CHAPTER - MOTION IN TWO AND THREE DIMENSIONEXERCISE - All Questions
47 Videos
- NEET 2020BOOK - NEET PREVIOUS YEAR (YEARWISE + CHAPTERWISE)CHAPTER - NEET 2020EXERCISE - All Questions
45 Videos
Similar Questions
Knowledge Check
The distance covered by a particle executing S.H.M. in one complete oscillation is
AABA/2C2AD4AThe average velocity of a particle executing SHM with an amplitude A and angular frequency ω during one oscillation is
AωABωA2C2ωA/πDzeroEnergy of particle executing SHM depends upon
Aamplitude onlyBAmplitude and frequencyCvelocity onlyDfrequency only
The maimum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View SolutionThe acceleration of a particle executing S.H.M. is
View Solution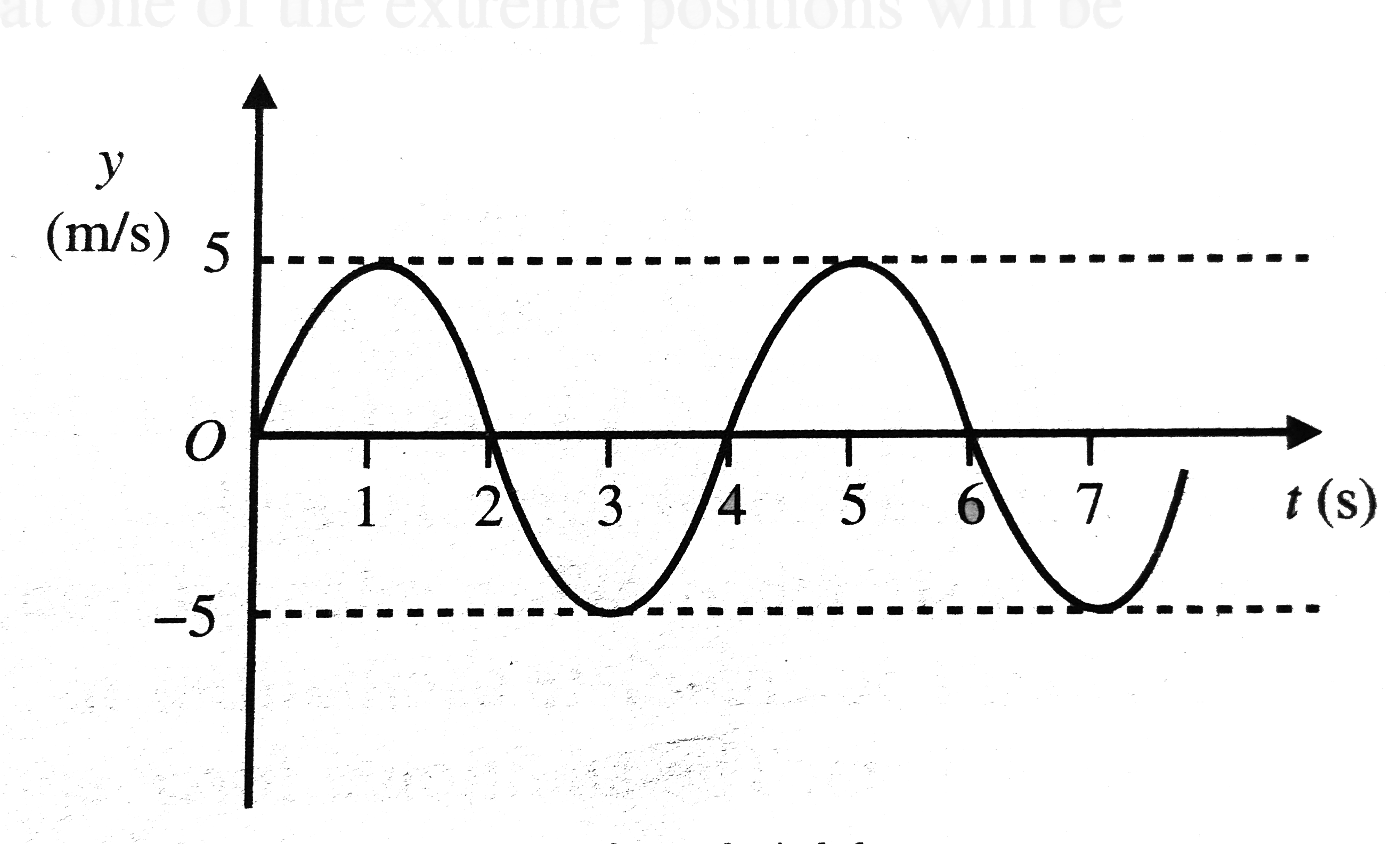
The variation of velocity of a particle executing SHM with time is shown is fig. The velocity of the particle when a phase change of π6 takes place from the instant it is at one of the extreme positions will be
View SolutionThe average acceleration of a particle performing SHM over one complete oscillation is
View SolutionThe average acceleration of a particle performing SHM over one complete oscillation is
View Solution
