Question

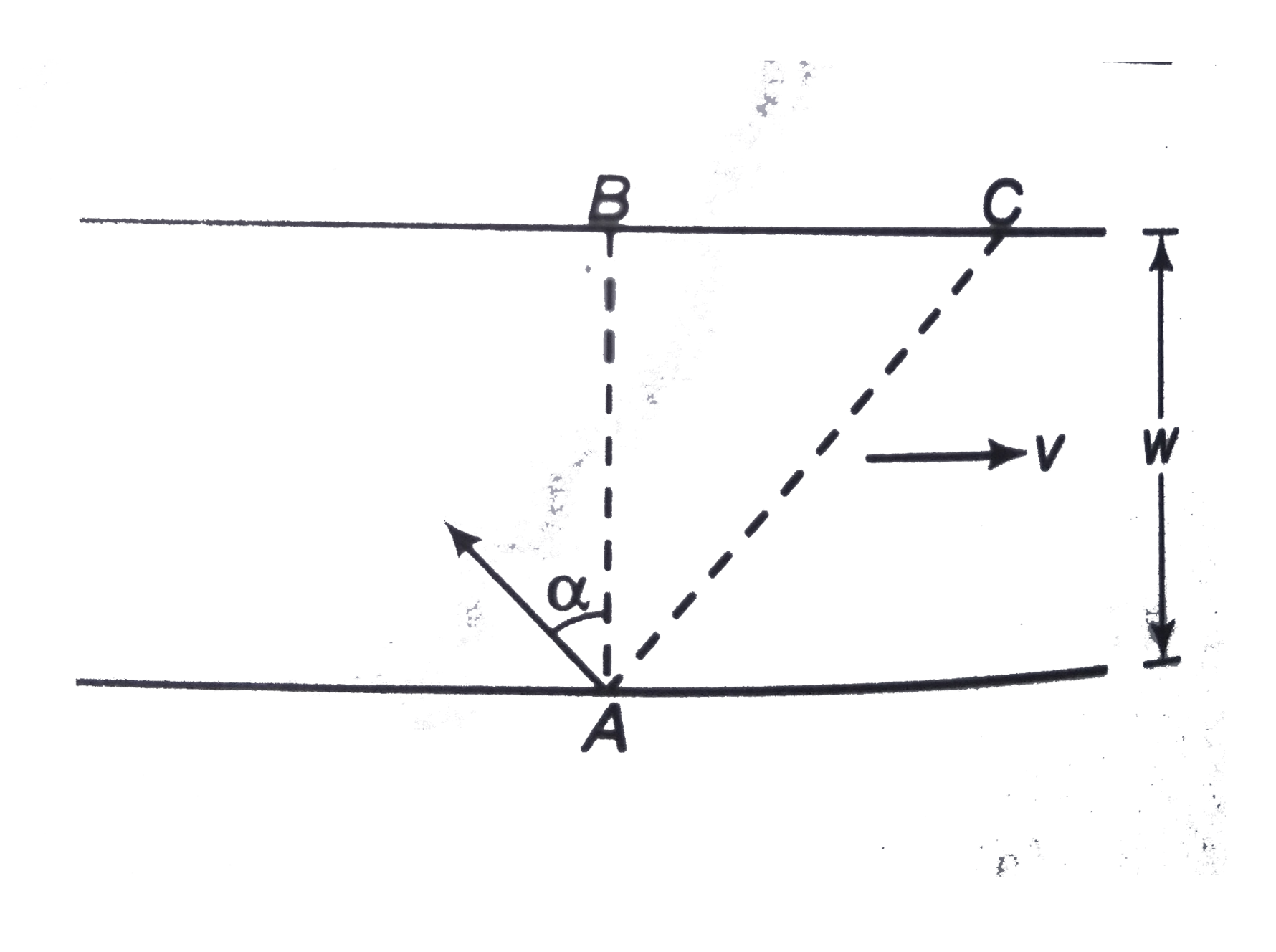
A man crosses a river in a boat. If he cross the river in minimum time he takes $10min$ with a drift $120m.$ If he crosses the river taking shortest path, he takes $12.5min,$ find
(a) width of the river
(b) velocity of the boat with respect to water
(c) speed of the current

Answer

A::B::C

Explanation

To solve the problem step by step, we will analyze the two scenarios given: crossing the river in minimum time and crossing the river in the shortest path.

Step 1: Understanding the Minimum Time Crossing
When the man crosses the river in minimum time, he goes straight across the river. The drift caused by the current is given as 120 m. The time taken for this crossing is 10 minutes.

1. Calculate the velocity of the river (vr):
\( vr = \frac{\text{displacement}}{\text{time}} = \frac{120 \text{ m}}{10 \text{ min}} = \frac{120 \text{ m}}{600 \text{ s}} = 0.2 \text{ m/s} \)

Step 2: Understanding the Shortest Path Crossing
In the second scenario, the man crosses the river taking the shortest path, which takes 12.5 minutes.

1. Let the width of the river be \(d\).
2. The velocity of the boat with respect to water is \(vb\).
3. The time taken to cross the river is 12.5 minutes or 750 seconds.

Using the formula for velocity:
\( vb = \frac{d}{12.5 \text{ min}} = \frac{d}{750 \text{ s}} \)

Step 3: Setting Up the Equations
1. For the minimum time crossing:
\( vb \sin \theta = \frac{d}{10 \text{ min}} = \frac{d}{600 \text{ s}} \quad \text{(1)} \)
2. For the shortest path crossing:
\( vb \cos \theta = vr = 0.2 \text{ m/s} \quad \text{(2)} \)

Step 4: Relating the Two Scenarios
From equation (1):
\( \sin \theta = \frac{d}{600 \cdot vb} \)
From equation (2):
\( \cos \theta = \frac{0.2}{vb} \)

Using the identity \(\sin^2 \theta + \cos^2 \theta = 1\):
\( \left(\frac{d}{600 \cdot vb}\right)^2 + \left(\frac{0.2}{vb}\right)^2 = 1 \)

Step 5: Simplifying the Equation
Substituting \(x = vb\):
\( \frac{d^2}{360000x^2} + \frac{0.04}{x^2} = 1 \)
Multiplying through by \(360000x^2\):
\( d^2 + 14400 = 360000x^2 \)
Rearranging gives:
\( d^2 = 360000x^2 - 14400 \quad \text{(3)} \)

Step 6: Finding \(d\) and \(vb\)
From equation (2):
\( vb = \frac{0.2}{\cos \theta} \)
Substituting into equation (3) and solving for \(d\) and \(vb\):
1. From \(vr = 0.2\):
\( vb = \frac{0.2}{\cos \theta} \)
2. Using \(d = 600 \cdot vb \sin \theta\) and substituting values.

Final Calculations
1. Calculate \(d\) using the derived equations.
2. Calculate \(vb\) using the relationship established.

Answers:
- (a) Width of the river \(d = 200 \text{ m}\)
- (b) Velocity of the boat \(vb = 20 \text{ m/min} = 0.33 \text{ m/s}\)
- (c) Speed of the current \(vr = 0.2 \text{ m/s}\)