A 1.8kg block is moved at constant speed over a surface for which coefficient of friction μ=14 it is pulled by a force F acting at 45∘ with horizontal as shown in Fig. The block is displaced by 2 m. Find the work done on the block by (a) the force F (b) friction (c) gravity.
 .
.
The correct Answer is:A, B, C
N=mg−Fsin45∘=18−F√2
Moving with constant speed means net force=0.
Fcos45∘−μN=14(18−F√2)
∴4F√2=18−F√2
∴F=18√25N
(a) WF=FScos45∘
=(18√25)(2)(1√2)=7.2J
(b) Wf=(μN)(S)cos180∘
=(14)(18−F√2)(2)(−2)
(c) Wmg=(mg)(s)cos90∘=0.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.29videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.32videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Miscellaneous Example6videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question11videos
WAVE MOTION
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
A wooden block of mass m resting on a rough horizontal table (coefficient of friction =μ ) is pulled by a force F as shown in figure.The acceleration of the block moving horizontally is:
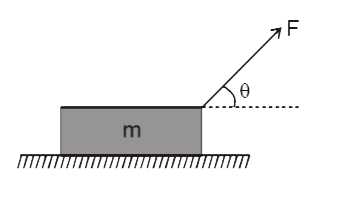 View Solution
View SolutionA block of mass m is pulled slowly by a minimum constant force on a rough (μ) horizontal surface through a distance x. Find work done by force F.
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m is lying on horizontal surface of coefficient of friction μ. A force is applied to the block at an angle θ with the horizontal. The block will move with a minimum force F if
Aμ=tanθBμ=cosθCμ=sinθDμ=cotθ - Question 2 - Select One
A horizontal force F pulls a 20 kg box at a constant speed along a rough horizotal floor. The coefficient of friction between the box and the floor is 0.25. The work done by force F on the block in displacing it by 2 m is
A49 JB98 JC147 JD196 J - Question 3 - Select One
A block of mass m lying on a rough horizontal surface of friction coefficient μ is pulled by a force F as shown , the limiting friction between the block and surface will be
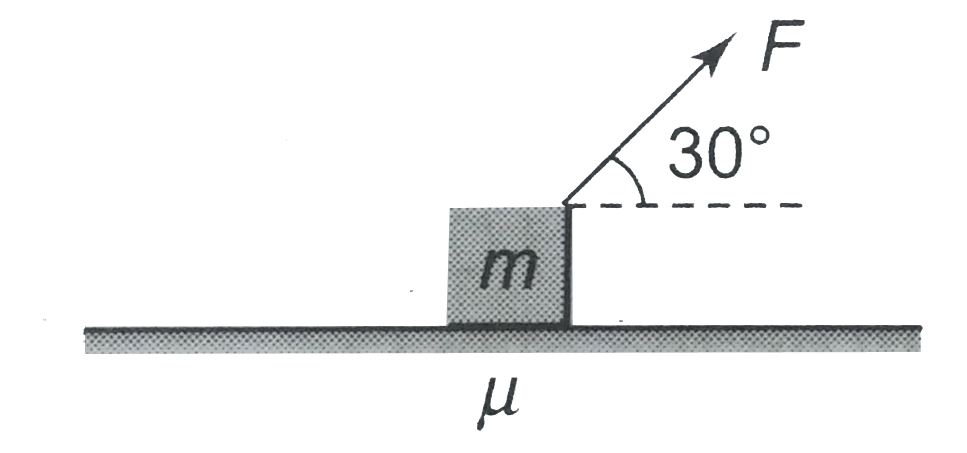 AμmgBμ(mg+F2)Cμ(mg−F2)Dμ(mg−√3F2)
AμmgBμ(mg+F2)Cμ(mg−F2)Dμ(mg−√3F2)
A force of 10N is acting on a block of 20kg on horizontal surface with coefficient of friction μ=0.2. Calculate the work done by the force.
View SolutionIn the fig. as shown mass of each block is same. The surface are rough with coefficient of friction μ. The block B moves with acceleration a. The frictional force on the block C is k×μma. Calculate the value of K
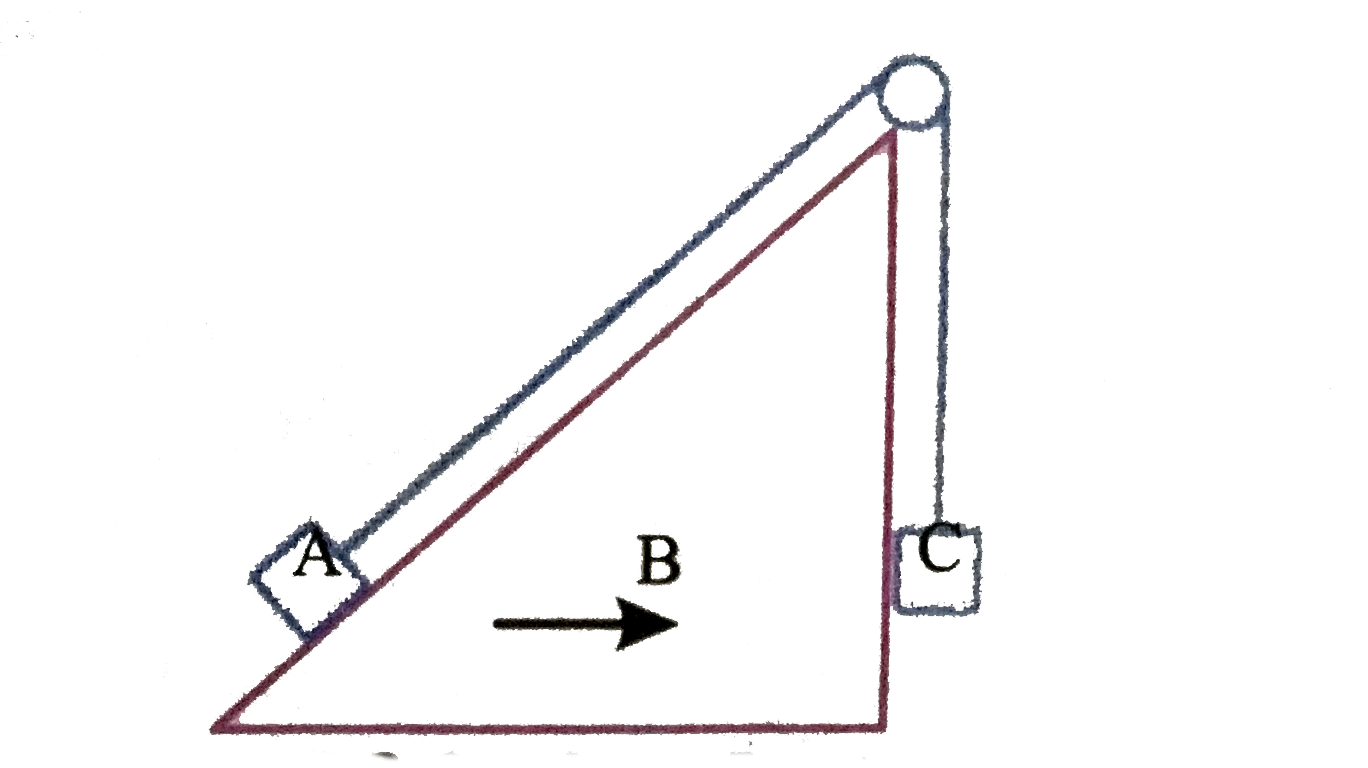 View Solution
View SolutionA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 View Solution
View SolutionA block of mass m is pulled slowly by a minimum constant force on a rough (μ) horizontal surface through a distance x. Find work done by force F.
View SolutionA horizontal force F acts on the block of mass m and the block remains stationary, thr value of friction force is.
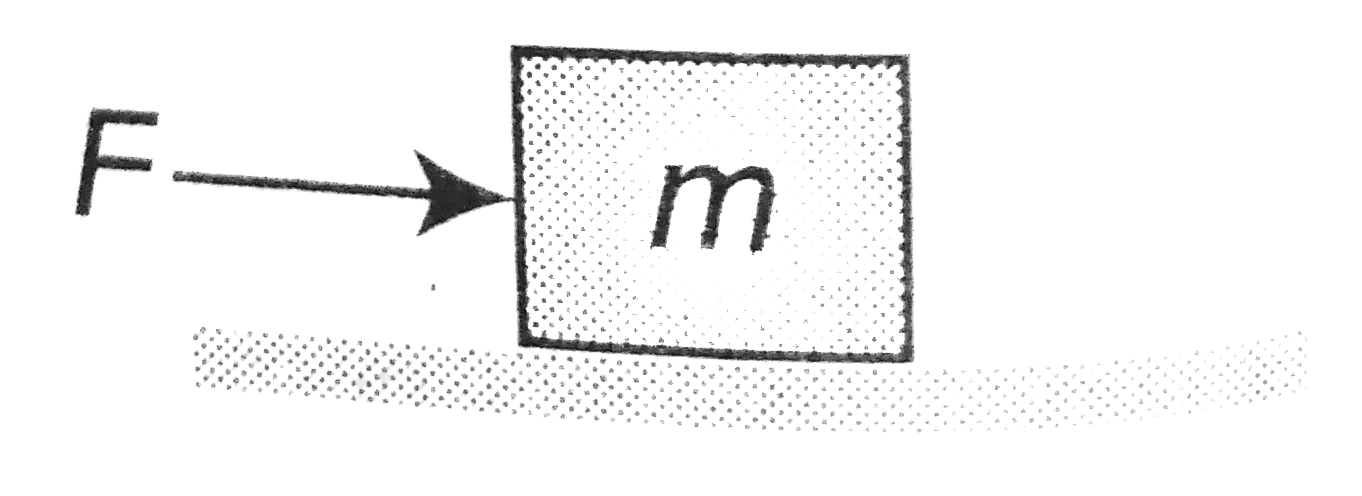 View Solution
View Solution

