The block shown in the figure is acted on by a sping with spring constant (k) and (a) weak frictional force of constant of constant magitude (f.) The block is pulled a distance x0 from equilibrium position and then then then fren released. It oscillates many times ultimately comes to rest.
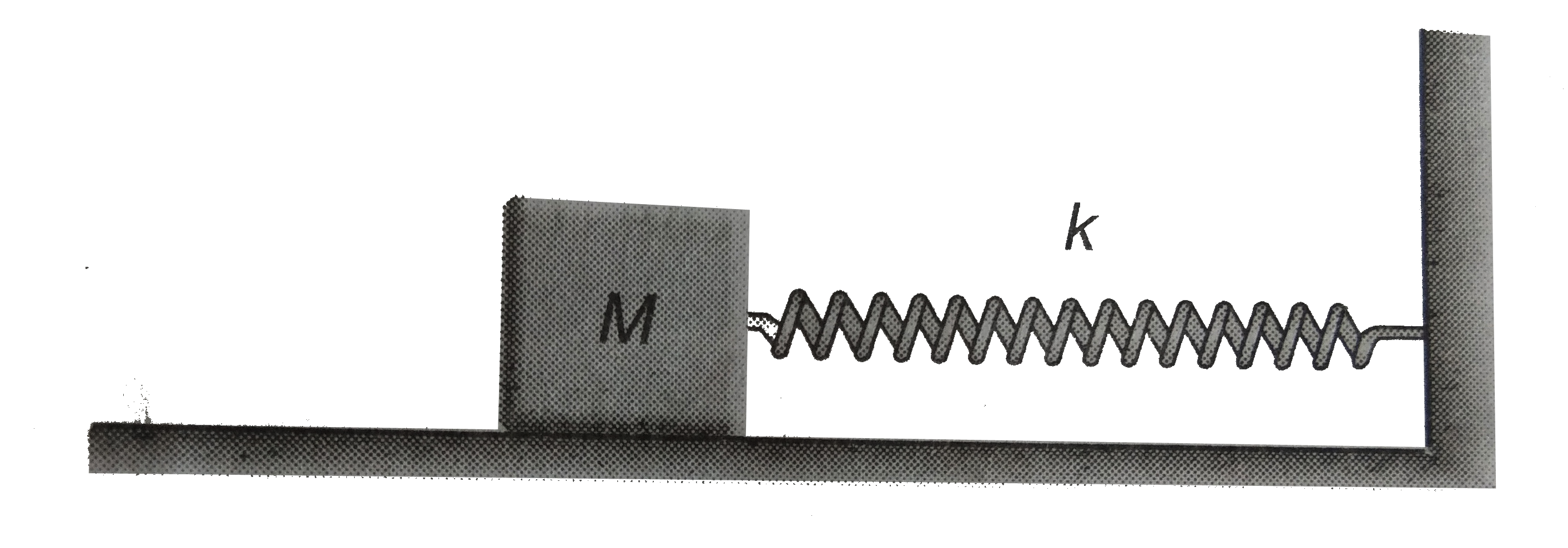
(a) Show that the decrease of amplitude is the same for each cycle of lscillation.
(b) Find the number of cycles the mass oscillates before coming to rest.
The correct Answer is:A, B, D
(a) From energy conservation principle,
Work done against friction=dicrease in elastic PE
or f(x0+a1)=12k(x20−a20)
or x0=a1=2fk...(i)
From Eq. (i), we see that decrease of amplitude
(x0−a1) is 2fk, which is constant and same for each cycle of oscillation.
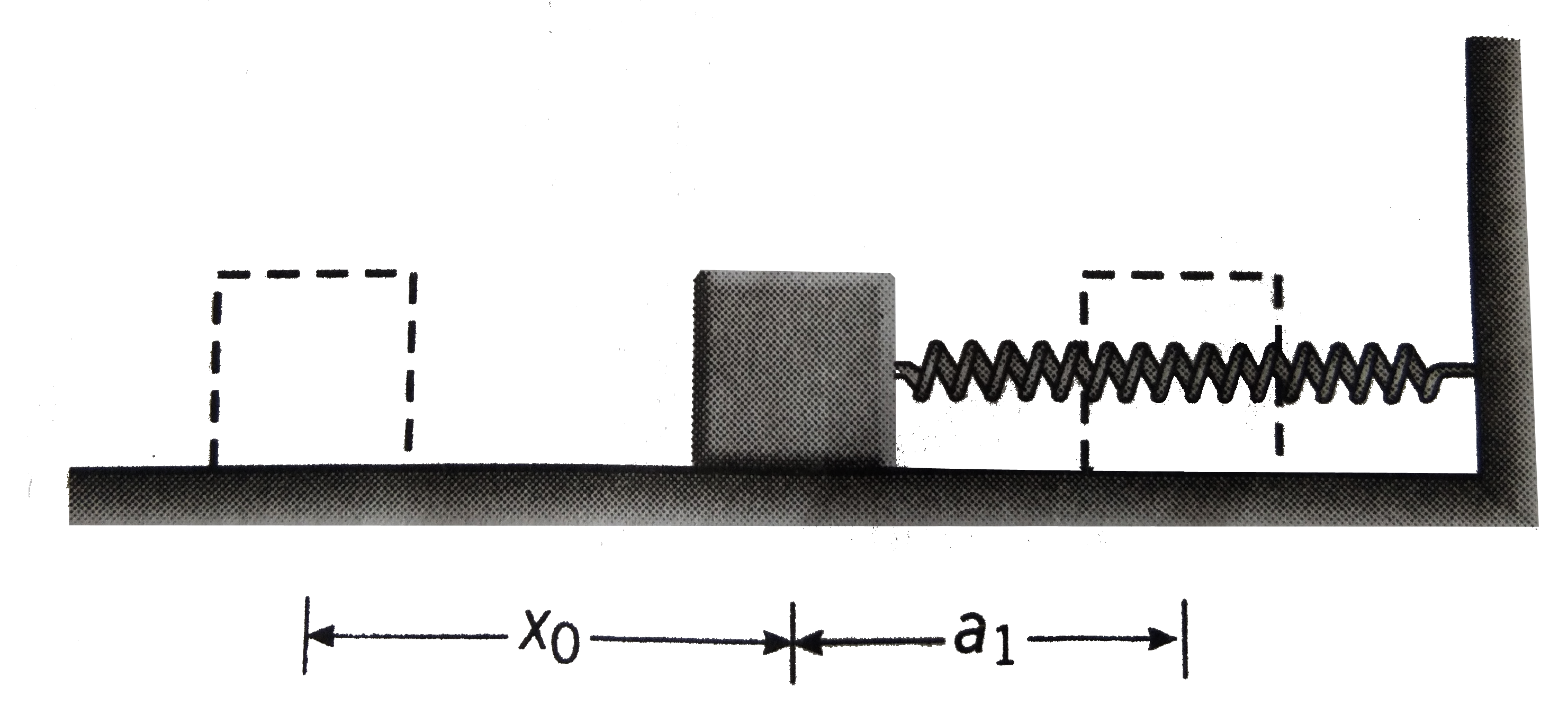
(b) The block will come to rest when ka=f
a=kf
In the similar manner, we can write
a1−a2=2fk....(i)
a2−a3=2fk......(ii)
an−1−an=2fk....(n)
Adding Eqs. (i),(ii),...etc., we get
x0−an=n(2fk)
or an=x0−n(2fk).....(B)
Equating Eq. (A) and (B), we get
kf=x0−n(2fk)
or n=x0−kf2f=kx02f−12
Number of cycles,
m=n2kx04f−14=14(kx0f−1).
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 More Than One Correct9videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question11videos
WAVE MOTION
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
A block whose mass m is 680g is fastened to a spring whose spring constant k is 65N/m. The block is pulled a distance x=11cm from its equilibrium position at x=0 on a frictionless surface and released from rest at t= 0.
What is the amplitude of the oscillation?View SolutionA block whose mass m is 680g is fastened to a spring whose spring constant k is 65N/m. The block is pulled a distance x=11cm from its equilibrium position at x=0 on a frictionless surface and released from rest at t= 0.
What is the phase constant ϕ for the motion?View Solution
Knowledge Check
- Question 1 - Select One
As shown in the figure, two light springs of force constant k1 and k2 oscillate a block of mass m. Its effective force constant will be
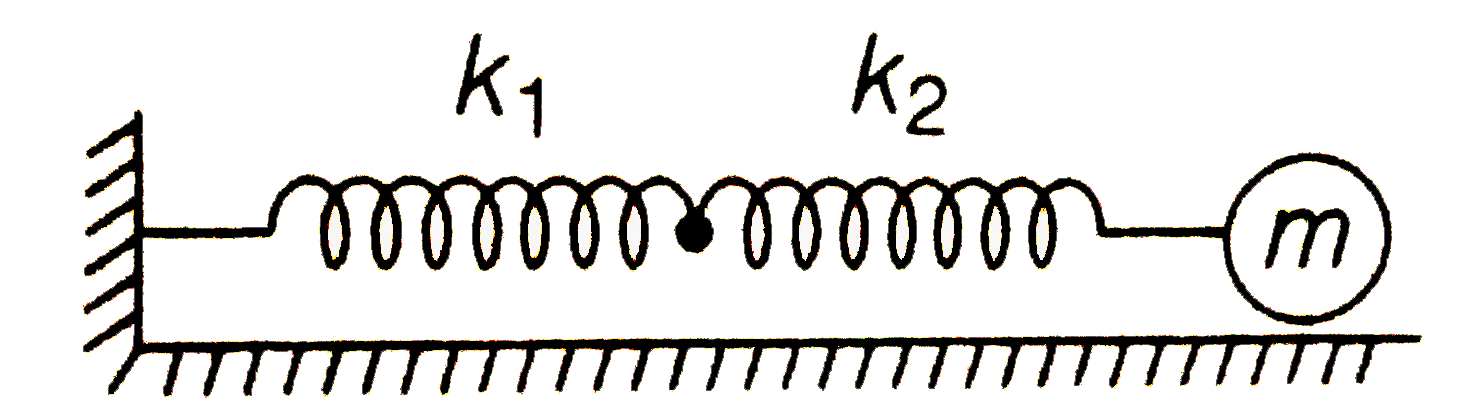 Ak1k2Bk1+k2C1k1+1k2Dk1k2k1+k2
Ak1k2Bk1+k2C1k1+1k2Dk1k2k1+k2 - Question 2 - Select One
Figure shows a system consisting of a massless pulley, a spring of force constant k and a block of mass m. If the block is slightly displaced vertically down from is equilibrium position and then released, the period of its vertical oscillation is
 A2π√mKBπ√m4KCπ√mKD4π√mK
A2π√mKBπ√m4KCπ√mKD4π√mK - Question 3 - Select One
A block of mass m length force a verical of spring constant k If the block is polled down by a distance of 2mg/k from its equilibrium position and released for the subsequent in the spring to maximum compressed in it mg/k
A2B3C4D1
A block of mass one kg is fastened to a spring with a spring constant 50Nm−1. The block is pulled to a distance x=10cm from its equilibrium position at x=0 on a frictionless surface from rest at t=0. Write the expression for its x(t) and v(t).
View SolutionA block whose mass m is 680g is fastened to a spring whose spring constant k is 65N/m. The block is pulled a distance x=11cm from its equilibrium position at x=0 on a frictionless surface and released from rest at t= 0.
What is the maximum speed vm of the oscillating block, and where is the block when it has this speed?View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
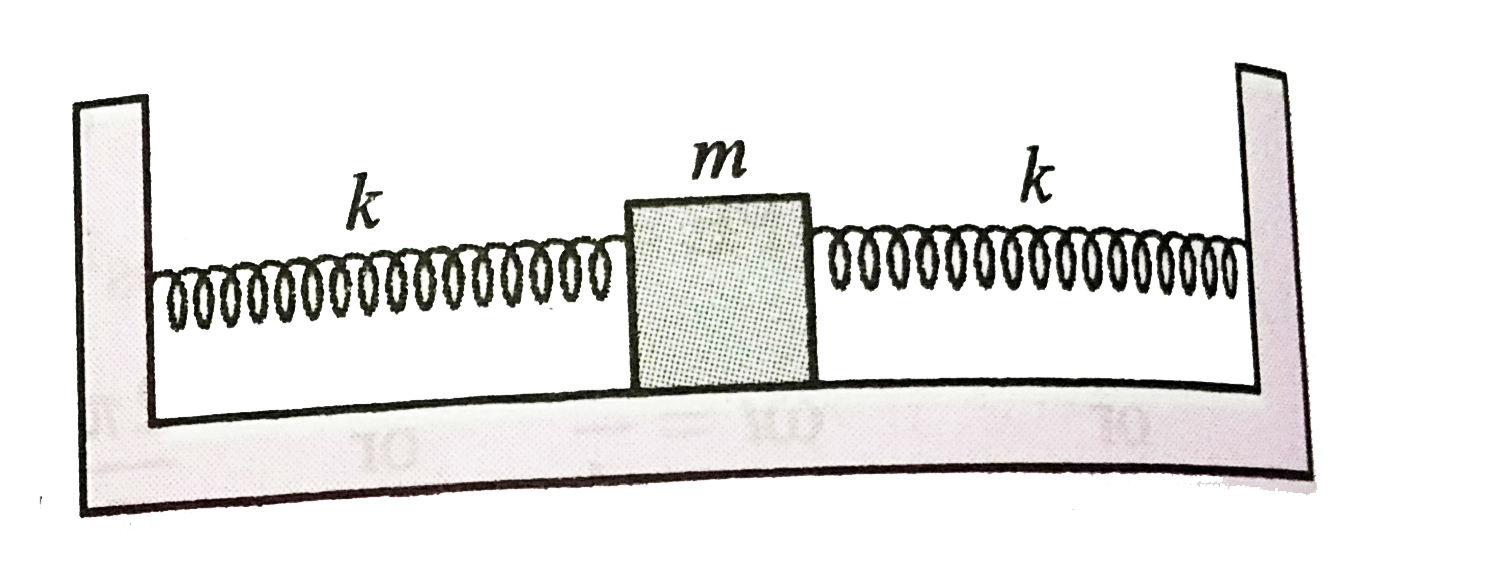 View Solution
View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.
 View Solution
View SolutionAn elastic string of constant k is attached to a block of mass m as shown. The block is given an extension of 2mgk from the equilibrium position and released. The time period of oscillations of the block is
 View Solution
View Solution

