ABCD is such a quadrilateral that A is the centre of the circle passing through B,CandD. Prove that ∠CBD+∠CDB=12∠BAD.
Step by step video solution for ABCD is such a quadrilateral that A is the centre of the circle passing through B, C and D. Prove that /_CBD+ /_CDB =1/2 /_BAD. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
ABCD is a cyclic quadrilateral whose side AB is a diameter of the circle through A, B, C, D. If ∠ADC=130∘, find ∠BAC.
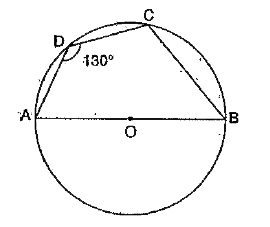 A40∘B50∘C60∘D30∘
A40∘B50∘C60∘D30∘
Circle with centre (-1,2) and passing through origin is
View SolutionA circle passes through A,B,C and D as shown in the figure. If ∠BAD=63∘, find x.
View SolutionIn Figure, ABCD is a cyclic quadrilateral whose side AB is a diameter of the circle through A, B, C, D. If (∠ADC)=1300, find ∠BAC
View SolutionIn a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that ∠COD=12(∠A+∠B).
View SolutionCircle will centre origin and passing through (-1,2) is
View SolutionIn a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that ∠COD=12(∠A+∠B)
View SolutionIn the following figure PQ is the tangent to the circle at A, DB is the diameter and O is the centre of the circle. If ∠ADB=30∘ and ∠CBD=60∘. Calculate :
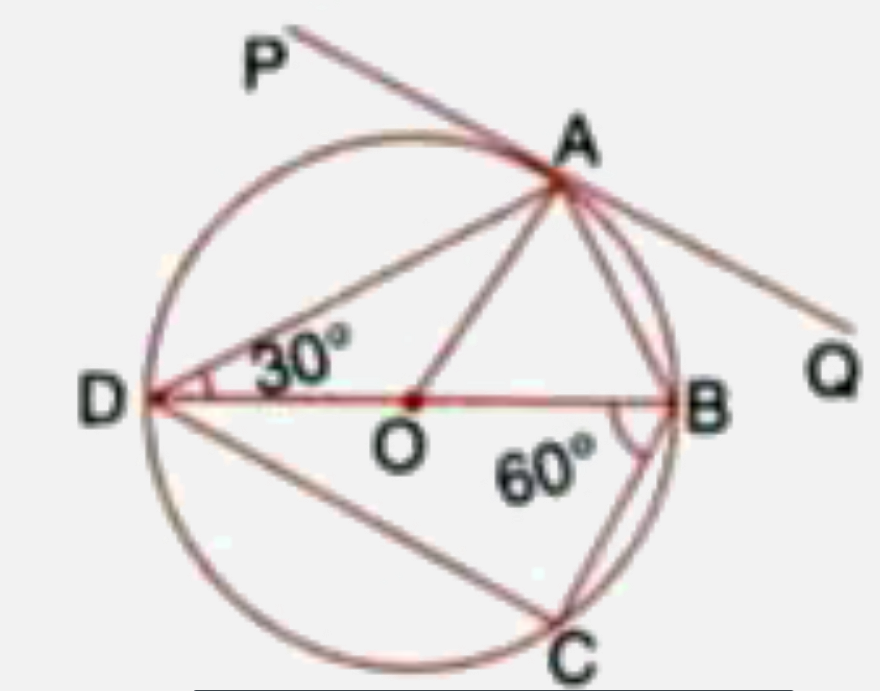
(i) ∠QAB
(ii) ∠PAD
(iii) ∠CDB
View Solution
