Consider a uniform rod of mass m and length 2l with two particles of mass $m$ each at its ends. Let AB be a line perpendicular to the length of rod and passig through its centre. Find the moment of inertial of the system about AB.

A

$\frac{5}{3} m l^{2}$

B

$\frac{7}{3} m l^{2}$

C

$\frac{8}{3} m l^{2}$

D

$\frac{11}{3} m l^{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

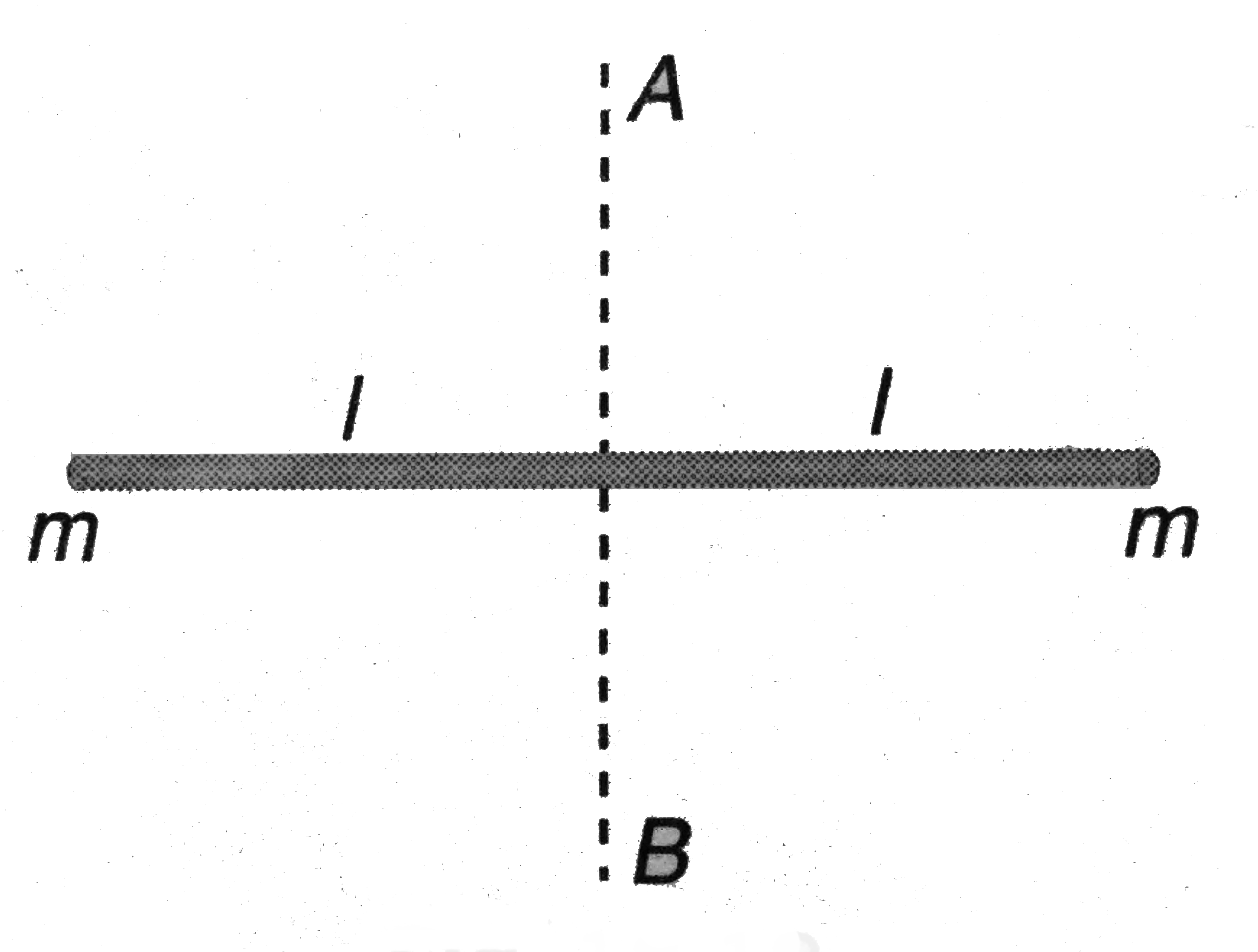
$I_{A B} = I_{r o d} + I_{both particles}$
$= \frac{m {(2 l)}^{2}}{12} + 2 (m l^{2})$
$= \frac{7}{3} m l^{2}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Moment of inertia of a thin rod of mass 'M' and length 'L' about the axis perpendicular to the rod and passing through its centre is ............
A $M L^{\frac{2}{4}}$
B $M L^{\frac{2}{6}}$
C $M L^{\frac{2}{12}}$
D $M L^{\frac{2}{2}}$
Question 2 - Select One
If $l_{1}$ is the moment of inertia of a thin rod about an axis perpendicular to its length and passing through its centre of mass and $l_{2}$ is the moment of inertia (about central axis) of the ring formed by bending the rod, then the ratio of $I_{1}$ to $I_{2}$ is
A $l : l = 1 : 1$
B $l : l = π : 3$
C $l : l = π : 4$
D $l : l = 3 : 5$
Question 3 - Select One
The M.I. of thin uniform rod of mass 'M' and length 'l' about an axis passing through its centre and perpendicular to its length is
A $M l^{2}$
B $M l^{2} / 3$
C $M l^{2} / 2$
D $M l^{2} / 12$

DC PANDEY-ROTATIONAL MECHANICS-Subjective Questions

Home
Ask Doubt
Profile