Show that if a body be projected vertically upward from the surface of the earth so as to reach a height nR above the surface
(i) the increase in its potential energy is (nn+1)mgR,
(ii) the velocity with it must be projected is √2ngRn+1, where r is the radius of the earth and m the mass of body.
The correct Answer is:A
(i) ΔU=mgh1+hR
putting h=nR, we get
ΔU=(nn+1)mgR
(ii) ΔU=(nn+1)mgR
12mν2
∴ν=√2ngRn+1
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Exercise 13.63videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Exercise 13.75videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Exercise 13.44videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE11videos
KINEMATICS
Similar Questions
A body is projected vertically upward from the surface of earth with a velocity sufficient to carry it to initially. Calculate the time taken by it to reach height h.
View SolutionA body of mass m is taken from earth surface to the height h equal to radius of earth, the increase in potential energy will be
View Solution
Knowledge Check
- Question 1 - Select One
A body is projected vertically upward from the surface of the earth, then the velocity-time graph is:-
A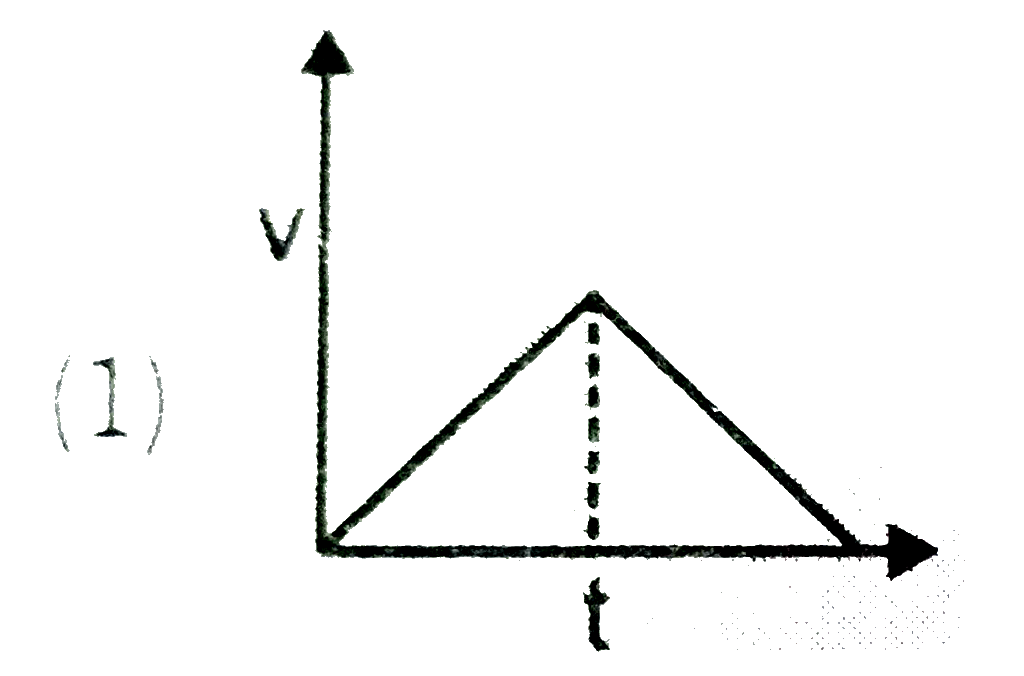 B
B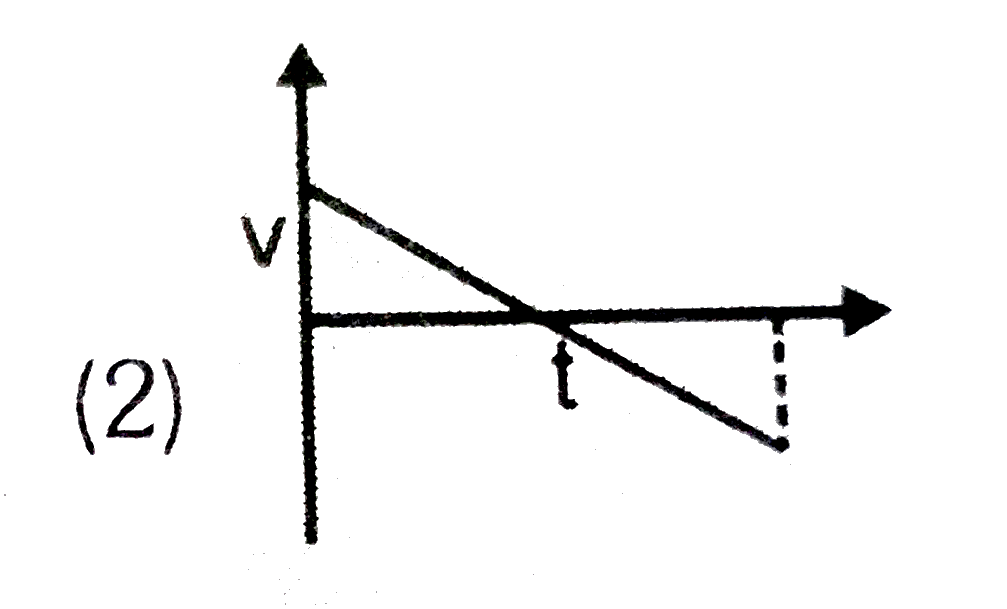 C
C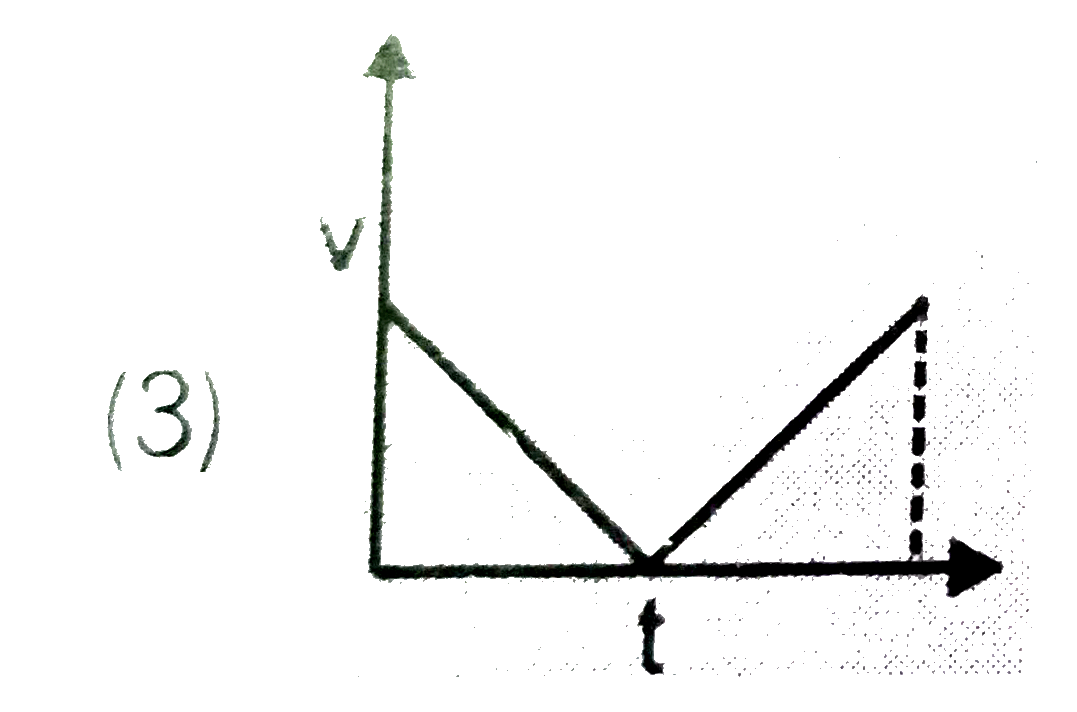 D
D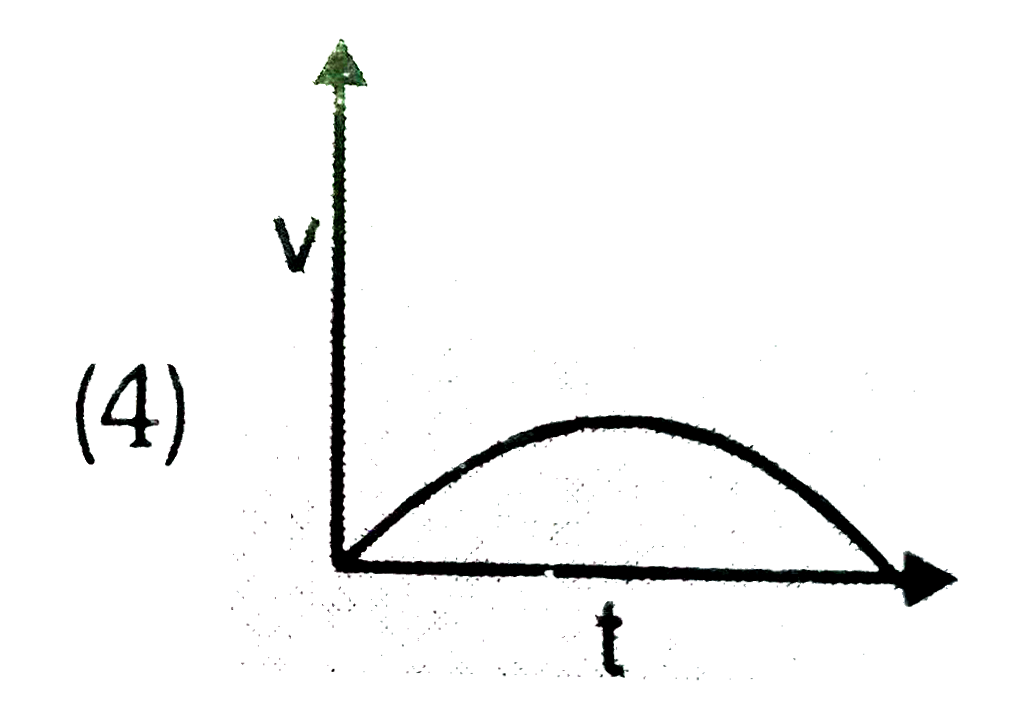
- Question 2 - Select One
An object is taken to height 2R above the surface of earth , the increase in potential energy is [ R is radius of earth ]
AmgR2BmgR3C2mgR3D2mgR - Question 3 - Select One
If a body is to be projected vertically upwards from earth's surface to reach a height of 10R where R is the radius of earth. The velocity required to be si is
A√2411grB√2211grC√2011grD√1811gr
The escape velocity of a body projected vertically upwards from the surface of the earth is v.If the body is projected in a direction making an angle θ with the vertical, the vetical, the escape velocity would be
View SolutionWhen a body id lifted from surface of earth height equal to radius of earth, then the change in its potential energy is
View SolutionA body projected from the surface of the earth attains a height equal to the radius of the earth. The velocity with which the body was projected is
View SolutionA body is projected vertically up from surface of the earth with a velocity half of escape velocity. The ratio of its maximum height of ascent and radius of earth is
View SolutionA body of mass m rises to a height h=R/5 from the surface of earth. If g is the acceleration due to gravity at the surface of earth, the increase in potential energy is (R = radius of earth)
View Solution

