A tunnel is dug in the earth across one of its diameter. Two masses m and 2m are dropped from the two ends of the tunel. The masses collide and stick each other. They perform SHM, the ampulitude of which is (R= radius of earth)
R
R/2
R/3
2R/3
The correct Answer is:C
To solve the problem, we will analyze the situation step by step.
Step 1: Understanding the System
We have a tunnel dug through the Earth along its diameter. Two masses, m and 2m, are dropped from either end of the tunnel. When they meet, they collide and stick together, forming a single mass of 3m.
Hint: Visualize the scenario with a diagram showing the tunnel and the two masses.
Step 2: Forces Acting on the Masses
As the masses fall towards the center of the Earth, they experience gravitational force. Inside the Earth, the gravitational force acting on a mass at a distance r from the center is given by:
\( F = -\frac{G M(r) m}{r^2} \)
where \( M(r) \) is the mass of the Earth within radius r. By the shell theorem, this force can be simplified to:
\( F = -\frac{G M_e m}{R^3} r \)
where \( M_e \) is the total mass of the Earth, and \( R \) is the radius of the Earth.
Hint: Recall the shell theorem and how gravity behaves inside a spherical shell.
Step 3: Equation of Motion
The acceleration a of the masses can be expressed as:
\( a = -\frac{G M_e}{R^3} r \)
This is the equation of motion for simple harmonic motion (SHM), where:
\( \omega^2 = \frac{G M_e}{R^3} \)
Hint: Identify the form of the SHM equation and relate it to the standard form \( a = -\omega^2 x \).
Step 4: Amplitude of SHM
When the two masses collide and stick together, they will oscillate about the center of the tunnel. The amplitude of their oscillation will be equal to the maximum distance they can reach from the center, which is equal to the radius of the Earth \( R \).
Hint: Think about the maximum displacement in SHM, which is the amplitude.
Step 5: Conclusion
Thus, the amplitude of the resulting SHM after the collision of the two masses is:
\( \text{Amplitude} = R \)
Final Answer: The amplitude of the SHM performed by the combined mass after the collision is \( R \) (the radius of the Earth).
We have a tunnel dug through the Earth along its diameter. Two masses, m and 2m, are dropped from either end of the tunnel. When they meet, they collide and stick together, forming a single mass of 3m.
As the masses fall towards the center of the Earth, they experience gravitational force. Inside the Earth, the gravitational force acting on a mass at a distance r from the center is given by:
The acceleration a of the masses can be expressed as:
When the two masses collide and stick together, they will oscillate about the center of the tunnel. The amplitude of their oscillation will be equal to the maximum distance they can reach from the center, which is equal to the radius of the Earth \( R \).
Thus, the amplitude of the resulting SHM after the collision of the two masses is:
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 2 More Than One Correct
10 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 2 Subjective
14 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Level 1 Subjective
19 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
Similar Questions
A tunnel is dug through the centre of the earth. Shwo that a body of mass m when dropped form rest from one end of the tunnel will execute simple harmonic motion.
View SolutionA tunnel is dug inside the earth across one of its diameters. Radius of earth is R and its mass is M. A particle is projected inside the tunnel with velocity √2GMR from one of its ends then maximum velocit attained by the particle in the subsequent motion is √nGMR (assuming tunnel to be frictionless). Find n
View Solution
Knowledge Check
A hole is drilled along the diameter of the earth and pen is dropped into it. The time taken by it is reach other end of the earth is
A162.2 minB84.6 minC21.2 minD42.3 minA straight tuning is due into the earth as shows in figure at a distance b from its centre. A ball of mass m is dropped from one of its ends. The time it takes to reach the other end is approximately
 A42minB84minC84(bR)minD42(bR)min
A42minB84minC84(bR)minD42(bR)minA tunnel is dug across the diameter of earth. A ball is released from the surface of Earth into the tunnel. The velocity of ball when it is at a distance R2 from centre of earth is (where R = radius of Earth and M = mass of Earth)
A√3GM4RB√2GM3RC√GM2RD√2GMR
A tunnel is dug along a diameter of the earth. Find the force on a particle of mass m placed in the tunnel at a distance x from the centre.
View SolutionA tunnel is dug inside the earth across one of its diameters. Radius of earth is R and its mass is M. A particle is projected inside the tunnel with velocity √2GMR from one of its ends then maximum velocity attained by the particle in the subsequent motion is (assuming tunnel to be frictionless)
View SolutionA tuning is dug along the diameter of the earth. There is particle of mass m at the centre of the tunel. Find the minimum velocity given to the particle so that is just reaches to the surface of the earth. (R= radius of earth)
View SolutionTwo bodies of masses m and 2m are kept at distance r apart from each other. Then the value of G varies as
View SolutionA ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
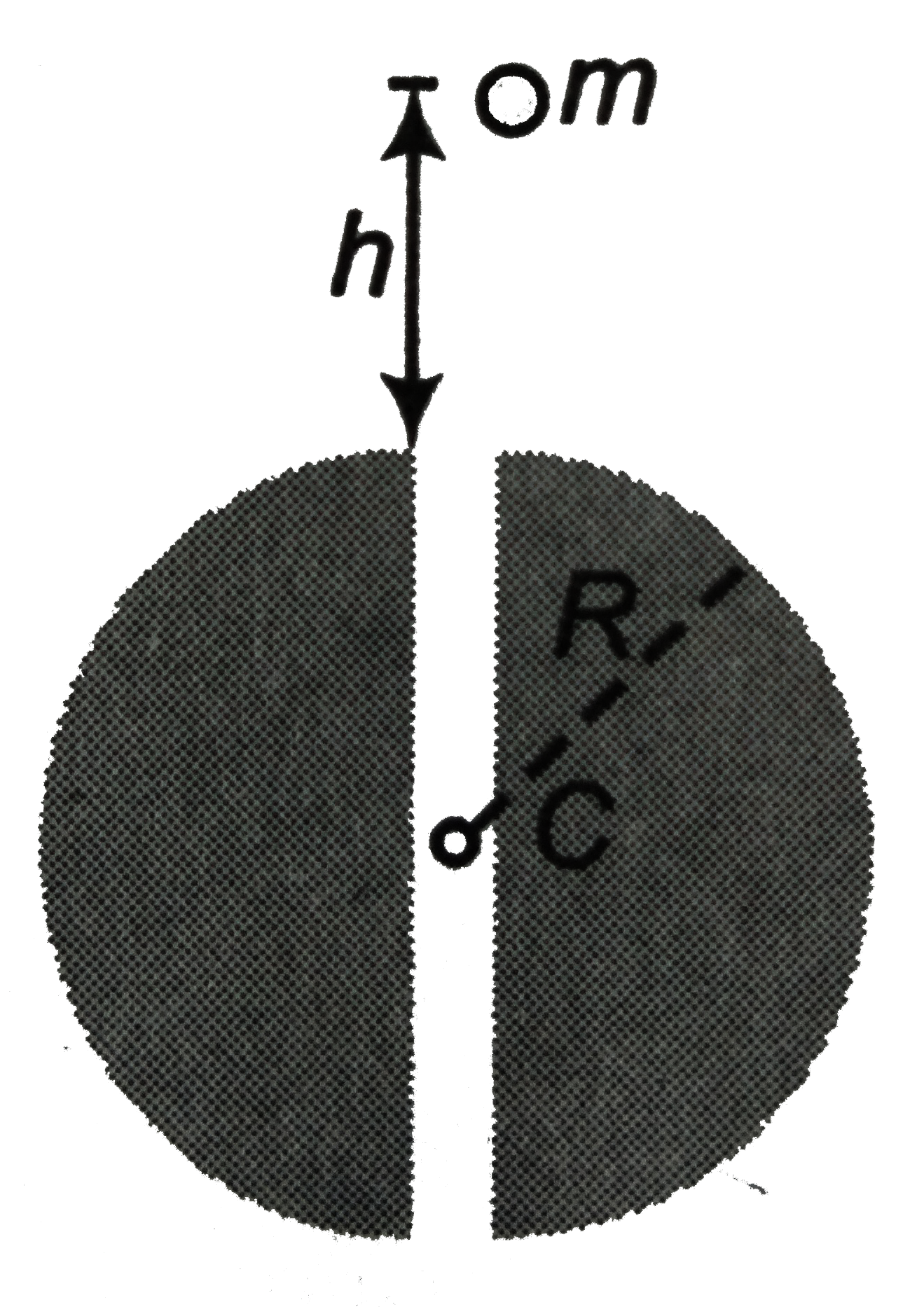
View Solution
