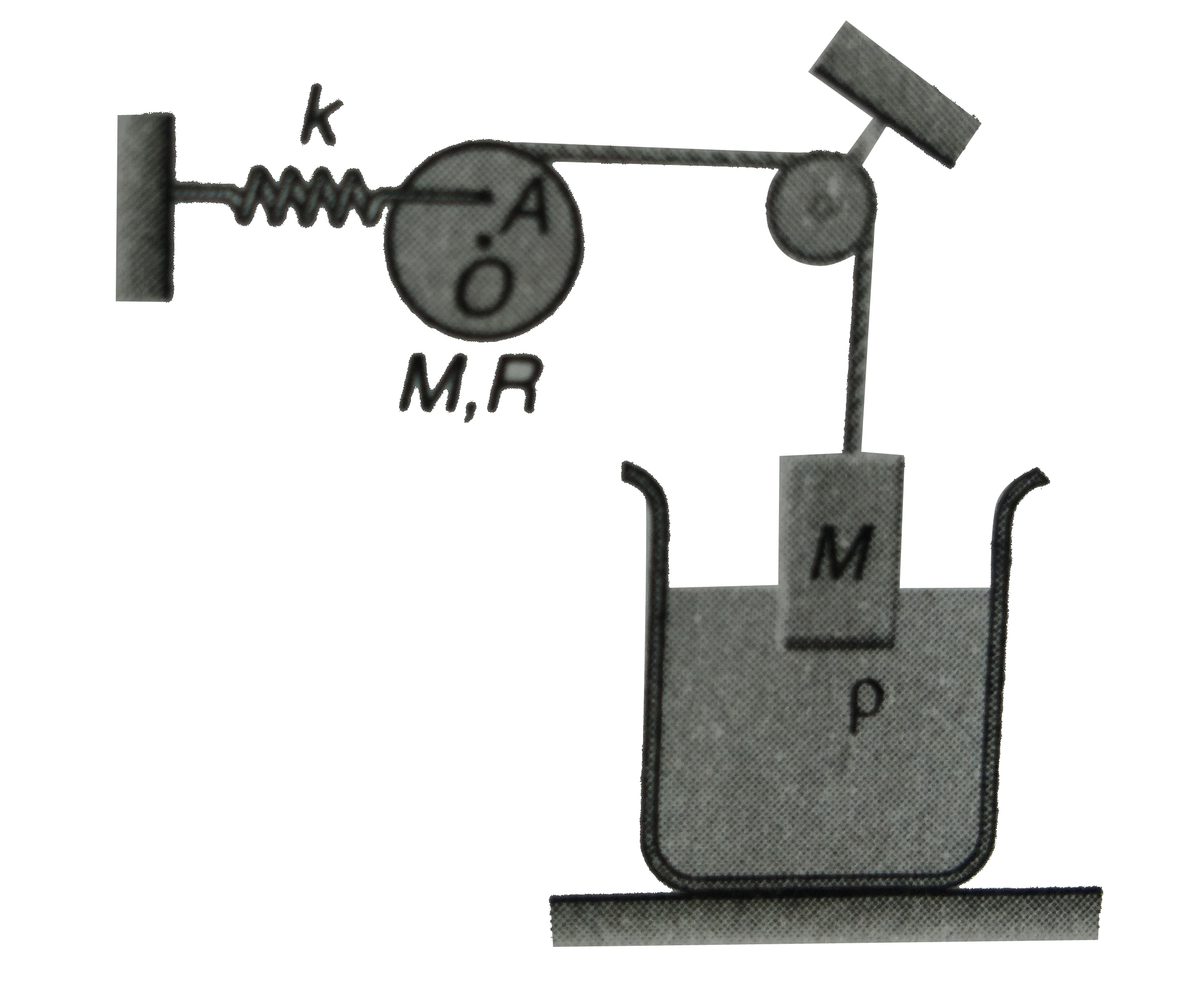
Figure shows a solid uniform cylinder of radius and mass , which is free to rotate about a fixed horizontal axis and passes through centre of the cylinder. One end of an ideal spring of force constant is fixed and the other end is higed to the cylinder at . Distance is equal to . An inextensible thread is wrapped round the cylinder and passes over a smooth, small pulley. A block of equal mass and having cross sectional area is suspended from free end of the thread. The block is partially immersed in a non-viscous liquid of density .
If in equilibrium, spring is horizontal and line is vertical, calculate frequency of small oscillations of the system.
The correct Answer is:A, B, D
In equilibrium,
…(i)
When the block is further depressed by , weight remains unchanged, upthrust increases by and let be the increase in tension.

If is the acceleration of block then,
...(ii)
Restoring torque on the cylinder,
or
or
Here negative sign has been used for restoting nature of torque
.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.15videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Intro. Exer.1videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension2videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
A cylinder of radius R full of liquid of density p is rotated about its axis at w rad/s. The increase in pressure at the centre of the cylinder will be
View SolutionA uniform cylinder of radius r and mass m can rotate freely about a fixed horizontal axis. A thin cord of length l and mass m0 is would on the cylinder in a single layer. Find the angular acceleration of the cylinder as a function of the length x of the hanging part of the end. the wound part of the cord is supposed to have its centre of gravity on the cylinder axis is shown in figure.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A solid cylinder of mass 50kg and radius 0.5m is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other end hanging freely. Tension in the string required to produce an angular acceleration of 2 revolution s−2 is
A25NB50NC78.5ND157N - Question 2 - Select One
A cylinder of mass 50mg and radius 0.5m is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other hanging freely. Tension in the string required to produce an angular acceleration of 2 revolutions s−2 is
A25 NB50 NC78.5 ND157 N - Question 3 - Select One
A solid cylinder of mass 50 kg and radius 0.5 m is free to rotate about the horizontal axis . A massless string is wound around the cylinder with one end attached to it and other hanging freely . Tension in the string required to produce an angular acceleration of 2 revolutions s−2 is
A25 NB50 NC78.5 ND157 N
A uniform cylinder of mass m rests on two rough horizontal planks. A thread is wound on the cylinder. The hanging end of the thread is pulled vertically down with a constant force F
View SolutionA solid cylinder has mass M radius R and length / its moment of inertia about an axis passing through its centre and perpendicular to its own axis is
View SolutionA solid cylinder has mass M radius R and length / its moment of inertia about an axis passing through its centre and perpendicular to its own axis is
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder isView Solution
A uniform solid cylinder having mass M and radius R is pulled by a horiozntal force F acting through the center as shown. The cylinder rolls to the right without slipping. What is the magnitude of the force of friction between the cylinder and the ground?View Solution

